Doubts and Solutions
OR
CBSE XI Science - Maths
Asked by kaivalyaam | 08 May, 2024, 12:25: AM
CBSE VIII - Science
Asked by heenaanwar141 | 07 May, 2024, 08:01: PM
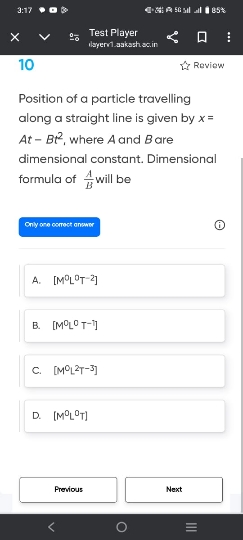
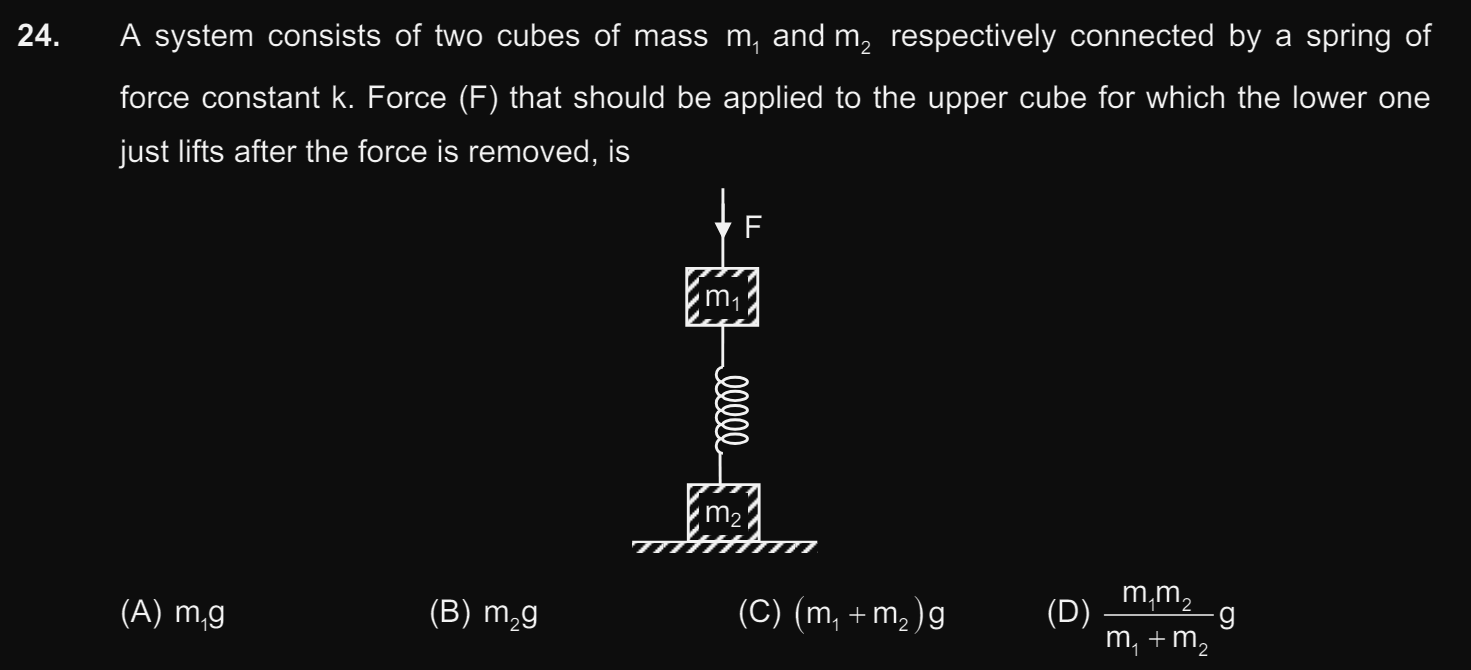
JEE Main - Physics
Asked by ashwinskrishna2006 | 07 May, 2024, 05:33: PM
CBSE VIII - Science
Asked by sppatil3668 | 07 May, 2024, 05:29: PM
CBSE XII Commerce - Accountancy
Asked by riyanprajapati308 | 07 May, 2024, 05:01: PM
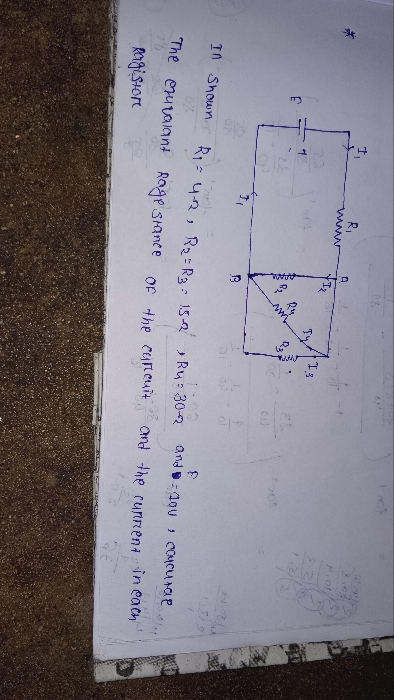
CBSE XI Science - Physics
Asked by rajeshabirami27 | 07 May, 2024, 04:31: PM