The upper half of an inclined plane with an inclination a, is perfectly smooth, while the lower half is rough. A body starting from rest at the top will again come to rest at the bottom, if the coefficient of friction for the lower half is given by:
Asked by Arushi Juyal
12th November 2017,
5:00 PM
Answered by Expert
Answer:
Refer the diagram above. For upper half which is smooth, the acceleration is g×sin(a).
Since the object starts from rest the final velocity when it reaches the end of upper half
is √( g×l×sin(a) ).
For the lower part the acceleration is ( g×sin(a) - μ×g×cos(a) ) due to friction
Since the oblect comes to rest when it reaches the bottom of inclined plane
we use the relation " u2 + 2αs = 0 ", where α is acceleration.
Hence ( g×l×sin(a) ) = -2 ( g×sin(a) - μ×g×cos(a) ) (l/2)
Solving for μ we will get μ = 2 × tan(a)
Answered by Expert
13th November 2017,
3:27 PM
Rate this answer
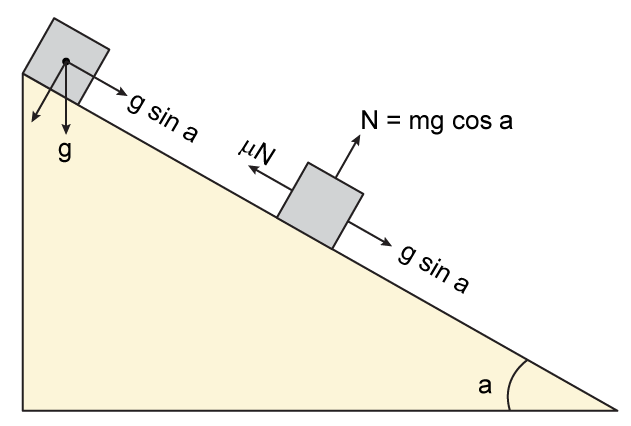
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
You have rated this answer 2/10
Your answer has been posted successfully!
RELATED STUDY RESOURCES :
NEET QUESTION ANSWERS
Trending Tags
Latest Questions
JEE Main Mathematics
Asked by amaladelsingh
28th October 2020,
10:24 PM
CBSE X Biology
Asked by reenacharan2008
28th October 2020,
9:11 PM
