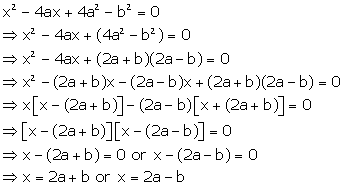Class 10 R S AGGARWAL AND V AGGARWAL Solutions Maths Chapter 4 - Quadratic Equations
Quadratic Equations Exercise Ex. 4A
Solution 1(i)
(i)![]() is a quadratic polynomial
is a quadratic polynomial
![]() = 0 is a quadratic equation
= 0 is a quadratic equation
Solution 1(ii)
![]()
Clearly ![]() is a quadratic polynomial
is a quadratic polynomial
![]() is a quadratic equation.
is a quadratic equation.
Solution 1(iii)
![]() is a quadratic polynomial
is a quadratic polynomial
![]()
![]() = 0 is a quadratic equation
= 0 is a quadratic equation
Solution 1(iv)

Solution 1(v)
![]() is not a quadratic polynomial since it contains
is not a quadratic polynomial since it contains![]() in which power
in which power ![]() of x is not an integer.
of x is not an integer.
![]() is not a quadratic equation
is not a quadratic equation
Solution 1(vi)
![]()
![]()
And ![]() Being a polynomial of degree 2, it is a quadratic polynomial.
Being a polynomial of degree 2, it is a quadratic polynomial.
Hence, ![]() is a quadratic equation.
is a quadratic equation.
Solution 1(vii)

Solution 1(viii)
![]()
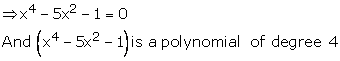
![]() is not a quadratic equation
is not a quadratic equation
Solution 1(ix)
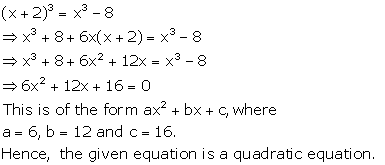
Solution 1(x)
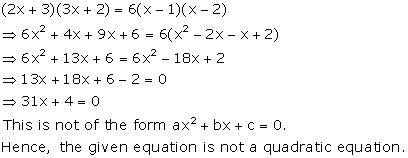
Solution 1(xi)
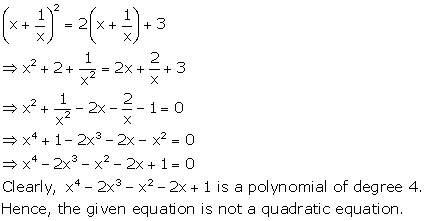
Solution 2
The given equation is ![]()
(i)On substituting x = -1 in the equation, we get
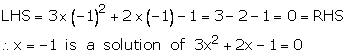
(ii)On substituting ![]() in the equation, we get
in the equation, we get
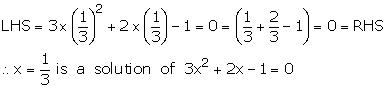
(iii)On substituting ![]() in the equation
in the equation ![]() , we get
, we get
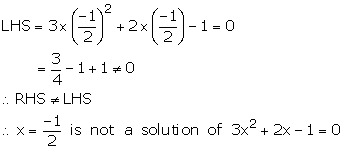
Solution 3(i)
Since x =1 is a root of the equation ![]() , it must satisfy
the equation.
, it must satisfy
the equation.

Substituting ![]() in
in ![]() ,
,

Hence k=-4 and other root is 3.
Solution 3(ii)
Since ![]() is a root of
is a root of ![]() , we have
, we have![]()
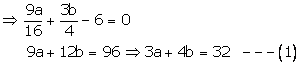
Again x = -2 being a root of ![]() , we have
, we have
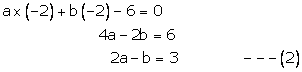
Multiplying (2) by 4 adding the result from (1), we get
11a = 44 ![]() a = 4
a = 4
Putting a = 4 in (1), we get
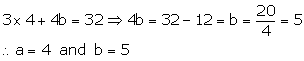
Solution 4
Given equation is ![]() .
.
Substituting ![]() in LHS of the given equation,
in LHS of the given equation,
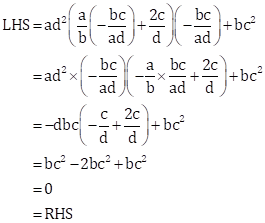
![]() is a solution of the quadratic equation
is a solution of the quadratic equation ![]() .
.
Solution 5

Solution 6
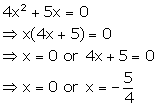
Solution 7
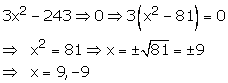
Hence, 9 and -9 are the roots of the equation ![]()
Solution 8
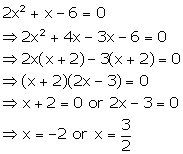
Solution 9
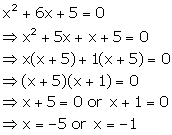
Solution 10

Solution 11

Solution 12
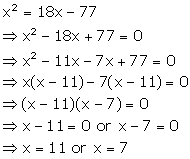
Solution 13
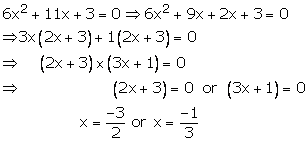
Hence, ![]() are the roots of
are the roots of ![]()
Solution 14
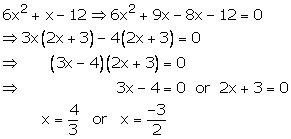
Hence, ![]() are the roots of equation
are the roots of equation![]()
Solution 15
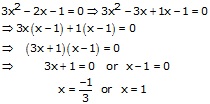
Hence, ![]() and 1 are the roots of the equation
and 1 are the roots of the equation ![]() .
.
Solution 16
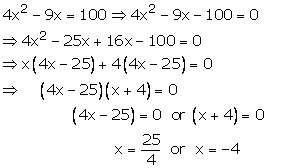
![]() are the roots of the equation
are the roots of the equation ![]()
Solution 17

Hence, ![]() are the roots of the given equation
are the roots of the given equation ![]()
Solution 18
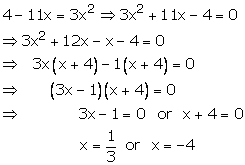
Hence, ![]() are the roots of given equation
are the roots of given equation
Solution 19
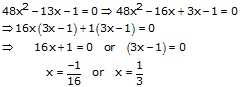
Hence, ![]() are the roots of
are the roots of ![]()
Solution 20

Solution 21
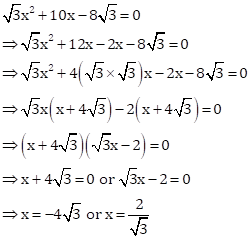
Solution 22

![]()

Solution 23

Hence, ![]() are the roots of the given equation
are the roots of the given equation
Solution 24

Solution 25
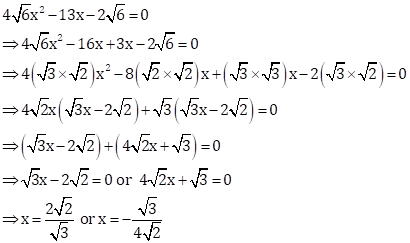
Solution 26

Solution 27

Solution 28

Solution 29

Solution 30

Solution 31
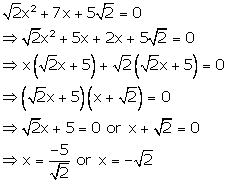
Solution 32
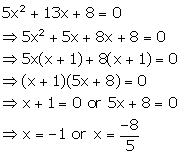
Solution 33
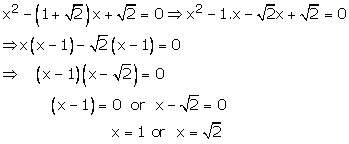
Hence, 1 and ![]() are the roots of the given equation
are the roots of the given equation
Solution 34

Solution 35
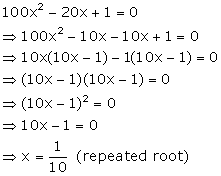
Solution 36
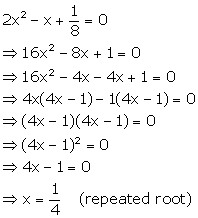
Solution 37
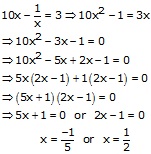
Hence, ![]() are the roots of the given equation
are the roots of the given equation
Solution 38
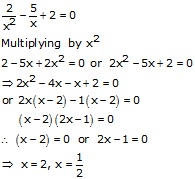
Hence, 2 and ![]() are the roots of given equation
are the roots of given equation
Solution 39
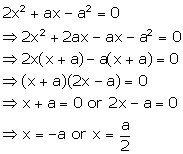
Solution 40
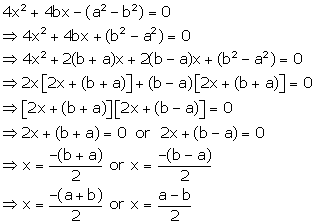
Solution 41
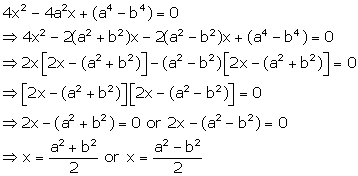
Solution 42
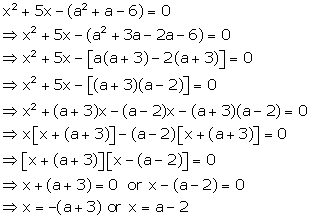
Solution 43
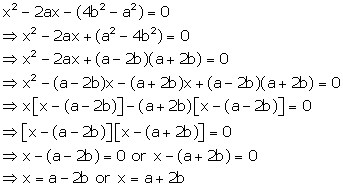
Solution 44
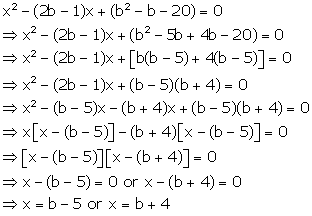
Solution 45
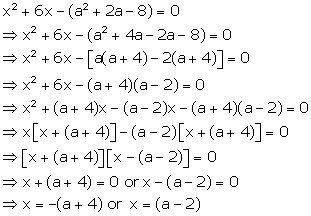
Solution 46

Hence, ![]() are the roots of the given equation
are the roots of the given equation
Solution 47
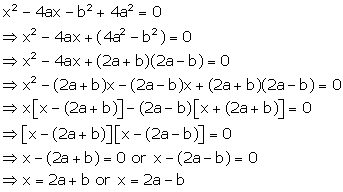
Solution 48

Hence, ![]() are the roots of given equation
are the roots of given equation
Solution 49

Hence, ![]() are the roots of given equation
are the roots of given equation
Solution 50

Hence, ![]() are the roots of given equation
are the roots of given equation
Solution 51
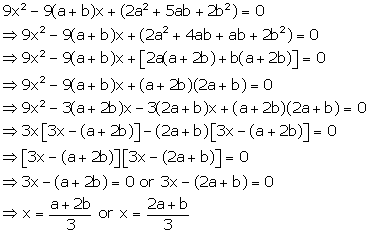
Solution 52

Solution 53
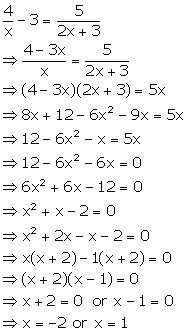
Solution 54
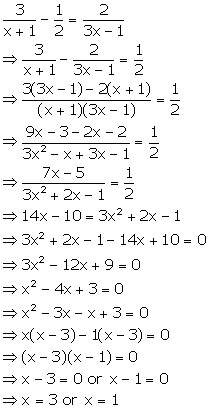
Solution 55(i)
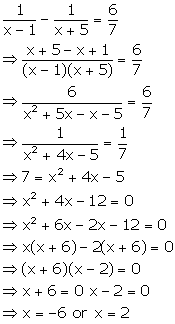
Solution 55(ii)
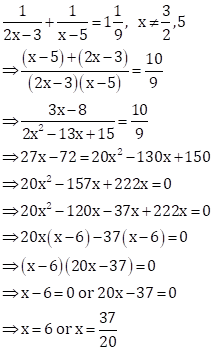
Solution 56
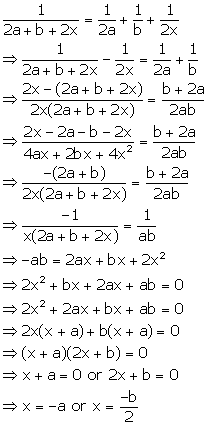
Solution 57
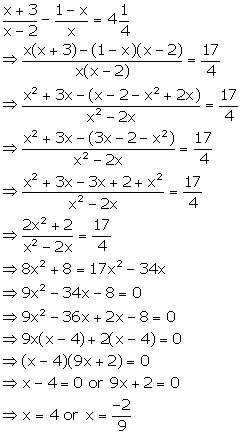
Solution 58
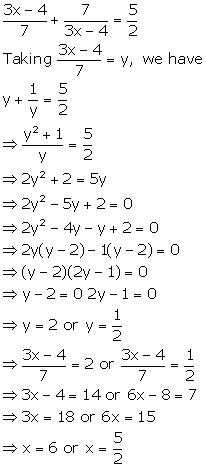
Solution 59(i)
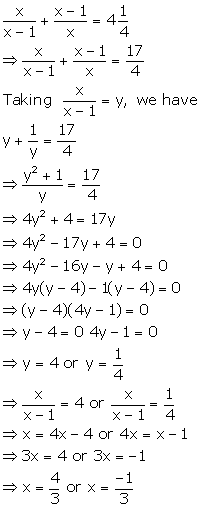
Solution 59(ii)
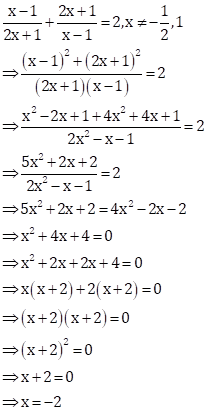
Solution 60

Solution 61
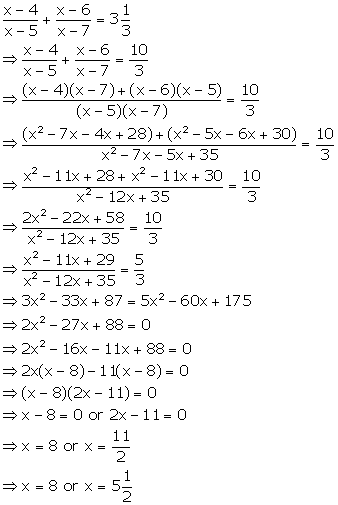
Solution 62
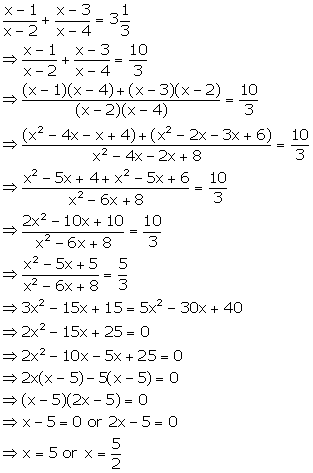
Solution 63

Solution 64(i)
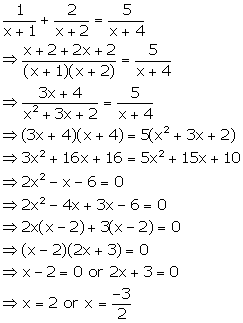
Solution 64(ii)
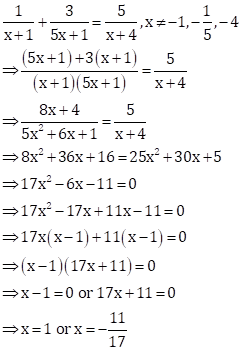
Solution 65

Solution 66
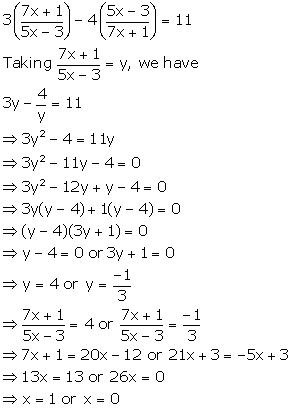
Solution 67
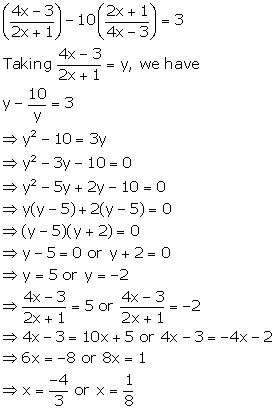
Solution 68
Putting ![]() the given equation become
the given equation become
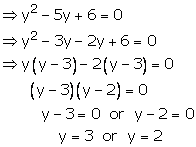
Case I:

Case II:
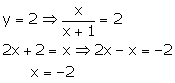
Hence, ![]() are the roots of the given equation
are the roots of the given equation
Solution 69
The given equation

Hence, ![]() is the roots of the given equation
is the roots of the given equation
Solution 70

Solution 71
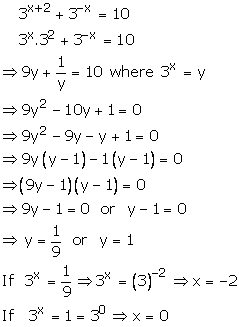
Hence, -2,0 are the roots of given equation
Solution 72

Hence, ![]() are the roots of given equation
are the roots of given equation
Solution 73

Hence, 3 and 2 are roots of the given equation
Quadratic Equations Exercise Ex. 4B
Solution 1(i)
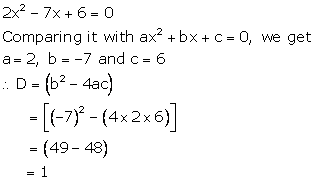
Solution 1(ii)
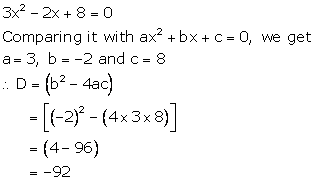
Solution 1(iii)
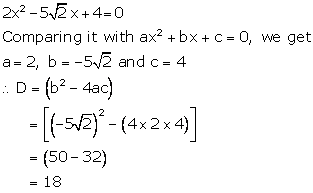
Solution 1(iv)

Solution 1(v)

Solution 1(vi)
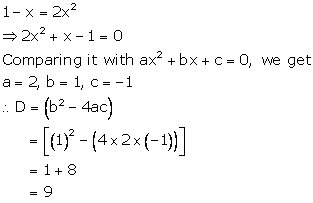
Solution 2

Solution 3

Solution 4
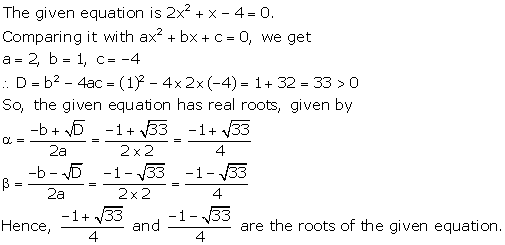
Solution 5

Solution 6
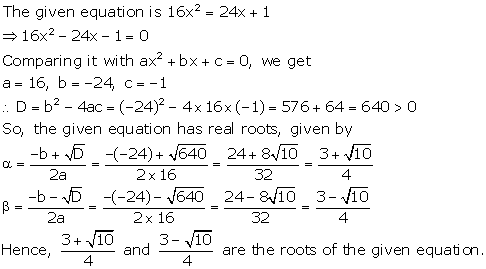
Solution 7
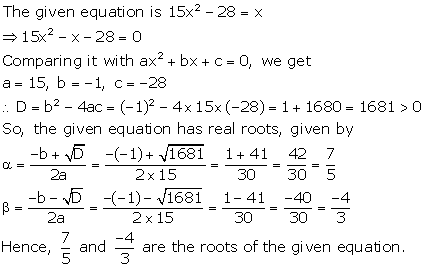
Solution 8

Solution 9

Solution 10

Solution 11

Solution 12

Solution 13

Solution 14

Solution 15
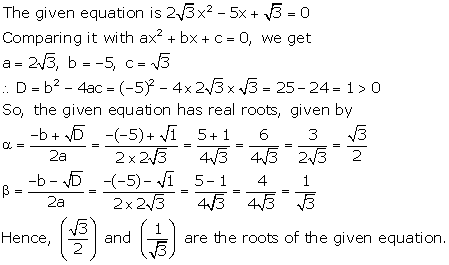
Solution 16
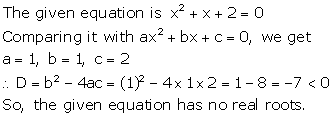
Solution 17
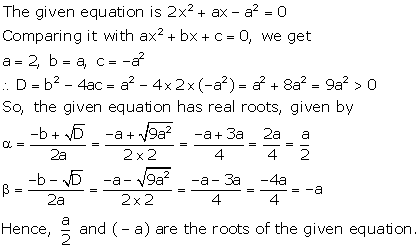
Solution 18

Solution 19

Solution 20
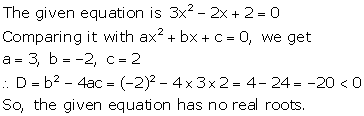
Solution 21
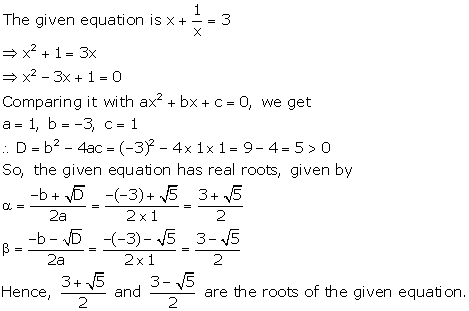
Solution 22
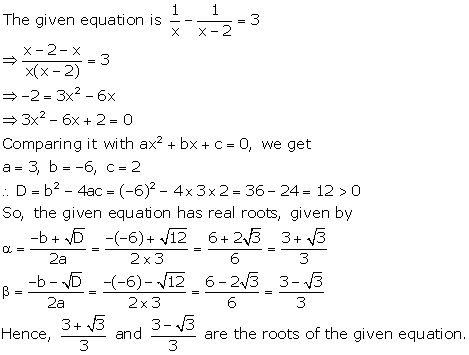
Solution 23
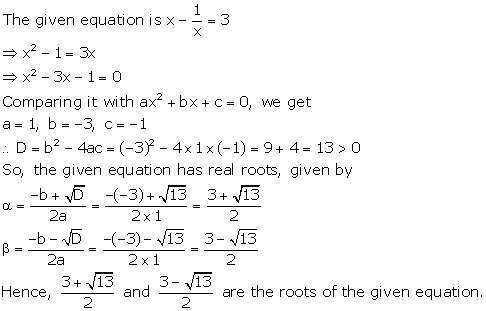
Solution 24

Solution 25

Solution 26

Solution 27

Solution 28

Solution 29

Solution 30
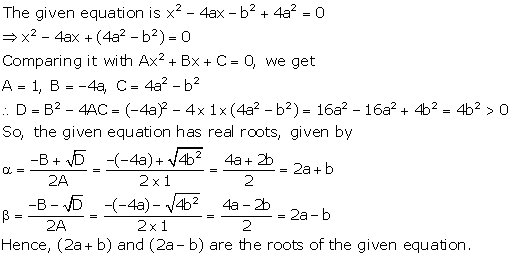
Solution 31
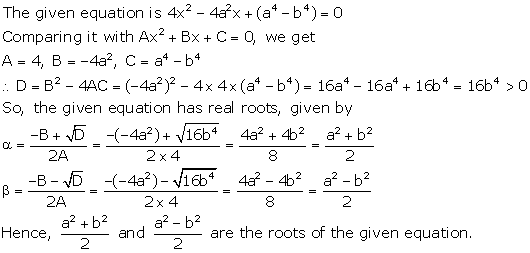
Solution 32

Solution 33
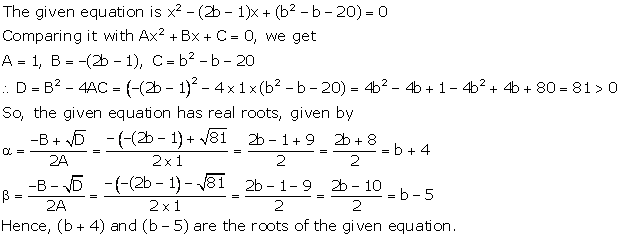
Solution 34
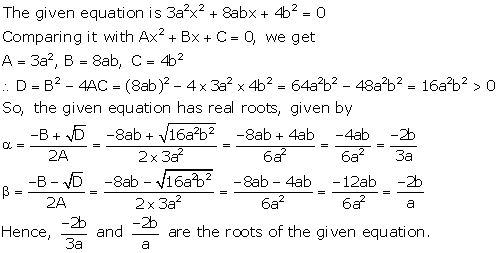
Solution 35
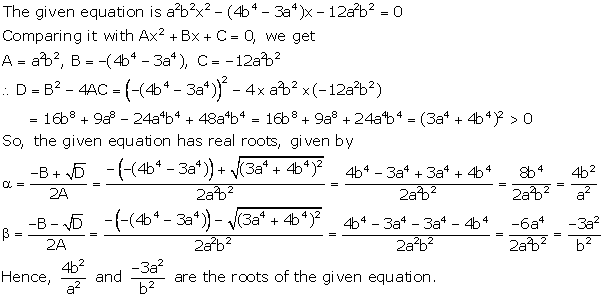
Solution 36
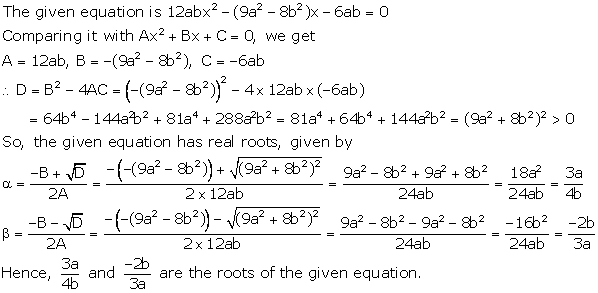
Quadratic Equations Exercise Ex. 4C
Solution 1(i)
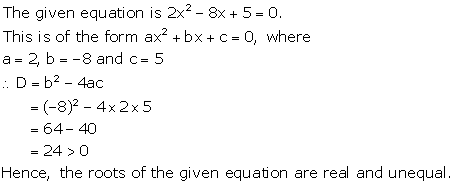
Solution 1(ii)
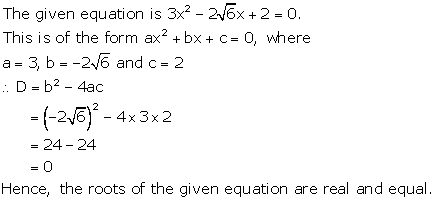
Solution 1(iii)
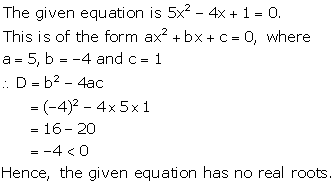
Solution 1(iv)
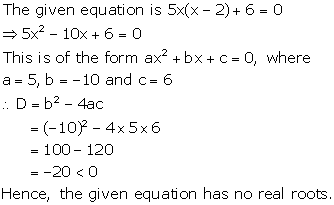
Solution 1(v)
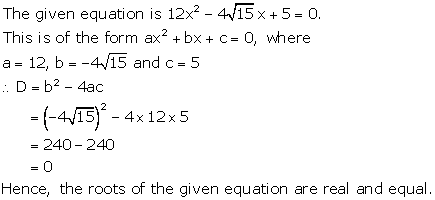
Solution 1(vi)
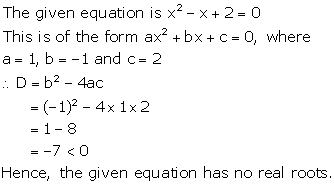
Solution 2
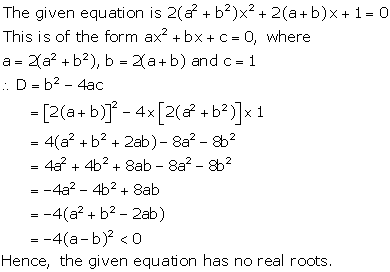
Solution 3
The given equation is ![]()
This is the form of ![]()

Now ![]() .
.
So, the roots of the given equation are real for all real values of p and q.
Solution 4
Solution 5

Solution 6
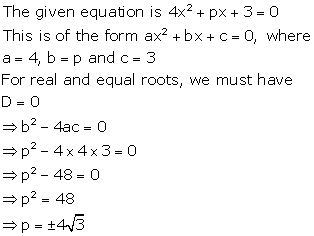
Solution 7
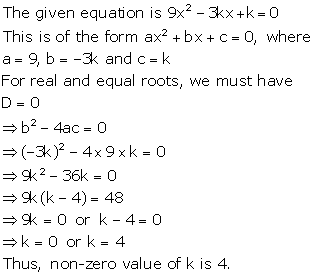
Solution 8(i)
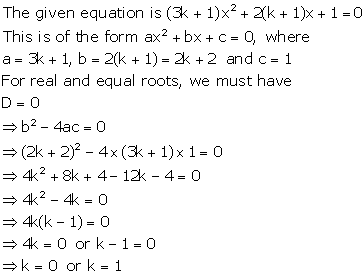
Solution 8(ii)
The given equation is
![]()
![]()
This is of the form
![]() , where
, where
![]()
For real and equal roots, we must have
Discriminant, D = 0
![]()
![]()
![]()
![]()
![]()
![]()
Solution 9
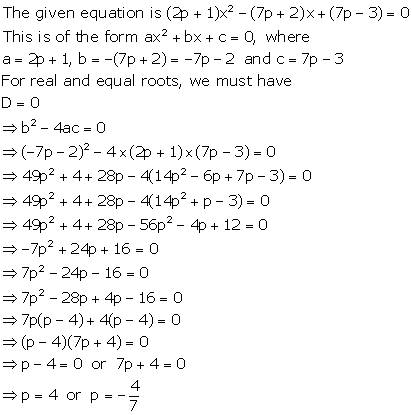
Solution 10

Solution 11
Since -5 is a root
of the quadratic equation ![]() , it must satisfy
the equation.
, it must satisfy
the equation.

The other quadratic
equation is ![]()
![]() (i)
(i)
Substituting p = 7 in (i),
![]()
This is of the form
![]() , where
, where
![]()
For equal roots, we must have
Discriminant, D = 0
![]()
![]()
![]()
![]()
![]()
Solution 12

Solution 13
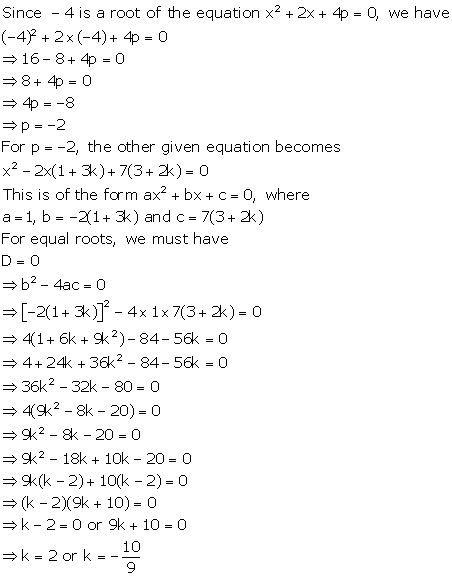
Solution 14
The given equation is ![]()


For real and equal roots, we must have D = 0

Solution 15
The given equation is ![]()
![]()
![]()
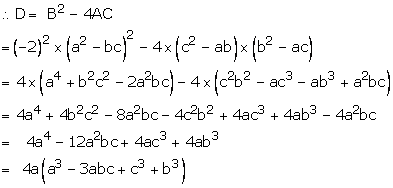
For real and equal roots , we must have D =0

Solution 16
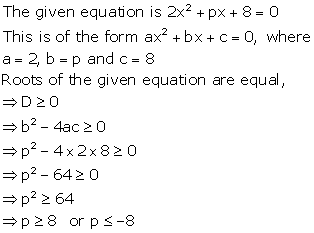
Solution 17

Solution 18
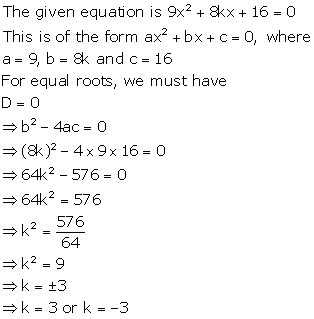
Solution 19(i)
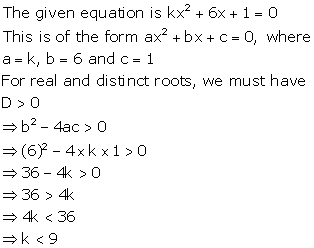
Solution 19(ii)
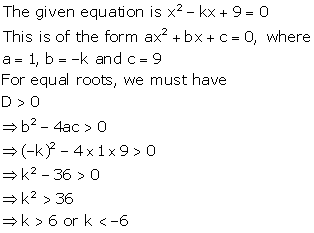
Solution 19(iii)
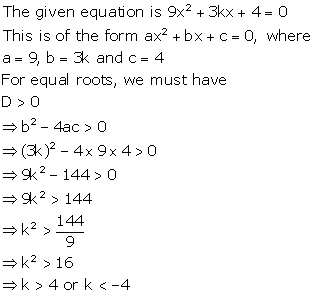
Solution 19(iv)
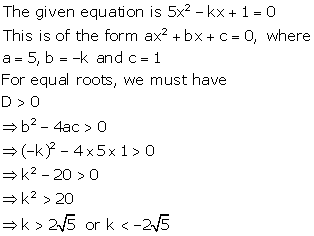
Solution 20

Solution 21
The given equation is ![]()
This is of the form ![]() , where
, where
![]()
For equal roots, Discriminant, D = 0
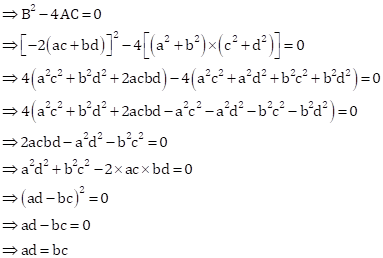
Solution 22
The given equation
is ![]()
This is of the form
![]() , where
, where
![]()
Now, Discriminant
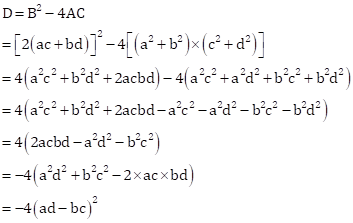
Now,
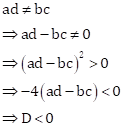
Hence, the given equation has no real roots.
Solution 23

Solution 24
Since ![]() is a root of the
quadratic equation
is a root of the
quadratic equation ![]() , it must satisfy
the equation.
, it must satisfy
the equation.
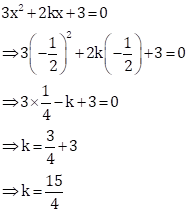
Solution 25
Since ![]() is a root of the quadratic equation
is a root of the quadratic equation ![]() , it must satisfy
the equation.
, it must satisfy
the equation.
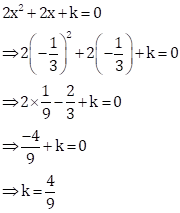
Solution 26
Given quadratic
equation is ![]() .
.

But, square of a number cannot be negative.
Hence, the given equation has no solution.
Solution 27
The given equation
is ![]()
This is of the form
![]() , where
, where
![]()
For no real roots,
Discriminant, D <0
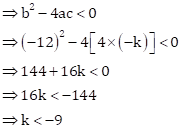
Solution 28
The given equation is ![]()
This is of the form ![]() , where
, where
![]()
Let the roots of the equation be ![]()
Then,
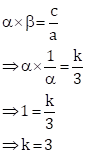
Solution 30
The given equation
is ![]()
This is of the form
![]() , where
, where
![]()
For real and equal roots, we must have
Discriminant, D = 0
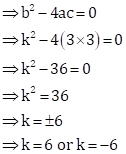
Solution 31
The given equation
is ![]()
This is of the form
![]() , where
, where
![]()
For real and equal roots, we must have
Discriminant, D = 0

Solution 32
The given equation
is ![]()
![]()
This is of the form
![]() , where
, where
![]()
For real and equal roots, we must have
Discriminant, D = 0
![]()
![]()
![]()
![]()
![]()
![]()
Solution 29
The given equation is ![]()
This is of the form ![]() , where
, where
![]()
Let the roots of the equation be ![]()
Then,
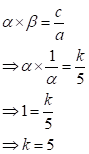
Quadratic Equations Exercise Ex. 4D
Solution 1

Solution 2

Solution 3

Solution 4

Solution 5

Solution 6

Solution 7

Solution 8
Let the required natural number be x and x - 3.
Then,

But, x is a natural number.

Hence, 
Therefore, the required numbers are 24 and 21.
Solution 9

Solution 10

Solution 11

Solution 12

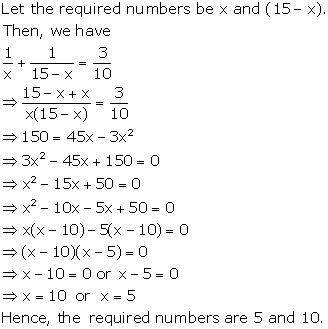
Solution 13
Solution 14
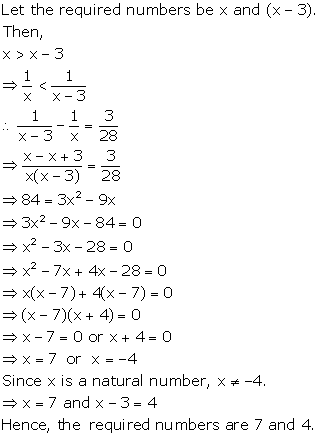
Solution 15

Solution 16

Solution 17
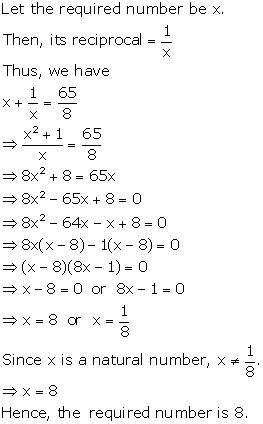
Solution 18

Solution 19
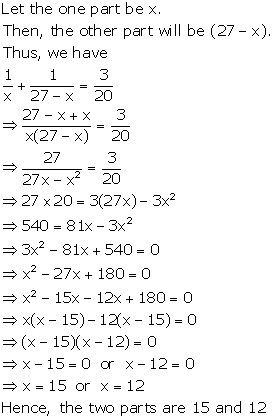
Solution 20
Let the smaller part and larger part be x, 16 - x
Then,

-42 is not a positive part.
Hence, the larger part is 10 and the smaller part is 6.
Solution 21
Let the required natural number be x and y.
Then

Therefore, the required natural numbers are 30 and 10 respectively.
Solution 22
Let x, y be the two natural numbers and x > y
![]() ------(1)
------(1)
Also, square of smaller number = 4 larger number
![]() ---------(2)
---------(2)
Putting value of ![]() from (1), we get
from (1), we get
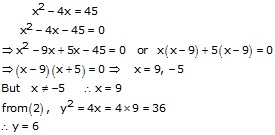
Thus, the two required numbers are 9 and 6.
Solution 23
Let the three consecutive numbers be x, x + 1, x + 2
Sum of square of first and product of the other two
![]()
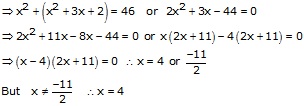
![]() Required numbers are 4, 5 and 6.
Required numbers are 4, 5 and 6.
Solution 24
Let the tens digit be x and units digit be y


Hence, the tens digit is 3 and units digit is 6.
Hence, the required number is 36.
Solution 25
Let the tens and units digits of the required number be x and y respectively.
Then,

Now, the number is  .
.
So, 

But, digit cannot be negative.

Hence, the required number is 27.
Solution 26
Let the numerator and denominator be x, x + 3
Then,

Hence, numerator and denominator are 2 and 5 respectively and fraction is ![]()
Solution 27

Solution 28
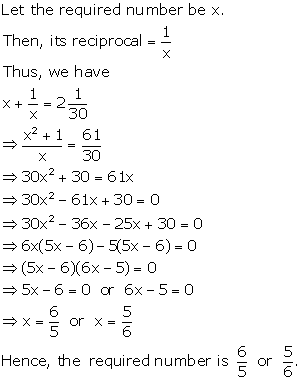
Solution 29
Let there be x rows and number of students in each row be x.
Then, total number of students = ![]()

Hence, total number of students
![]()
Therefore, total number of students is 600.
Solution 30
Let the number of students be x.
Then,

![]()
Hence, the number of students is 50.
Solution 31
Let the marks obtained by Kamal in Mathematics and English be x and y respectively.
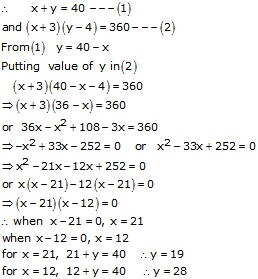
Therefore, the marks obtained by Kamal in Mathematics and English respectively are (21,19) or (12,28).
Solution 32

Solution 33
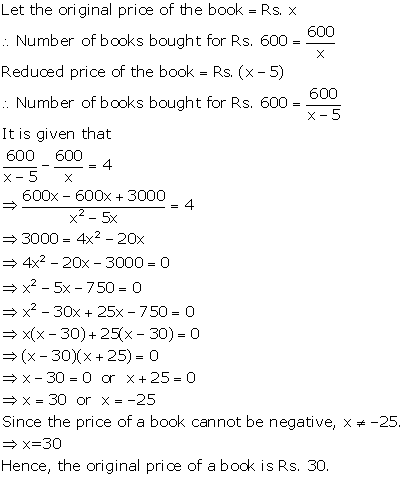
Solution 34

Solution 35
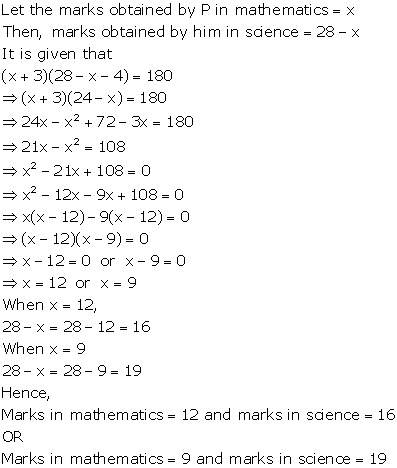
Solution 36

Solution 37
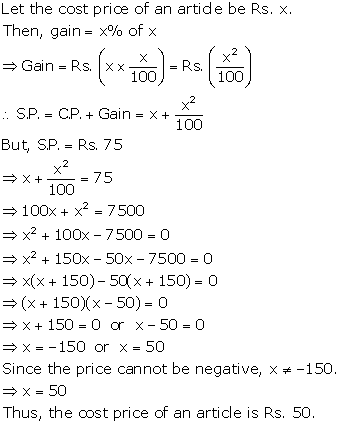
Solution 38(i)
Let the age of son be x years and that of man be y years.
1 year ago,
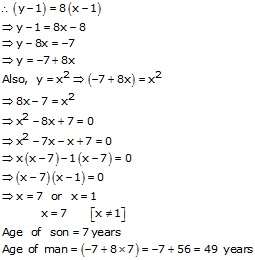
Solution 38(ii)
Let the ages of son and father be x years and y years respectively.
Then, ![]()
Now, sum of the squares of their ages = 1325
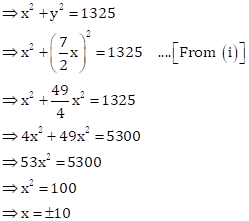
But, age cannot be negative.
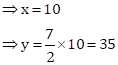
Therefore, the age of father is 35 years and that of son is 10 years.
Solution 39

Solution 40

Solution 41
Let the present age of Tanvi be x years.
Then,
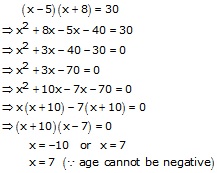
Hence, the present age of Tanvi is 7 years.
Solution 42
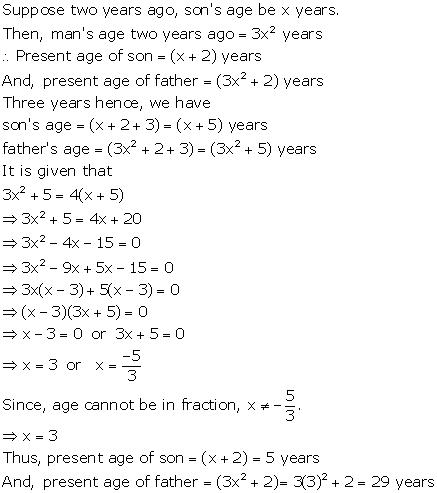
Solution 43

Solution 44
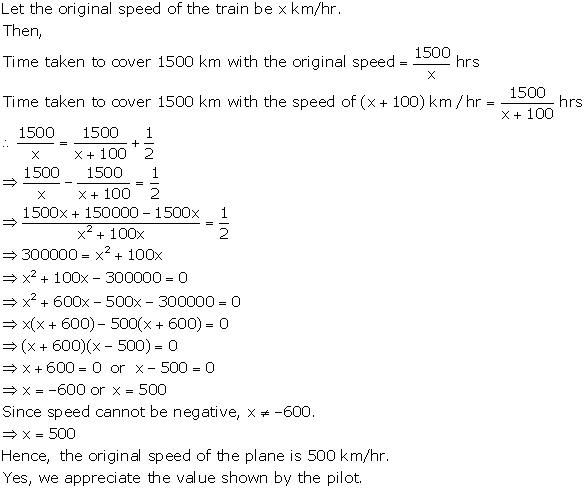
Solution 45

Solution 46
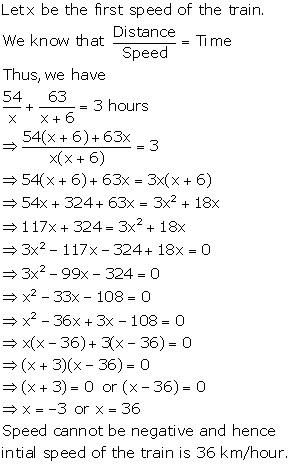
Solution 47

Solution 48
Let the
original speed of the train be ![]() km/hr.
km/hr.
Then, increased speed = (x+5)km/hr
Time taken at
original speed = ![]() hours
hours
Time taken at
increased speed = ![]() hours
hours
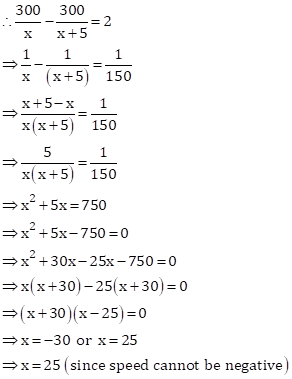
Hence, the original speed of the train is 25 km/hr.
Solution 49

Solution 50
Let the speed of the Deccan Queen = x kmph
The, speed of other train = (x - 20)kmph
Then, time taken by Deccan Queen = ![]()
Time taken by other train = ![]()
Difference of time taken by two trains is ![]()
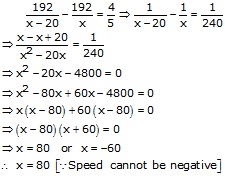
Hence, speed of Deccan Queen = 80km/h
Solution 51
Let the speed of stream be x km/h
Speed of boat in still stream = 18 km/h
Speed of boat up the stream = 18 - x km/h
![]() Time taken by boat to go up the stream 24 km =
Time taken by boat to go up the stream 24 km = ![]()
![]() Time taken by boat to go down the stream =
Time taken by boat to go down the stream = ![]()
Time taken by the boat to go up the stream is 1 hour more that the time taken down the stream
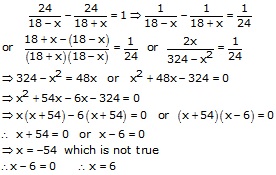
![]() Speed of the stream = 6 km/h
Speed of the stream = 6 km/h
Solution 52
Let the speed of the stream be = x km/h
Speed of boat in still water = 15 km/hr
Speed of boat upstream = (15-x) km/hr
∴ Time taken by the boat to go 30 km upstream ![]() hours
hours
Speed of boat downstream
= ![]() km/hr
km/hr
∴ Time taken by the boat to go 30 km downstream ![]()
Total time taken by the boat ![]()

Hence, the speed of the stream is 5 km/hr.
Solution 53
Let the speed of the stream be = x km/h
Speed of boat in still waters = 9 km/h
Speed of boat down stream = 9 + x
![]() time taken by boat to go 15 km downstream =
time taken by boat to go 15 km downstream = ![]()
Speed of boat upstream = 9 - x
![]() time taken by boat to go 15 km of stream =
time taken by boat to go 15 km of stream = ![]()
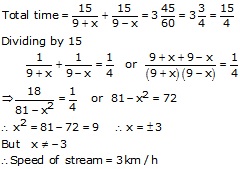
Solution 54
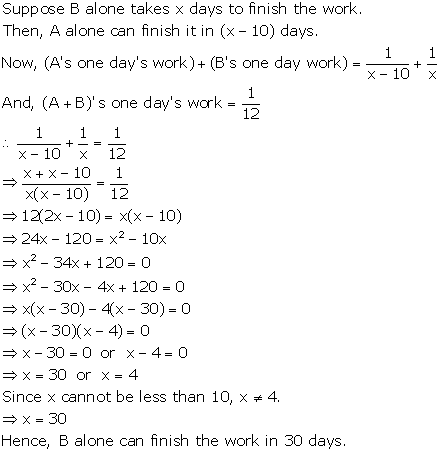
Solution 55
Let one tap takes x minutes to fill the tank.
Then, the other tap will take (x + 3) hours to fill tank.
Total time taken to fill the tank by two taps = 
Part filled by one tap in 1 hour 
Part filled by other tap in 1 hour 
Part filled by both the taps in 1 hour 

Therefore, one tap takes 5 hours to fill the tank and other tap takes (5 + 3) 8 hours to fill the tank.
Solution 56

Solution 57

Solution 58
Let the breadth of a rectangle = x cm
Then, length of the rectangle = 2x cm
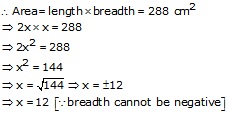
Thus, breadth of rectangle = 12 cm
And length of rectangle = (2 x 12) = 24 cm
Solution 59
Let the breadth of a rectangle = x meter
Then, length of rectangle = 3x meter
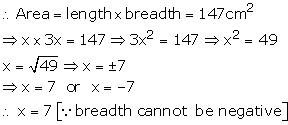
Thus, breadth of rectangle = 7 m
And length of rectangle = (3 x 7)m = 21 m
Solution 60
Let the breadth of hall = x meters
Then, length of the hall = (x + 3) meters
![]() Area = length breadth =
Area = length breadth = ![]()

Thus, the breadth of hall is 14 m
And length of the hall is (14 + 3) = 17 m
Solution 61
We know that,
Perimeter of a rectangle = 2(length + breadth)
⇒ 60 = 2(length + breadth)
⇒ length + breadth = 30 m
Let the length of a rectangular plot be x m.
Then, breadth of a rectangular plot = (30-x) m
Now,
Area of a rectangular plot = 200 sq. m
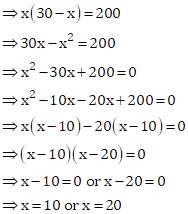
Hence, the length and breadth of a rectangular plot are 20 m and 10 m respectively.
Solution 62
Let the width of the path be x m.
Length of the field
including the path = ![]() m
m
Breadth of the field including
the path = ![]() m
m
Area of the field including the
path = ![]()
Area of the field excluding the
path = ![]()
Therefore, area of the path ![]()

Hence, the width of the path is 2 m.
Solution 63
Let x and y be the lengths of the two square fields.
![]()
4x - 4y = 64
![]() x - y = 16------(2)
x - y = 16------(2)
From (2),
x = y + 16,
Putting value of x in (1)
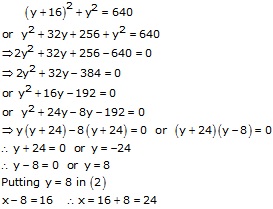
![]() Sides of two squares are 24 m and 8 m respectively.
Sides of two squares are 24 m and 8 m respectively.
Solution 64
Let the side of square be x cm
Then, length of the rectangle = 3x cm
Breadth of the rectangle = (x - 4) cm
![]() Area of rectangle = Area of square x
Area of rectangle = Area of square x
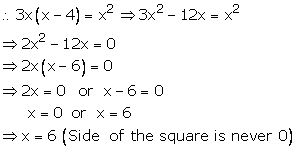
Thus, side of the square = 6 cm
And length of the rectangle = (3 6) = 18 cm
Then, breadth of the rectangle = (6 - 4) cm = 2 cm
Solution 65
Let the length = x meter
Area = length breadth = ![]()

If ength of the rectangle = 15 m
![]()
Also, if length of rectangle = 24 m
![]()
Solution 66
Let the altitude of triangle be x cm.
Then, base of triangle is (x + 10) cm.

Hence, altitude of triangle is 30 cm and base of triangle 40 cm.
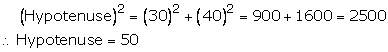
Therefore, the dimensions of the triangle are 30 cm, 40 cm and 50 cm.
Solution 67
Let the altitude of triangle be x meter.
Hence, base = 3x meter

Hence, altitude of triangle is 8 m.
And base of triangle = 3x = (3 x 8) cm = 24 m
Solution 68
Let the base of triangle be x meter
Then, altitude of triangle = (x + 7) meter

Thus, the base of the triangle = 15 m
And the altitude of triangle = (15 + 7) = 22 m
Solution 69
Let the other sides of triangle be x and (x - 4) meters.
By Pythagoras theorem, we have

Thus, height of triangle = 16 m
And the base of the triangle = (16 - 4) = 12 m
Solution 70
Let the base of the triangle be x
Then, hypotenuse = (x + 2) cm

Thus, base of triangle = 15 cm
Then, hypotenuse of triangle = (15 +2 )= 17 cm
And altitude of triangle = ![]()
Solution 71
Let the shorter side of triangle be x meter
Then, its hypotenuse = (2x - 1)meter
And let the altitude = (x + 1) meter

Solution 72
Let the speed of the fast train be x kmph.
Then, the speed of the slow train = ![]() kmph
kmph
Time taken by fast train to cover 200 km ![]() hours
hours
Time taken by slow train to cover 200 km ![]() hours
hours
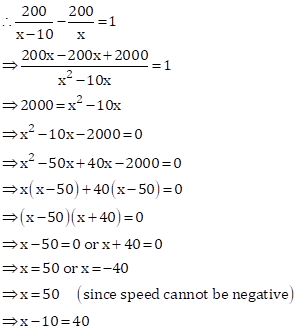
Hence, the speeds of two trains are 50 kmph and 40 kmph respectively.
Solution 73
Let the speed of the stream be = x kmph
Speed of boat in still water = 18 kmph
Speed of boat upstream = (18-x) km/hr
∴ Time
taken by the boat to go 36 km upstream ![]() hours
hours
Speed of boat downstream = (18+x) km/hr
∴ Time
taken by boat to go 36 km downstream ![]()
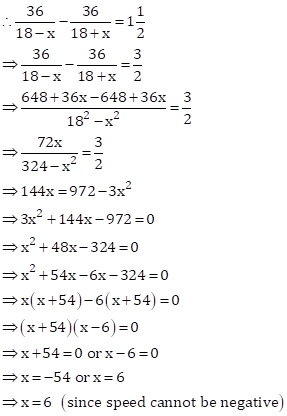
Hence, the speed of the stream is 6 kmph.
Solution 74
Let the time taken by the second pipe to fill the tank = x hours
Then, the time taken by the first pipe to fill the tank = (x-10) hours
Total time taken by both pipes to fill the tank = 12 hours
Part filled by second pipe in 1
hour = ![]()
Part filled by first pipe in 1
hour = ![]()
Part filled by both pipes
together in 1 hour = ![]()
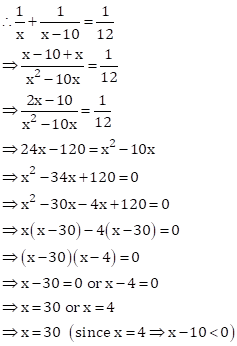
Hence, the time taken by second pipe to fill the tank is 30 hours.
Solution 75
Let the time taken by smaller tap to fill the tank = x hours
Then, the time taken by larger tap to fill the tank = (x-2) hours
Total time taken by both pipes to
fill the tank = ![]()
Part filled by smaller tap in 1
hour = ![]()
Part filled by larger tap in 1
hour = ![]()
Part filled by both taps together
in 1 hour = ![]()
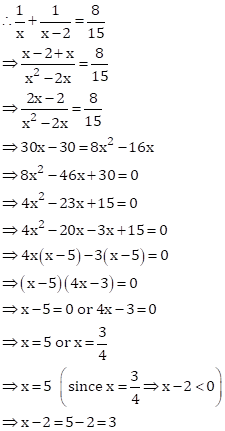
Hence, the smaller tap will take 3 hours and the larger tap will take 5 hours to fill the tank separately.
Solution 76
Let the original speed of the aircraft be x kmph.
Then, time taken to
cover 600 km = ![]() hours
hours
Reduced speed = (x-200) kmph
Time taken to cover
600 km at this speed = ![]() hours
hours
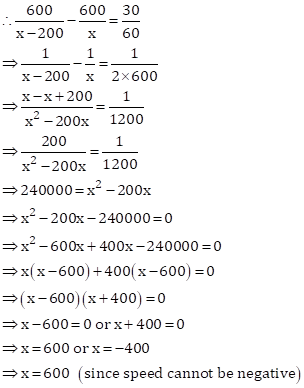
Therefore, original speed of the aircraft = 600 kmph
And, original
duration of the flight![]()
Solution 77
Let the
time taken by larger pipe and the smaller pipe to fill the pool be ![]() hours and
hours and ![]() hours respectively.
hours respectively.
Total time taken by both pipes to fill the tank = 12 hours
![]()
In 4 hours, part of
pool filled by the larger pipe ![]()
In 9 hours, part of
pool filled by the smaller pipe ![]()
![]()
Multiplying (i) by 4,
![]()
Subtracting (iii) from (ii),
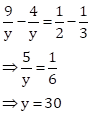
Substituting y=30 in (i),
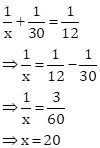
Hence, the larger pipe will take 20 hours and the smaller pipe will take 30 hours to fill the pool separately.
Quadratic Equations Exercise Test Yourself
Solution 1
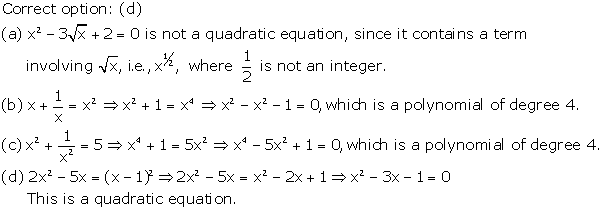
Solution 2
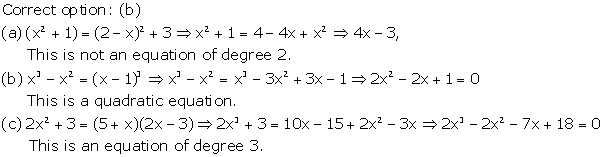
Solution 3
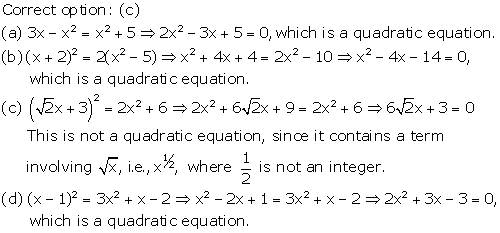
Solution 4
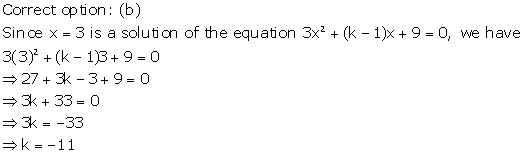
Solution 5

Solution 6
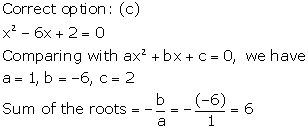
Solution 7

Solution 8
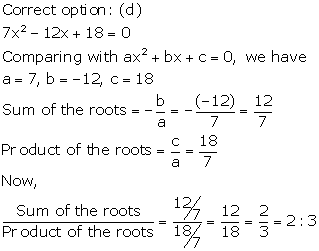
Solution 9

Solution 10

Solution 11

Solution 12

Solution 13
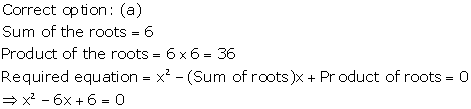
Solution 14
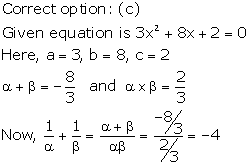
Solution 15

Solution 16
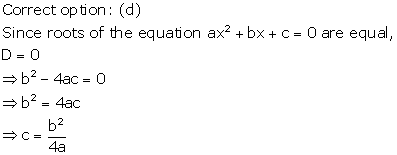
Solution 17
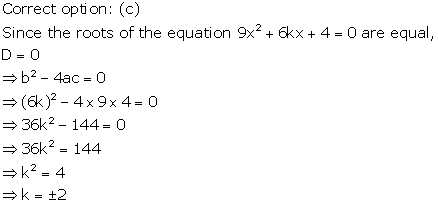
Solution 18
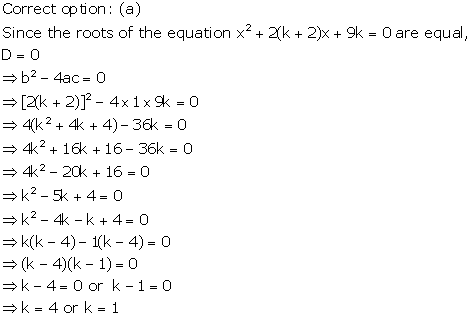
Solution 19
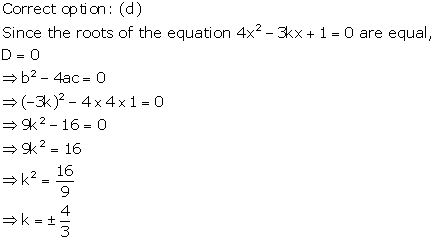
Solution 20
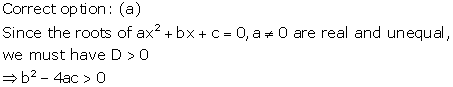
Solution 21
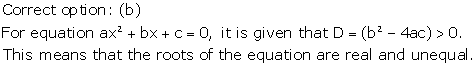
Solution 22
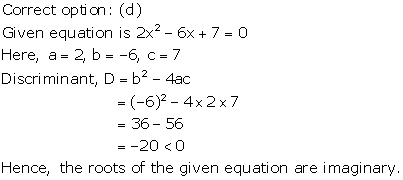
Solution 23
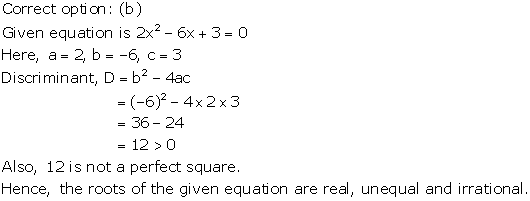
Solution 24
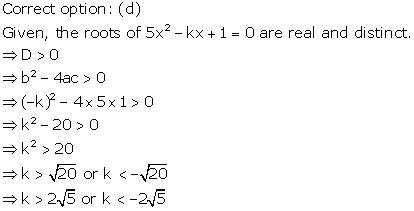
Solution 25

Solution 26
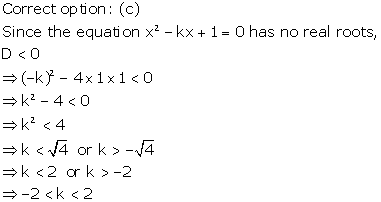
Solution 27
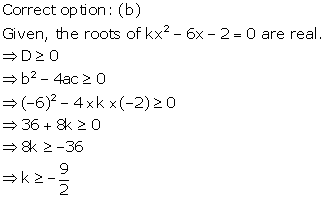
Solution 28
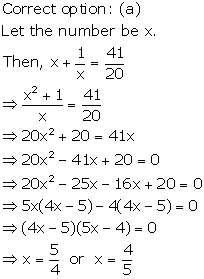
Solution 29
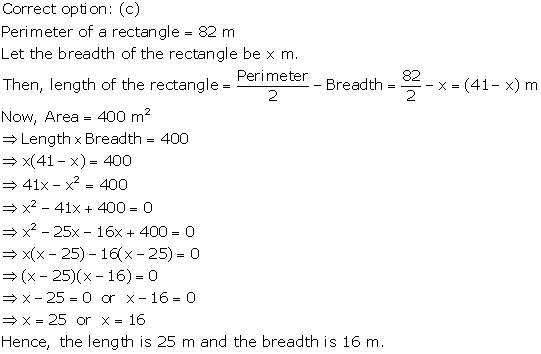
Solution 30

Solution 31
Correct option: (b)
Given equation is ![]() .
.
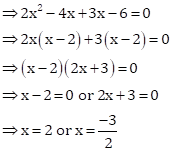
Solution 32
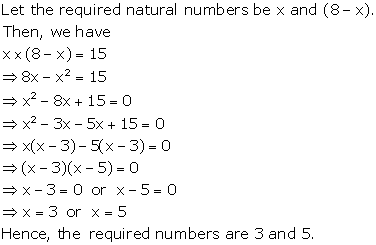
Solution 33
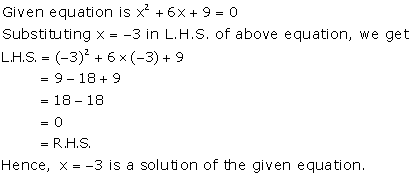
Solution 34

Solution 35
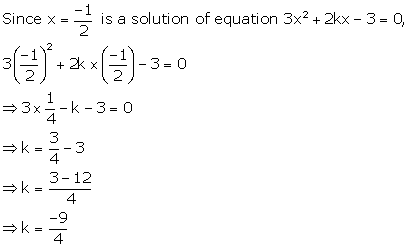
Solution 36
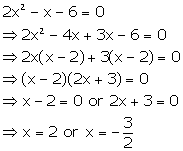
Solution 37
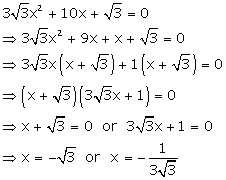
Solution 38
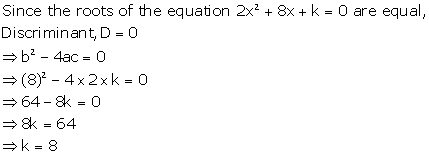
Solution 39
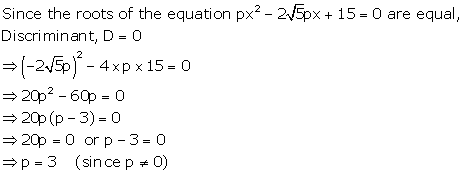
Solution 40
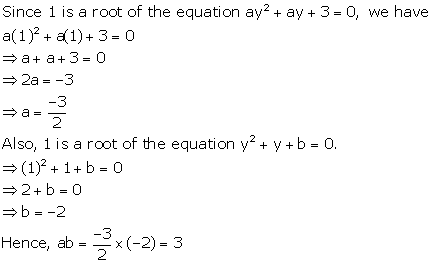
Solution 41
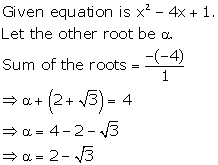
Solution 42

Solution 43
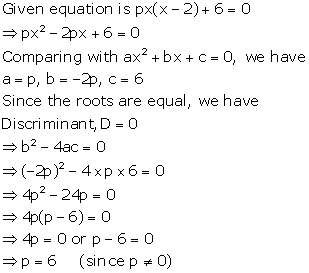
Solution 44
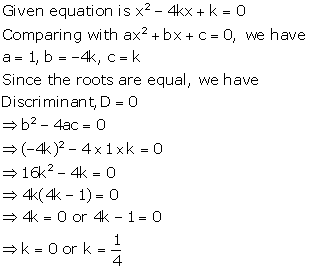
Solution 45
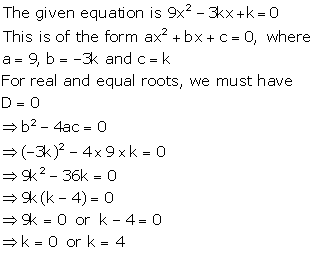
Solution 46

Solution 47
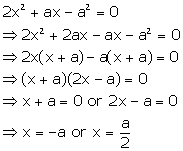
Solution 48
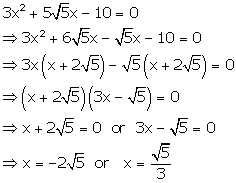
Solution 49
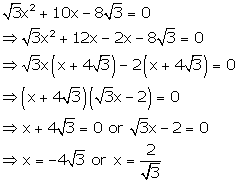
Solution 50

Solution 51
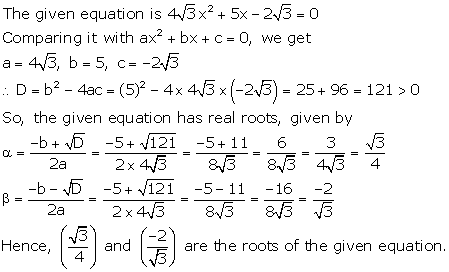
Solution 52
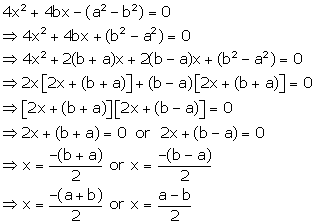
Solution 53
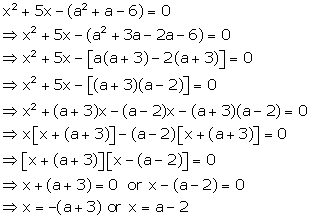
Solution 54

Solution 55