CBSE Class 10 Answered
if PT is drawn parallel to chord AB in a circle with centre O .prove that APB is an isoceles triangle
Asked by Murugan | 15 Mar, 2017, 06:23: PM
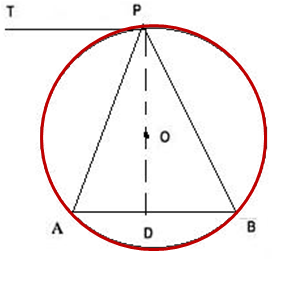
Construction: Join PO and produce it to D.
Now, OP is perpendicular TP (tangent makes a 90° angle with the radius of the circle at the point of contact)
Also, TP is parallel to AB (given)
angle ADP = 90° (interior angles)
So, OD is perpendicular to AB.
Since, a perpendicular drawn from the center of the circle to a chord bisects it,
PD is a bisector of AB. i.e. AD = DB
Now in triangle ADP and BDP
AB = DB (proved above)
angle ADP = angle BDP (both are 90°)
PD = DP (common)
Triangle ADP Triangle BDP (By SAS congruence criterion)
Triangle BDP (By SAS congruence criterion)
Hence, angle PAD = angle PBD (By CPCT)
Thus, APB is an isosceles triangle.
Answered by | 15 Mar, 2017, 06:30: PM
Application Videos
Concept Videos
CBSE 10 - Maths
Asked by aditikaldate7 | 21 Apr, 2024, 03:16: PM
CBSE 10 - Maths
Asked by pathaksuman622 | 21 Apr, 2024, 11:56: AM
CBSE 10 - Maths
Asked by yalagondanikhil | 16 Apr, 2024, 12:25: PM
CBSE 10 - Maths
Asked by rrajansinghakb199 | 08 Apr, 2024, 05:12: PM
CBSE 10 - Maths
Asked by nagalaxmidurgarao937 | 08 Apr, 2024, 01:42: PM
CBSE 10 - Maths
Asked by mraniruddha03 | 02 Apr, 2024, 06:44: PM
CBSE 10 - Maths
Asked by muttenenimalleswarrao | 29 Mar, 2024, 08:32: PM
CBSE 10 - Maths
Asked by pathakvaishnavi9139 | 23 Mar, 2024, 10:45: AM
CBSE 10 - Maths
Asked by mustafahssn786 | 23 Mar, 2024, 06:25: AM