Class 11-science H C VERMA Solutions Physics Chapter 5: Newton's Laws of Motions
Newton's Laws of Motions Exercise 79
Solution 1
From law of kinematics,
Solution 2
By law of kinematics ,
Solution 3
Solution 4
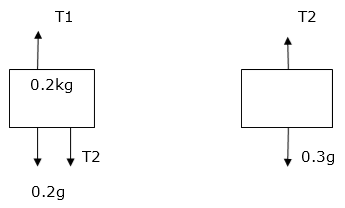
Solution 5
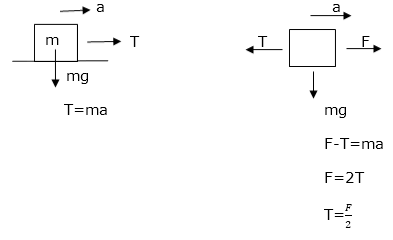
Solution 6
Slope of v-t graph gives acceleration,
t=25, a= slope
of straight line from t=0 to t=35=
=5m/s2
Solution 7
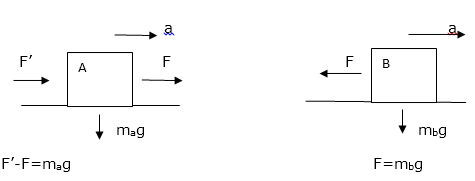
Solution 8
From 
Solution 9
(-ve sign =>
is opposite to displacement)
Solution 10
Solution 11
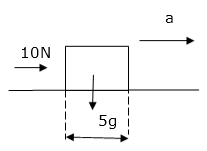
5a=10
a=2m/s2
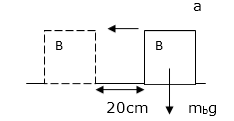
Solution 12
a)
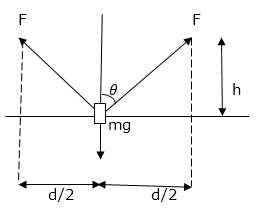
As man moves up, h
decrease 
As 
And F=Fmax=mg
b)
Newton's Laws of Motions Exercise 80
Solution 13
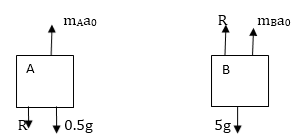
R=mAa0-0.5g
=0.5(2-10)=-4N
(-ve sign=> force acts opposite to our convention)
Solution 14
a) pseudo force acts downward
=> T=m(a0+g)=0.05(1.2+9.8)=0.55N
b)Fp (psend 
force) acts downward , but a0=-ve
=> T=m(-a0+g)=0.05(-1.2+9.8)=0.43N
c) Fb=0 as a0=0 => T=mg=0.05x9.8=0.49N
d)F0 acts upward => T=m(-a0+g)=0.43N
e) Fp acts upward but a=-ve
T=m(-(-a0)+g)
=0.05(1.2+9.8)=0.55N
f) Fp=0 as a0=0
=>T=mg=0.49N
Solution 15
W=Wmax (when Fp is along mg)
W=Wmin (when Fp is opposite to mg)
Wmax=m(a0+g)=72 ……………(1)
Wmin=m(-a0+g)=60 ……………(2)
a)Adding equation (1) and (2)
mg=
=66kg
b)Subtracting equation (1) and (2)
a0=
Solution 16
T- 1.5g-
=1.5a T-3g-
=-3a
T= 1.5g- +1.5a T=3g--3a
1.5g- +1.5a=3g--3a
4.5a=
(1.5)
a=
=3.593m/s2
Force on spring
Balance=2T
=2x(1.5a+
)
=43.1N
Mass (reading )
of spring=
=
=
=4.4kg
Solution 17
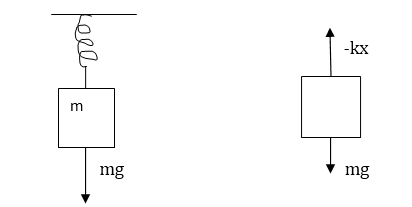
1st Case:
mg=-kx
x=
=
=-0.196m=-0.2m
2nd Case:
m=3kg
x'=
=-0.294m=-0.3m
|x'-x|=0.1m
Solution 18
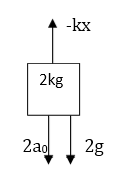
Solution 19
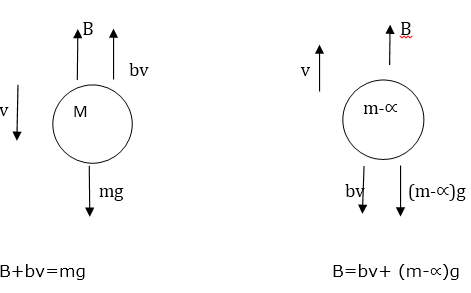
Bv is constant
Mg-B=B-( M-
)g
2Mg=2B+ g
=2
Solution 20
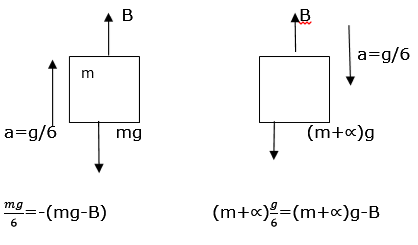
B force is constant
Solution 21
=
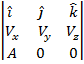To go undeflected 
,
ð V=Vmin if sin 
->1 =>
->90
ð i.e Vmin
along x- axis
Solution 22
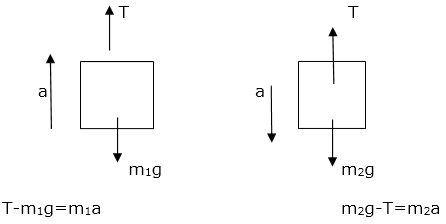
m1g +m2a=m2(g-a)
(m1+m2)a=(m2-m1)g
a) use x-x0=
b) T=m1(g+a)=m1(9.8+3.266)
=0.3(13.06)
=3.918N
c)F=2T=2x3.918=7.8N
Solution 23
Use law of kinematics v=u+at
v=0+3.26x2
=6.52m/s
m2 is moving down with v
m1 is moving down with v
at t=25, m2 stops
m1 moves upward and reaches v=0
v=0, u=6.52
0=u+at=6.52+(-9.8)t
t=
=0.66s
Solution 24
For 0.1m-> mA=10x0.10=1kg
For 0.2m-> mB=10x0.20=2kg
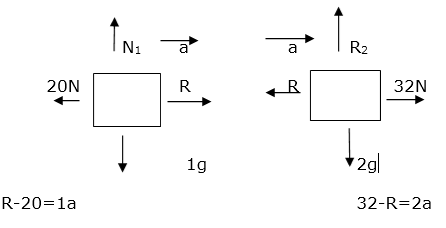
a+20=32-2a=>3a=12
=>a=4m/s2……………………{a is towards right as32N>20N}
R=20+1a=24N
Newton's Laws of Motions Exercise 81
Solution 25
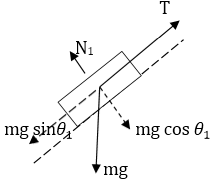
……….(1)
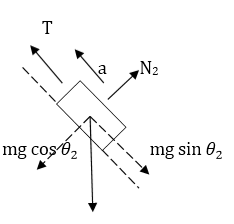
……….(2)
mg sin
ma = mg sin
+ma
g(sin
- sin)=2a
a=
Solution 26
Solution 27
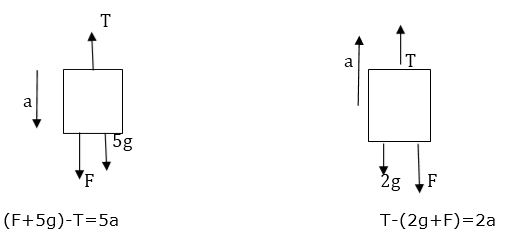
F+5g-5a=2a+2g+F
3g=7a =>a=
=4.2m/
After breaking T=0
m1a=F+5g=1+5g
a=
Solution 28
T=
………………………………………………(A)
…………………………….……………………(B)
Solving (A) and (B), we get
a1-a2 =
=
a1+a2=
a1 =
upward
a2 (acceleration of m2)=
down
a3 (acceleration of m3)=
down
using law of kinematics, S=ut+
a
Solution 29
a1=0
2T=m1g
T=m2(g-a2)……………………..T=m3(g+a2)
⇒ 
⇒ 
⇒
Solution 30
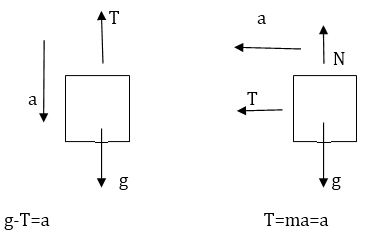
a=
=5m/
Solution 31
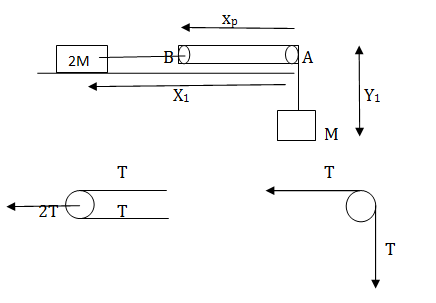
a) Take pulley A as reference print
length of string running through pulleys A,B
and mass M be 
{ is constant} 0=2
+
Length of string through pulley B and
mass 2M be 
⇒0=
{acceleration of 2M body =
}
(acceleration of mass M)= 
b) 
C)
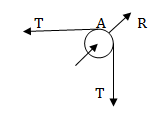
Horizontal Component is R cos 45
=
Solution 32
Take pulley A as reference point length of string through Mass M
(lAis constant)
Length of string through Mass 2M
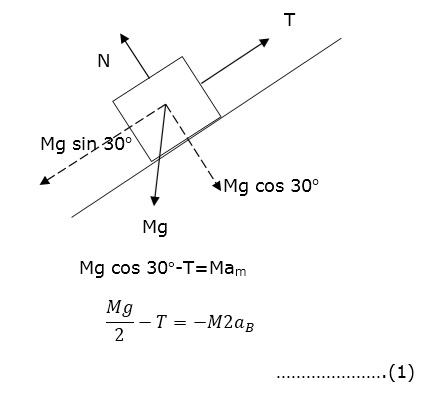
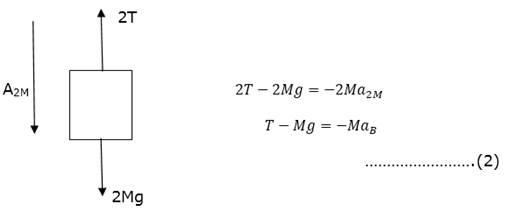
Solving (1) and (2), we get
(upward as our convention was downward, so we got a -ve sign)
Newton's Laws of Motions Exercise 82
Solution 33
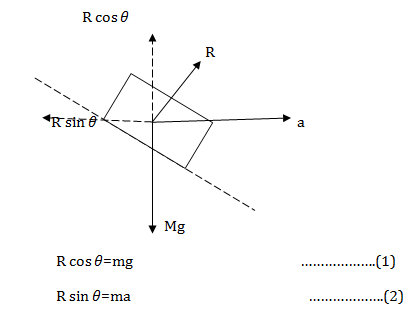
Solving (1) and (2),
a=g tan 
Solving (3) and (4)
R sin +M'a=Mg-Ma
Solution 34
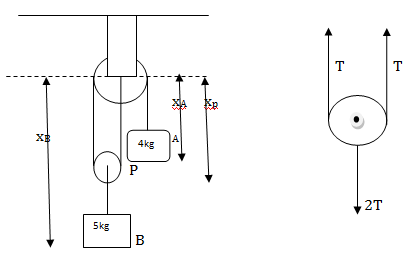
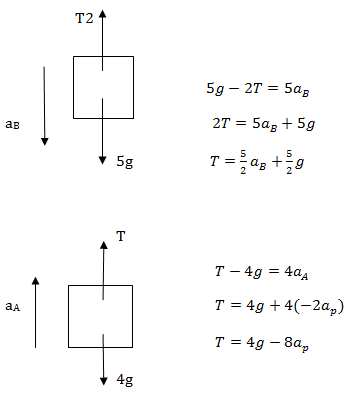
Length of string through mass A
Length of string through mass B
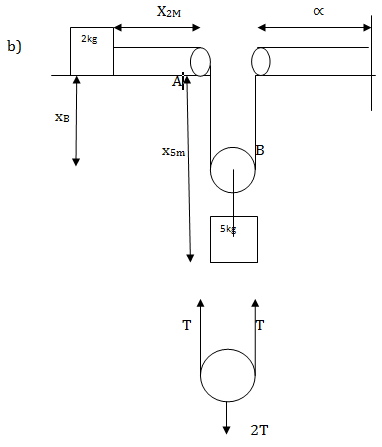
Pulley A= reference point
Length of string through 2kg mass
Length of string through 5kg mass
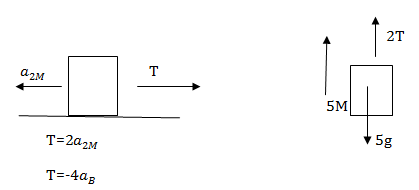
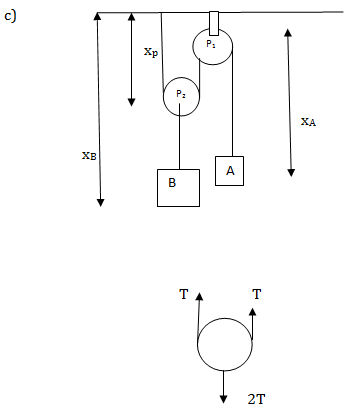
Length of string to A
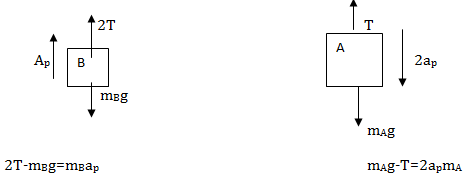
Solution 35
For 50g
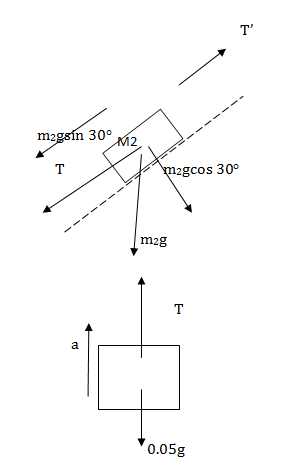
T-0.05g=0.05a ……………..(1)
T'-(T+ m2g sin 30°)= m2a
T'-T-0.05g=0.1a ………………(2)
0.5g-T'=0.5a ………………(3)
⇒ T'=0.5g-0.5a
Putting equation (3) in (2),
T=T'-0.05g-0.1a
=0.5g-0.5a-0.05g-0.1a
T=0.45g-0.6a
0.05a+0.05g=0.45g-0.60a
0.65a=0.4g
Solution 36
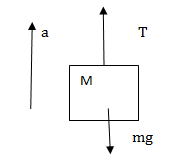
Fapplied=T
Use kinematics law, 
Solution 37
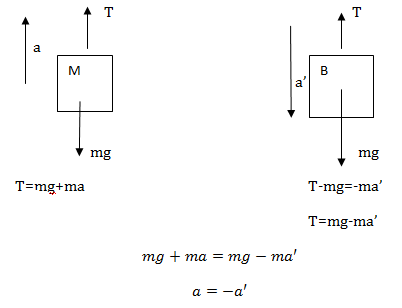
That means both are in same direction.
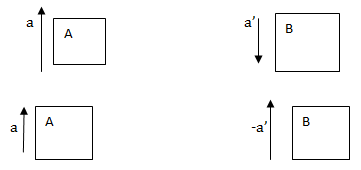
Solution 38
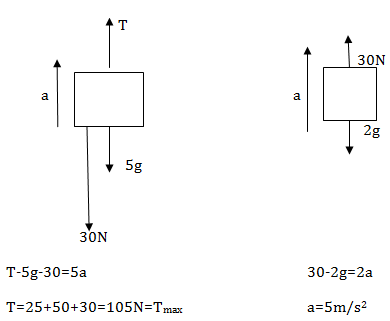
T=Tmin if a=0 so nem tension of second monkey
T'-2g=0
T'=20N
Tension of 1st Monkey ⇒ T=Tmin=5g+T'
=70N
Tmin=70NTmax=105N
Tmin<T<Tmax
Solution 39
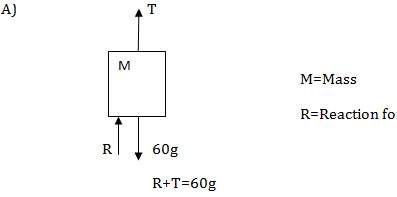
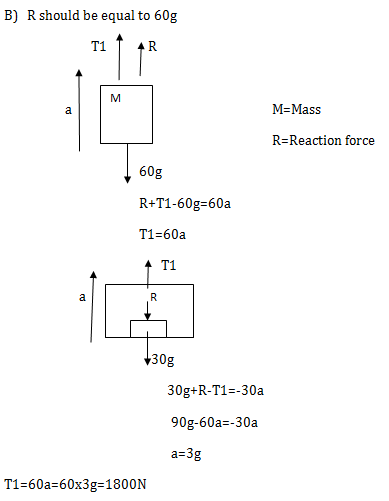
Newton's Laws of Motions Exercise 83
Solution 40
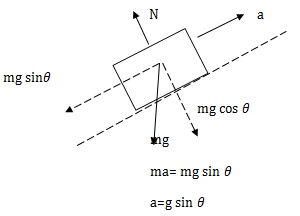
Use
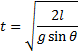
Solution 41
1)
T sin Ѳ = ma
T cosѲ = mg
2)
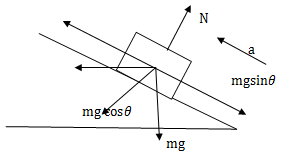
Solution 42
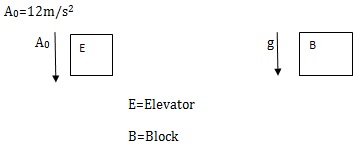
Block will be acted upon by g (only g)
