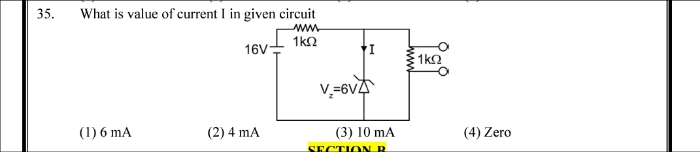
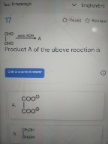
Doubts and Solutions
OR
CBSE XI Science - Maths
Asked by nitinkrjsr07082008 | 15 Apr, 2024, 11:32: PM
CBSE VIII - Maths
Asked by chauhanshivansh112 | 15 Apr, 2024, 08:41: PM
NEET NEET - Chemistry
Asked by raomayankup83 | 15 Apr, 2024, 07:46: PM
CBSE XII Science - Chemistry
Asked by ukg8612 | 15 Apr, 2024, 07:36: PM
ICSE VIII - Social Studies
Asked by krishnakantchoubey800 | 15 Apr, 2024, 06:00: PM
CBSE IX - Maths
Asked by gulshanelectronics2017 | 15 Apr, 2024, 05:35: PM
JEE Main - Maths
Asked by usakoyalshailesh | 15 Apr, 2024, 09:48: AM