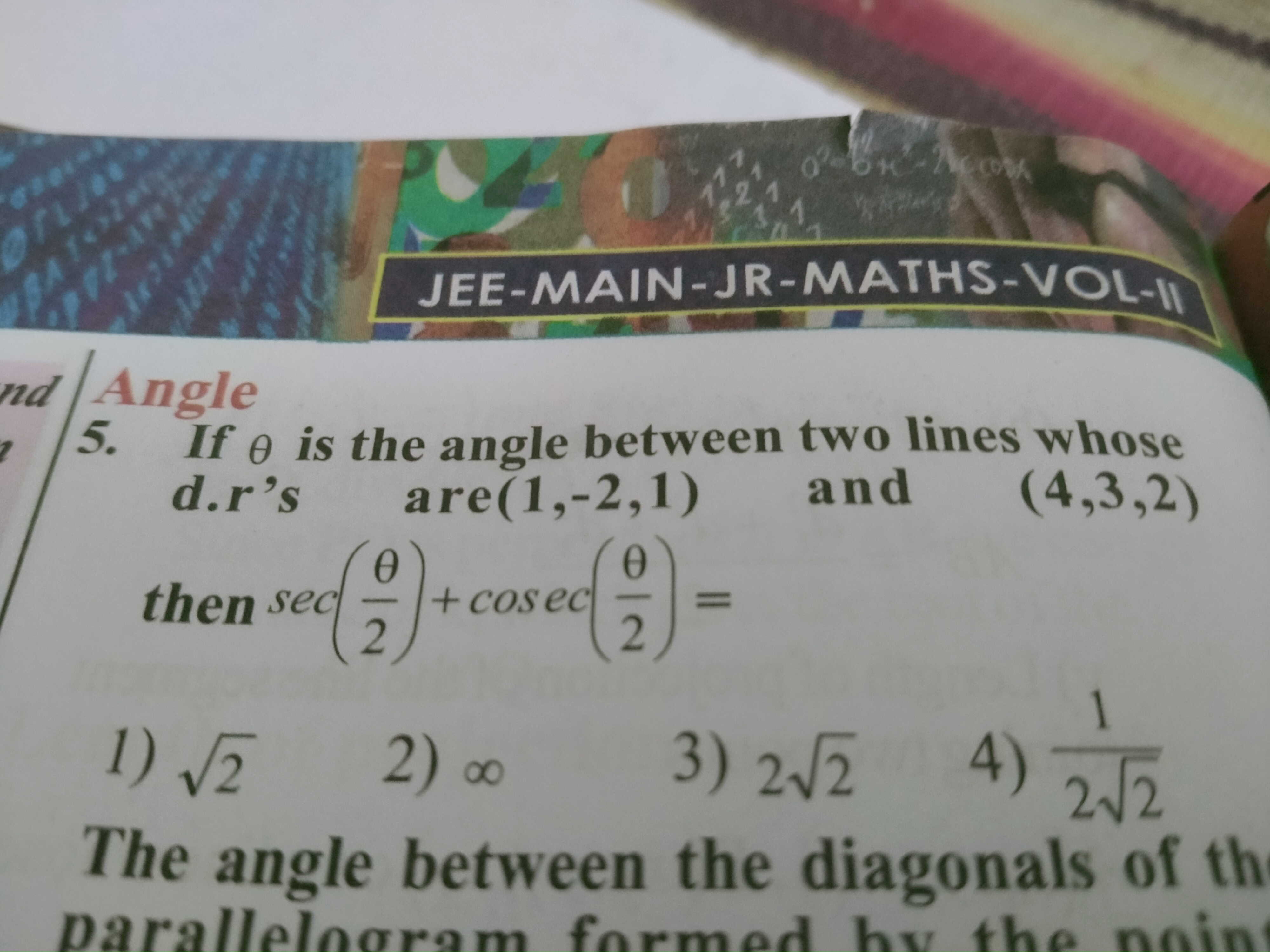Doubts and Solutions
OR
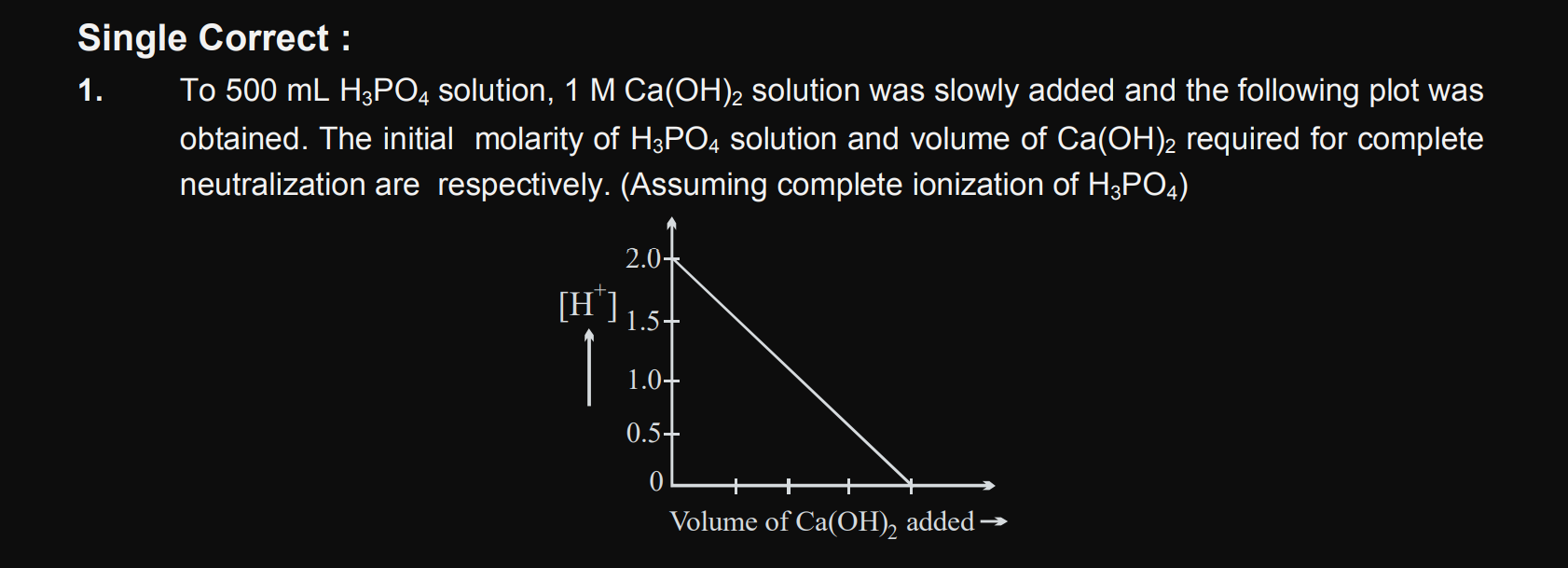
JEE Main - Chemistry
Asked by ashwinskrishna2006 | 17 Apr, 2024, 07:17: PM
NEET NEET - Biology
Asked by anandibastavade555 | 17 Apr, 2024, 07:08: PM
NEET NEET - Physics
Asked by gouranshi84 | 17 Apr, 2024, 05:23: PM
CBSE VII - Maths
Asked by shouryavijay0020012 | 17 Apr, 2024, 03:45: PM
CBSE XI Commerce - Accountancy
Asked by hydrogamerz.piyush | 17 Apr, 2024, 02:18: PM
CBSE VIII - Maths
Asked by japjigrewal54 | 17 Apr, 2024, 01:54: PM