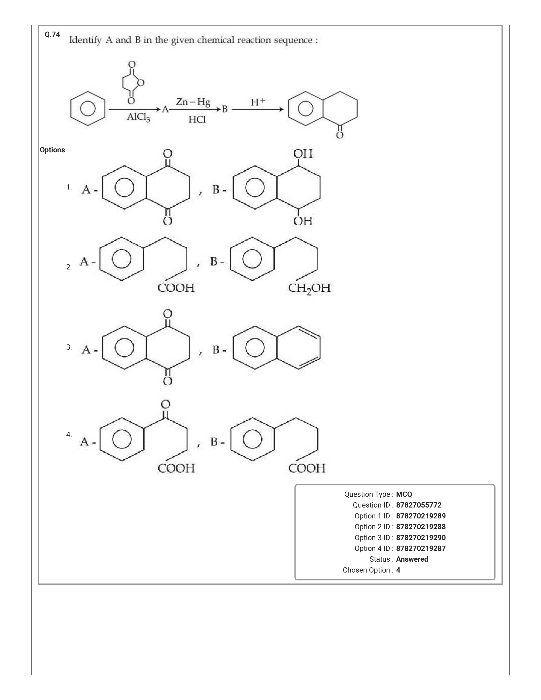
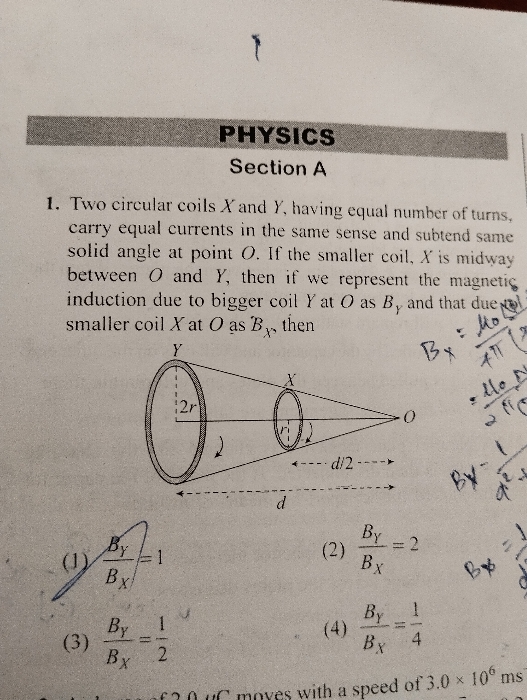
Doubts and Solutions
OR
NEET NEET - Chemistry
Asked by mahendar160786 | 16 Apr, 2024, 09:23: PM
CBSE VI - Grammar
Asked by beherajyoti253 | 16 Apr, 2024, 08:11: PM
CBSE XII Science - Maths
Asked by mahammadsharifdakhani67 | 16 Apr, 2024, 07:43: PM
CBSE VI - Social Studies
Asked by withmefun16 | 16 Apr, 2024, 07:21: PM
CBSE IX - History
Asked by nirajbhai228 | 16 Apr, 2024, 05:35: PM