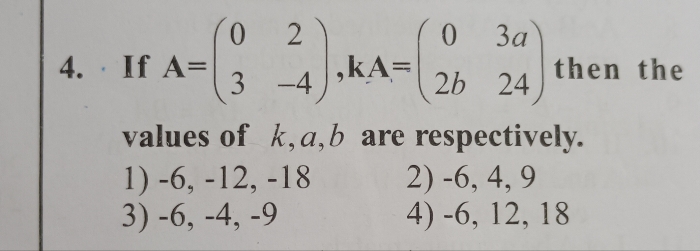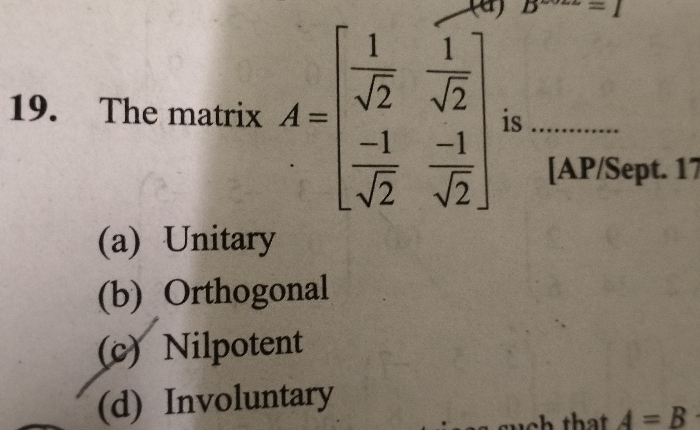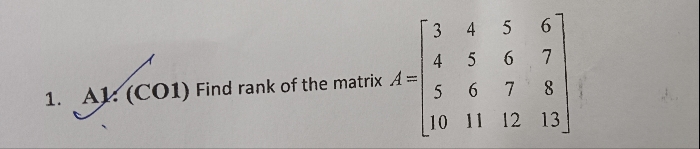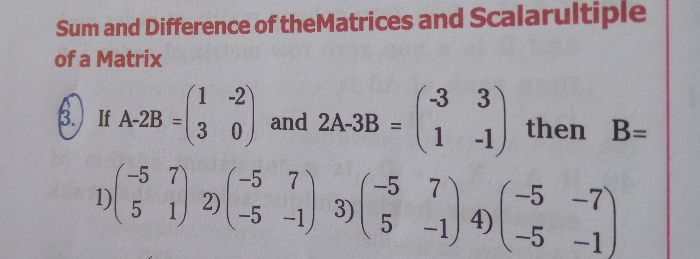the nos of 3X3 non singular matrices ,with4 entries as 1 and all other enterices as 0,is

JEE Class main Answered

the nos of 3X3 non singular matrices ,with4 entries as 1 and all other enterices as 0,is

Asked by mishrk261 | 28 Aug, 2019, 09:07: PM

Expert Answer

FIrst consider all the non-singular matrices with 3 entries as 1 and other 6 entries as 0

Now, for a 3 x 3 matrix to be non-singular, each row and column must have a non-zero value and that is 1

So, the possible matrices are:

open square brackets table row 1 0 0 row 0 1 0 row 0 0 1 end table close square brackets space space open square brackets table row 0 1 0 row 1 0 0 row 0 0 1 end table close square brackets space space open square brackets table row 0 1 0 row 0 0 1 row 1 0 0 end table close square brackets space space open square brackets table row 0 0 1 row 0 1 0 row 1 0 0 end table close square brackets space space open square brackets table row 1 0 0 row 0 0 1 row 0 1 0 end table close square brackets space space open square brackets table row 0 0 1 row 1 0 0 row 0 1 0 end table close square brackets

Now, in each of these possibilities, we can make any of the 6 0's into 1.

So, for each matrix there are 6 possibilities

Hence, the total number of possibilities = 6 x 6 =36

Answered by Renu Varma | 30 Aug, 2019, 10:14: AM

Answered Unanswered My Questions My Answers

JEE main - Maths

could you solve the following question from complex number topic?

Asked by ashwinskrishna2006 | 13 Apr, 2024, 11:25: AM

ANSWERED BY EXPERT

JEE main - Maths

process of the solution

Asked by vidyanath.j | 11 Apr, 2024, 09:28: PM

ANSWERED BY EXPERT

JEE main - Maths

ans

Asked by raghupaladi09 | 02 Apr, 2024, 08:19: PM

ANSWERED BY EXPERT

JEE main - Maths

in a 3 cross 3 square Matrix the elements of a column are 2,5k+1,3 and co factors of another column are 1-5k,2,4k-2

Asked by kavitha8wardmember | 25 Mar, 2024, 12:20: PM

ANSWERED BY EXPERT

JEE main - Maths

solve

Asked by bhatimahipalsingh21 | 11 Mar, 2024, 08:49: PM

ANSWERED BY EXPERT

JEE main - Maths

if a= 1 2 2 2 1 2 show that A2-4A-5i=0 2 2 1

Asked by dkittu40 | 09 Oct, 2023, 10:24: AM

ANSWERED BY EXPERT

JEE main - Maths

tell this answer

Asked by nlsa2007jul | 26 Mar, 2023, 11:00: AM

ANSWERED BY EXPERT

JEE main - Maths

help me in this

Asked by sunithadevi6666 | 17 Jun, 2022, 07:22: AM

ANSWERED BY EXPERT

JEE main - Maths

in determinants what is mean by r2-r1-r3 and how it is solved explain with some examples

Asked by divyapatel8985 | 29 Jan, 2022, 08:27: PM

ANSWERED BY EXPERT

JEE main - Maths

kindly answer this question

Asked by sharmasaumya453 | 16 Sep, 2020, 03:07: PM

ANSWERED BY EXPERT

Please Login or Sign up to continue!

JEE Class main Answered

Choose Filters

Open In Ask A Doubt App ?

Get in Touch

Call / Whats App