Prove that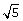 
is an irrational number.
Asked by Topperlearning User
4th June 2014,
1:23 PM
Answered by Expert
Answer:
Let us assume, on the contrary that 
is a rational number.
Therefore, we can find two integers a, b (b 
0) such that 
where a and b are co-prime integers.
Therefore, a2 is divisible by 5 then a is also divisible by 5. So a = 5k, for some integer k.
This means that b2 is divisible by 5 and hence, b is divisible by 5.
This implies that a and b have 5 as a common factor.
And this is a contradiction to the fact that a and b are co-prime.
So our assumption that is rational is wrong.
Hence,cannot be a rational number. Therefore, is irrational.
Answered by Expert
4th June 2014,
3:23 PM
Rate this answer
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
You have rated this answer /10
Your answer has been posted successfully!
RELATED STUDY RESOURCES :
- CBSE Sample Papers for Class 10 Mathematics
- R S Aggarwal and V Aggarwal Textbook Solutions for Class 10 Mathematics
- RD Sharma Textbook Solutions for Class 10 Mathematics
- NCERT Textbook Solutions for Class 10 Mathematics
- CBSE Syllabus for Class 10 Mathematics
- CBSE Previous year papers with Solutions Class 10 Mathematics
Browse free questions and answers by Chapters
- 1 Pair of Linear Equations in 2 Variables
- 2 Quadratic Equations
- 3 Arithmetic Progression
- 4 Triangles
- 5 Some Applications of Trigonometry
- 6 Polynomials
- 7 Coordinate Geometry
- 8 Introduction to Trigonometry
- 9 Circles
- 10 Constructions
- 11 Areas Related to Circles
- 12 Surface Areas and Volumes
- 13 Statistics
- 14 Probability
- 15 Real Numbers
Trending Tags
Latest Questions
JEE Plances JEE (Main) Mathematics
Asked by pks441006
11th December 2018,
1:55 PM
NEET1 NEET1 Physics
Asked by Deepak
11th December 2018,
12:37 PM
CBSE VII Civics
Asked by Yatharthbhadoriya
11th December 2018,
12:35 PM
