Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

CBSE Class 12-science Answered

An aeroplane can carry a maximum of 200 passengers. A profit of Rs 400 is made on each first class ticket and a profit of Rs 300 is made on each economy class ticket. The airline reserves at least 20 seats for first class. However, at least 4 times as many passengers prefer to travel by economy class to by the first class. Determine how many each type of ticket must be sold in order to maximum the profit for the airline. What is maximum profit?
Asked by Topperlearning User | 31 Jul, 2016, 07:21: PM
answered-by-expert Expert Answer

Let x and y be the numbers of first class tickets and economy class tickets respectively we have the following LPP

 


Maximum 400x + 300y
          st x + y  200
          x  20
          y  4x
          x  0, y  0 

 

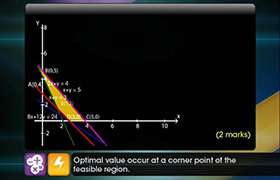
Let us draw the graph of the above LPP

 

 


 

Thus, the feasible region is ABC and the corner points are A(20,180), B(40,160) and C(20,80)

 

The value of the objective function at the corner points are:

 

table row cell C o r n e r space p o i n t end cell cell V a l u e space o f space Z equals 400 x plus 300 y end cell row cell A left parenthesis 20 comma 180 right parenthesis end cell 62000 row cell B left parenthesis 40 comma 160 right parenthesis end cell 64000 row cell C left parenthesis 20 comma 80 right parenthesis end cell 32000 end table

 

The maximum of the objective function is 64000 and it attains at A(40,160).

 

That is 40 first class tickets and 160 economy class tickets must be sold to get the maximum profit.

 


 


 


 

 

 

Answered by | 31 Jul, 2016, 09:21: PM

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
CBSE 12-science - Maths
using graph, maximize z=4x+3y subject to the constraints x+y=9, 9x+2y=18
Asked by gargisha381 | 26 May, 2022, 12:24: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
in ncert chapter 12 exercise 1 question no. 2 why we cant take points of x+2y=8 ... -24 should be the minimum value which comes from the point (8,0)..
Asked by ABHILASHA | 20 Apr, 2021, 12:07: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
Two godowns A and B have grain capacity of 100 quintals and 50 quintals respectively. They supply to 3 ration shops D, E and F whose requirements are 60, 50 and 40 quintals respectively. The cost of transportation per quintals form the godowns to the shops are given in following table To/Form A B D E F 6 3 2.5 4 2 3 How would the supplies be transported in order that the transportation cost is minimum. What is the minimum cost?
Asked by Topperlearning User | 22 Sep, 2014, 10:45: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
An oil company has two depots A and B with capacities 7000 and 4000 respectively. The company is to supply oil to three petrol pumps. D, E and F whose requirements are 4500 , 3000 and 3500 respectively. The distance (in km) between the depots and petrol pump is given as follows:   To/From A B DEF 763 342 How would the supplies be transported in order that the transportation cost is minimum? What is the minimum cost?
Asked by Topperlearning User | 12 Sep, 2014, 03:47: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
An aeroplane can carry a maximum of 200 passengers. A profit of Rs 400 is made on each first class ticket and a profit of Rs 300 is made on each economy class ticket. The airline reserves at least 20 seats for first class. However, at least 4 times as many passengers prefer to travel by economy class to by the first class. Determine how many each type of ticket must be sold in order to maximum the profit for the airline. What is maximum profit?
Asked by Topperlearning User | 31 Jul, 2016, 07:21: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
A brick manufacturer has two depots, A and B, with stocks of 30,000 and 20.000 bricks respectively. He receives orders from three builders P, Q and R for 15,000, 20,000 and 15,000 bricks respectively. The cost in Rs of transporting 1000 bricks to the builders from the depots are given below :                  From To P Q R A 40 20 30 B 20 60 40           How should the manufacturer fulfil the orders so as to keep the cost of transportation minimum ? Formulate the above linear programming problem.
Asked by Topperlearning User | 31 Jul, 2016, 06:04: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
An aeroplane can carry a maximum of 200 passengers. A profit of Rs. 1000 is made on each executive class ticket and a profit of Rs. 600 is made on each economy class ticket.  The airline reserves at least 20 seats for executive class.  However, at least 4 times as many passengers prefer to travel by economy class than by the executive class. Determine how many tickets of each type must be sold in order to maximize the profit for the airline.  What is the maximum profit?
Asked by Topperlearning User | 31 Jul, 2016, 05:58: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
There is a factory located at each of the two places  P and Q. From these location, a certain commodity is delivered to each of these depots situated at A, B and C. The weekly requirements of the depots are respectively 5, 5 and 4 units of the commodity while the production capacity of the factories at P and Q are respectively 8 and 6 units. The cost of transportation per unit is given below :   To From                                     Cost (in Rs) A B C P 16 10 15 Q 10 12 10   How many units should be transported from each factory to each depot in order that the transportation cost in minimum. Formulate the above LPP mathematically and the solve it.
Asked by Topperlearning User | 31 Jul, 2016, 05:47: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
A toy company manufactures two types of dolls A and B. Resources indicate that the combined production level should not exceed 1200 dolls per week and the demand for dolls of type B is atmost half of that for dolls of type A. Further the production level of dolls of type A can exceed three times the production of dolls of other type of atleast 600 units. If the company makes profit of Rs 12 and Rs 16 per doll respectively on dolls A and B. How many each should be produced weekly in order to maximize profit?
Asked by Topperlearning User | 31 Jul, 2016, 08:05: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
A company manufactures two types of souveniers. Souvenier of type A requires 5 minutes each for cutting and 10 minutes each for assembling souvenier B requires 8 minutes each of cutting and 8 minutes each for assembling. There are 3 hours 20 minutes available for cutting and 4 hours for assembling. The profit is Rs 5 each for type A and Rs 6 each for type B souvenier. How many souvenier of each type should the company manufactures in order to maximize the profit.
Asked by Topperlearning User | 04 Jun, 2014, 01:23: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt
Get Latest Study Material for Academic year 24-25 Click here
×