Please wait...
Contact Us
Contact
Need assistance? Contact us on below numbers

For Study plan details

93219 24448 / 89292 63204

10:00 AM to 7:00 PM IST all days.

For Franchisee Enquiry

81783 72169
OR

Chat with us on WhatsApp

or

Thanks, You will receive a call shortly.
Customer Support

You are very important to us

For any content/service related issues please contact on this number

93219 24448 / 99871 78554

Mon to Sat - 10 AM to 7 PM

Home / Doubts and Solutions/NEET/Physics

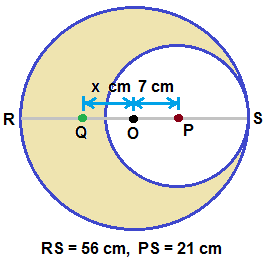
A circular plate of uniform thickness has a diameter of 56 cm. A circular portion of diameter 42 cm is removed from one edge of the plate. Find the position of the centre of mass of the remaining portion.

Asked by nasir.mirza 7th June 2018, 10:27 AM
Answered by Expert
Answer:
Figure shows the circular disc of diameter 56 cm after a circular portion of diameter 42 cm is removed.
 
We know that point O is centre of mass for the full disc before removing the above mentioned circular portion.
 
we take the point O as reference to calculate the centre of mass by considering the full disc is made of two objects, i.e., the disc showed as coloured one(white colour circular portion is removed) and the removed white colour circular portion. Let point Q which is centre of mass of coloured object, be at a distance x cm from point O.
 
(m1 × x + m2 × 7 )/(m1+m2) = 0 ..........................(1)
 
where m1 and m2 are masses of coloured object and white circular disc respectively.
We have written 0 in right hand side of eqn.(1) because we took point O which is centre of mass of full circular disc as reference point
 
from eqn.(1) we get x = - (m2×7)/m1...............(2)
 
mass of circular objects are proportional to square of diameter because thickness and density are same.
 
hence we write eqn.(2) as ,   x = -(42×42×7) / [ (56×56)-(42×42)] = -9 cm
Answered by Expert 8th June 2018, 8:27 AM
Rate this answer
  • 1
  • 2
  • 3
  • 4
  • 5
  • 6
  • 7
  • 8
  • 9
  • 10

You have rated this answer 10/10

Report an issue
Tags: centre-of-mass
Previous Question
Next Question
Your answer has been posted successfully!

Free related questions

masses 8,2,4,2 kg are placed at the corners A,B,C,D of a square ABCD whose diagonal is 80cm . the distance of COM from A is   
28th February 2022, 1:15 PM
A tube of mass 2m and radius 'R' is kept on smooth horizontal surface. A small sphere of mass m kept inside tube is imparted velocity v_{0} along y axis as shown in figure. The sphere just fits into the tube. Motion of particle and tube take place in horizontal plane and there is no friction at any contact. Then, speed of particle when it reaches point B is
17th March 2022, 9:21 PM
Two particles having mass ratio n : 1 are interconnected by a light inextensible string that passes over a smooth pulley. If the system is released, then the acceleration of the centre of mass of the system is
9th April 2022, 12:33 PM
Two particles having mass ratio n : 1 are interconnected by a light inextensible string that passes over a smooth pulley. If the system is released, then the acceleration of the centre of mass of the system is
9th April 2022, 12:30 PM
upper question
20th February 2024, 11:42 PM
RELATED STUDY RESOURCES :
NEET QUESTION ANSWERS

Browse free questions and answers by Chapters

Trending Tags

trigonometric equations Mole concept linear equations circle trigonometric ratios functions matrix operations integration arithmetic progression quadratic equation

Latest Questions

CBSE X Biology
What is enzyme  
Asked by Sureshgowdayd548 28th October 2020, 10:50 PM
JEE Main Mathematics
The following pseudocode is executed using the “Paragraph words” dataset. At the end of the execution, CountB captures the number of verbs with letter count less than the average letter count of verbs. But the pseudocode may have mistakes in one or more lines. Identify all such lines (if any). It is a Multiple Select Question (MSQ).  Error in Line 4  Error in Line 8  Error in Line 11  Error at Line 12  Error in Line 17  Error at Line 18  Error in Line 21 The following pseudocode is executed using the “Words” table. What will be the value of A at the end of the execution?
Asked by amaladelsingh 28th October 2020, 10:24 PM
CBSE X Biology
I want a brief clarity on Taxonomy
Asked by reenacharan2008 28th October 2020, 9:11 PM

Chat with us on WhatsApp