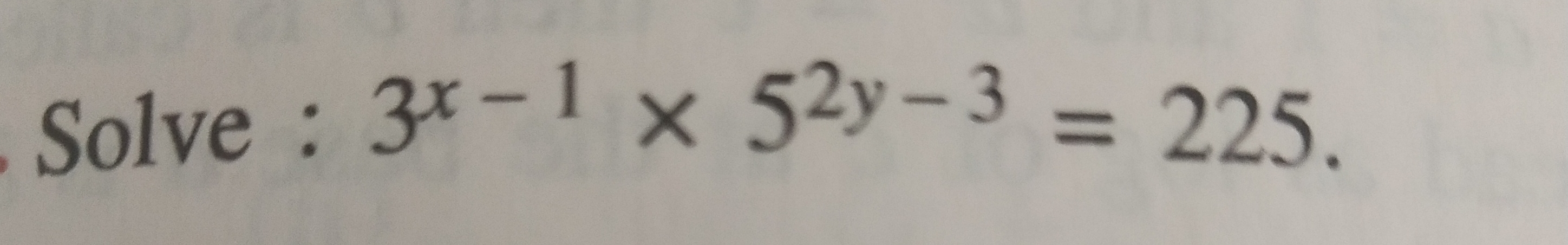ICSE Class 9 Answered
why root 2 is not a rational number
Asked by rkarim957 | 17 Mar, 2019, 07:41: PM
let us consider √2 is a rational number .
Then we can write, √2 = a / b .............................(1)
where a and b are co-prime, i.e., there is no common factor between a and b .
By squaring both sides of eqn.(1), we get, a2 / b2 = 2 .................(2)
From eqn.(2), it appears b2, which is an integer, is a factor of a .
[ if a square of integr number x is divisible by integer y, i.e., x2 is divisible by y, then y is a factor of x ]
But we started with the assumption a is prime number.
We get into this contradiction because of our assumption √2 is a rational number.
Hence √2 is not a rational number. √2 is an irrational number
Answered by Thiyagarajan K | 17 Mar, 2019, 09:58: PM
Application Videos
Concept Videos
ICSE 9 - Maths
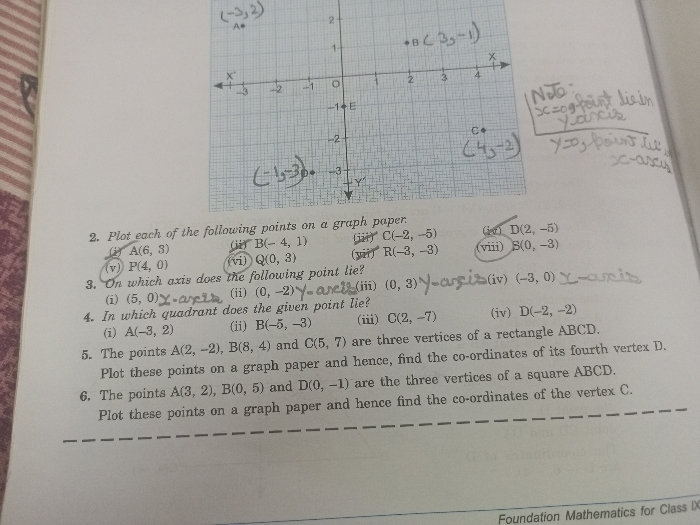
Asked by shantanupandey271208 | 21 Apr, 2024, 03:03: PM
ICSE 9 - Maths
Asked by harshansingh92 | 09 Apr, 2024, 06:10: PM
ICSE 9 - Maths
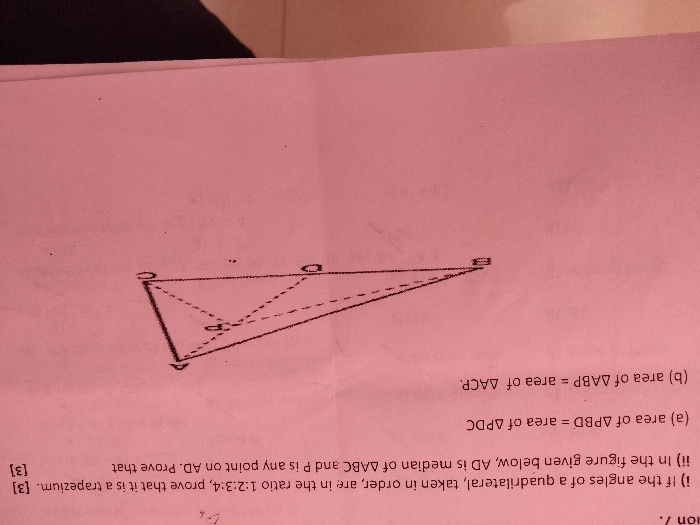
Asked by patnamprakash545 | 07 Apr, 2024, 05:23: PM
ICSE 9 - Maths
Asked by ysubha24 | 06 Apr, 2024, 06:24: AM
ICSE 9 - Maths
Asked by jahnvi | 31 Mar, 2024, 01:10: PM
ICSE 9 - Maths
Asked by papunsarkar | 28 Mar, 2024, 08:30: AM
ICSE 9 - Maths
Asked by djhsm.sarah.hooda.sajid.nadiya | 25 Mar, 2024, 05:33: PM