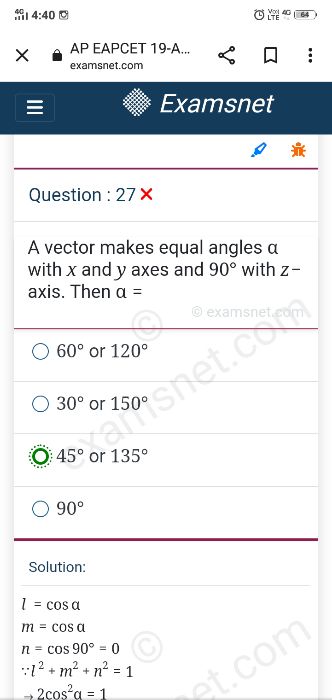
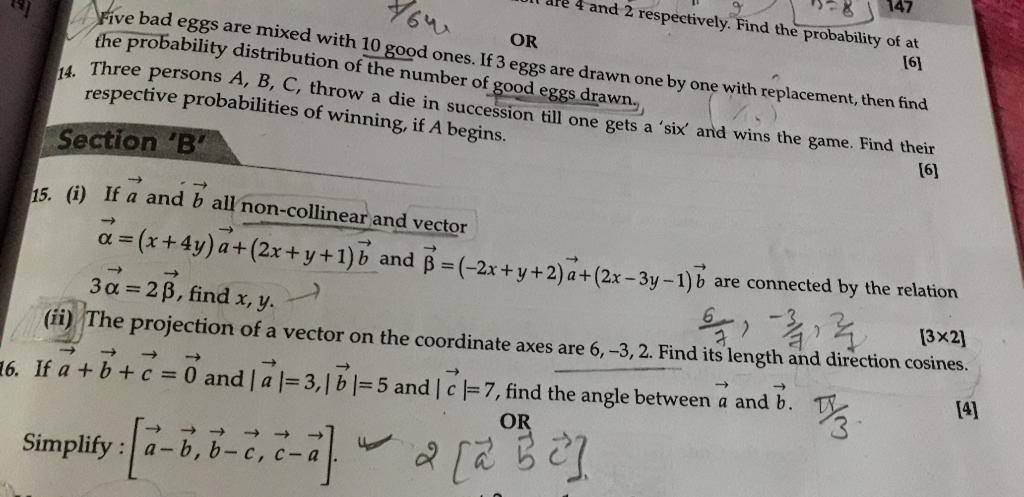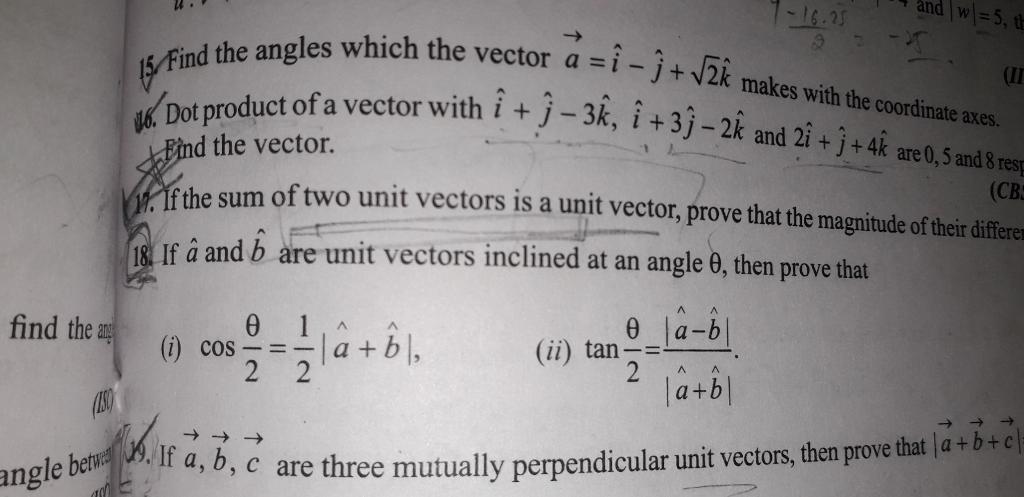CBSE Class 12-science Answered
Using vectors prove that angle inscribed in a semi circle is a right angle
Asked by DIVYA GARG | 06 Jun, 2013, 01:36: PM
Expert Answer
Center the circle at the origin, and scale to have radius 1. Let the vertex of the right triangle be at vector v, and let the diameter be the segment from the vector w to ?w.
Then (v?w)?(v?(?w))=(v?w)?(v+w)=(v?v)?(w?w)=1?1=0, so the angle formed by vw and v(?w) is a right angle.
Answered by | 07 Jun, 2013, 01:37: AM
Concept Videos
-
Vector multiplication concepts
Multiplication of a vector by a scalar, vector joining two points. Directio... -
Cross product
This video explains the properties of vector product especially cross produ... -
Introduction to vector algebra
This video explains the definition of vector and its types, direction angle... -
Concepts and application on properties of vector ...
Multiplication of a vector by a scalar, vector joining two points. Directio... -
Concepts on Vector cross product
Vector or cross product. Properties of vector product.
CBSE 12-science - Maths
Asked by sdmbotch1123 | 18 Jan, 2023, 11:01: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
If |𝑎⃗| = 3 and −1 ≤ 𝑘 ≤ 2, then |𝑘𝑎⃗| lies in the interval
(a) [0,6] (b) [−3,6] (c) [3,6] (d) [1,2]
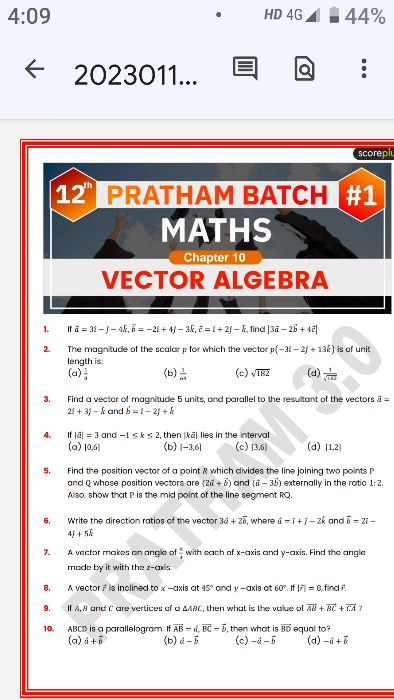
Asked by mohdtayyab682 | 18 Jan, 2023, 10:34: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by durgaprasaddurgaprasad174 | 01 Jul, 2022, 05:05: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by sujaychandramouli | 24 May, 2022, 10:46: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by abhimeena1011 | 18 Jan, 2022, 10:43: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by gurupadam3101985 | 11 Apr, 2020, 10:06: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by lovemaan5500 | 07 Feb, 2020, 09:49: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by lovemaan5500 | 07 Feb, 2020, 09:49: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by lovemaan5500 | 16 Dec, 2019, 09:34: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by Vivek.d8765 | 29 Oct, 2019, 10:14: PM
ANSWERED BY EXPERT
