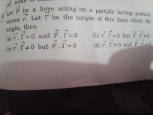Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

CBSE Class 11-science Answered

There is a disc of radius R. A small disc is cut from that disc such that the centre of first disc lies on the circumference of the second one. Find out the centre of mass of the remaining disc.
Asked by Anvita Chaudhary | 18 Oct, 2013, 05:43: PM
answered-by-expert Expert Answer
The disc of radius R has mass say M.
Now a disc is cut out such that centre of disc of radius R lies on circumference of the disc that is cut out. Hence, the radius of this disc will be R/2.
 
 
The centre of mass of big disc will be at its centre C. We consider this point as origin. Hence, coordinates of C are (0,0)
The centre of mass of small disc will be at its centre which is a distance R/2 away from C. Hence, coordinates of C1 are (R/2,0).
After the disc is cut, the centre of mass shifts. Let the coordinates of new centre of mass be (x,0).
 
Let the mass per unit area of big disc be m = M/?R2.
 
Thus, mass of the removed part is = M1 = m(?R2/4) = M/4.
Hence, mass of remaining part of disc is M2 = M - M/4 = 3M/4.
 
Thus, the position of centre of mass of whole disc can be written as
 
 
Hence, centre of mass of remaining disc shifts to the left of original centre C.
Answered by Romal Bhansali | 22 Oct, 2013, 09:41: AM

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
CBSE 11-science - Physics
obtain the expression for time period of simple pendulum performing shm.
Asked by rustampathan | 25 Feb, 2024, 06:39: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
explain how angular momentum can be expressed as the vector product of two vectors.how is it's direction determined discuss the cases of minimum and maximum angular momentum
Asked by gipaalsy | 08 Feb, 2024, 05:41: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
t
question image
Asked by rkdubey133 | 19 Jan, 2024, 10:03: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
rotational motion
question image
Asked by priyankaprajapti81 | 04 Nov, 2023, 10:47: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
please answer
question image
Asked by gdeadshot85 | 14 Jan, 2023, 09:58: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
three diSc each of mass m and radius r placed in contact with each otheer find moment of inertia
Asked by devanshchauhan325 | 23 Dec, 2022, 07:34: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
Prove that Torque acting due to a Force F in the x-y plane is Tau z=xfy-yfx.
Asked by koushtubhroy | 20 Dec, 2022, 07:36: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
one meter long string can bear maximum of 0.5 kg mass . a mass of 0.05 kg is tied to one end and rotated in a horizontal circle , calculate the maximum number of revolution so that string does not brakes
Asked by pushpakumari291279 | 20 Aug, 2022, 08:15: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
A Uniform Disc of diameter R/2 is put over another uniform disc of radius R of same thickness and Density. The peripheries of the two discs touch each other. The Centre of Mass of the system from centre of the big disc is? (I asked this question before but the answer was wrong. They took the Radius as R/2 while it is the Diameter)
Asked by adipadmakarri | 26 Sep, 2021, 06:08: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
A Uniform Disc of diameter R/2 is put over another uniform disc of radius R of same thickness and Density. The peripheries of the two discs touch each other. The Centre of Mass of the system from centre of the big disc is?
Asked by adipadmakarri | 25 Sep, 2021, 11:18: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt
Get Latest Study Material for Academic year 24-25 Click here
×