Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

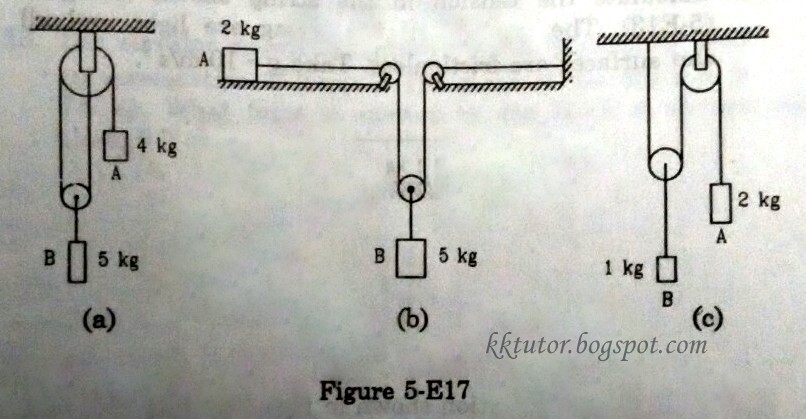
CBSE Class 11-science Answered

The potential energy of a certain field has the form U=a/r^2-b/r,where a and b are positive constants, r is the distance from the centre of the field. Find the value of R corresponding to the equilibrium position of the particle; examine whether this position is stable.
Asked by devanshu_jain | 19 Oct, 2010, 07:26: PM
answered-by-expert Expert Answer
Dear Student,
The equilibrium position is one with minimum potential energy, hence,
dU/dr = 0
-2ar-3 + br-2 = 0
r = 2a/b
r = R = 2a/b
To investigate the stability of this position, we need to find the curve is concave or convex at R.
d2U/dr2 = 6ar-4 -2br-3
at r = R
d2U/dr2 = 6a(2a/b)-4 -2b(2a/b)-3
= 6b4/(16a3) -2b4/(8a3)
= b4/(8a3) > 0
Hence the this position is concave and stable.
regards,
Team,
TopperLearning.
Answered by | 19 Oct, 2010, 10:12: PM

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
CBSE 11-science - Physics
Can you tell an example about a body having 0 velocity and still accelerating?
Asked by veenasangeeth2020 | 30 Jan, 2024, 09:56: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
mention the expression for work done
Asked by abdulkhadirchajjo549 | 06 Dec, 2023, 06:55: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
During calculation of acceleration due to gravity above the surface of earth if we assume that there is an external force opposite to that of Earth's gravity then the cos angle will be 180 between the two forces which is negative(-1), if we put the value in the equation of dw = F.dx then the final value of potential energy is positive which is not possible.how and why??
Asked by anushkaverma.25707 | 24 Aug, 2023, 07:35: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
In the problem no. 34 of concepts of physics HC Verma-1 bit(a) , why is the acceleration of block B is half the acceleration of block A? And why is it that distance travelled by block B is half the distance travelled by block A?
question image
Asked by sakethrockzz007 | 04 Aug, 2023, 11:17: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
A small spherical object having density 2P is placed inside the liquid of density P and connected to an ideal string of length l whose other end is kept fixed so that the object can move in a vertical cirlcle, minimum speed which must be given at the bottom to just complete the vertical circle.
Asked by dhruvund8769 | 04 Feb, 2023, 11:41: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
For a liquid drop of radius r falling in air with terminal velocity power delivered by gravitational force is proportional to
Asked by gdeadshot85 | 12 Jan, 2023, 10:28: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
when we push the car,workdineyis 0,how
Asked by vaniimtiyaz630 | 12 May, 2022, 12:19: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
Two identical balls moving in opposite directions with speeds 20m/s and 25m/s undergo head on perfectly inelastic collision. The speed of combined mass after collision is 1. 20m/s 2. 22.5m/s 3. 25m/s 4. 2.5m/s
Asked by KRISHPATEL.soc | 22 Apr, 2022, 04:19: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
explain about conservation of energy with problem
Asked by bandarirohinipriya | 08 Feb, 2022, 09:46: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
Derive expression for final velocity after perfectly inelastic collision  
Asked by unnatibhise08 | 22 Dec, 2021, 08:46: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt
Get Latest Study Material for Academic year 24-25 Click here
×