CBSE Class 10 Answered

Dear Abhijeet,

The Biot-Savart law allows us to calculate magnetic field due to steady current through a small element of wire. Since direction of magnetic field due to different current elements of an extended wire carrying current is not unique, we need to add individual magnetic vectors to obtain resultant or net magnetic field at a point. This method of determining the net magnetic field follows superposition principle, which says that magnetic fields due to individual small current element are independent of each other and that the net magnetic field at a point is obtained by vector sum of individual magnetic field vectors :

B = Σ B_i = B₁ + B₂ + B₃ + ......

We calculate magnetic field due to individual current element (I dl) using Biot-Savart law :

dB = μ_o/4π (integration of dl X r)/r³

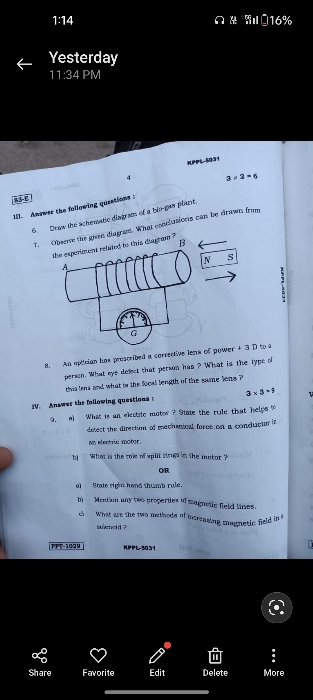
where "dl" is referred as “current length element” and "I dl"  as “current element”.

In the case of a straight wire, the task of vector addition is simplified to a great extent because direction of magnetic field at a point due to all current elements comprising the straight wire is same.

Hope this helps.

Topperlearning.com