CBSE Class 12-science Answered
Regarding Relations
Asked by thebluebloo | 31 Mar, 2009, 06:51: AM
Let a, b c belong to Z
a is related to a , as a-a=0 is divisible by m(reflexive)
if a is related to b then b must be related to a as a is related to b implies a-b is divisible by m.So b-a must also be divisible by m
since
a-b=mk for some k in Z
then b-a=m(-k)
a-b=rm where r=-k belongs to Z
so ais related to b implies b is related to a((symmetric)
if a is related to b and b is related to c
then
a-b= mk(say, for some k in Z)
and
b-c= mp(say, for some p in Z)
So a-c=(a-b)+(b-c)=mk+mp=m(k+p) is divisible by m
thus a-c is divisible by m
i.e. a is congruent to c modulo m
i.e. a is related to c (transitive)
hence the answer.
Answered by | 31 Mar, 2009, 08:53: AM
Concept Videos
CBSE 12-science - Maths
Asked by sdmbotch1123 | 18 Jan, 2023, 11:01: PM
CBSE 12-science - Maths
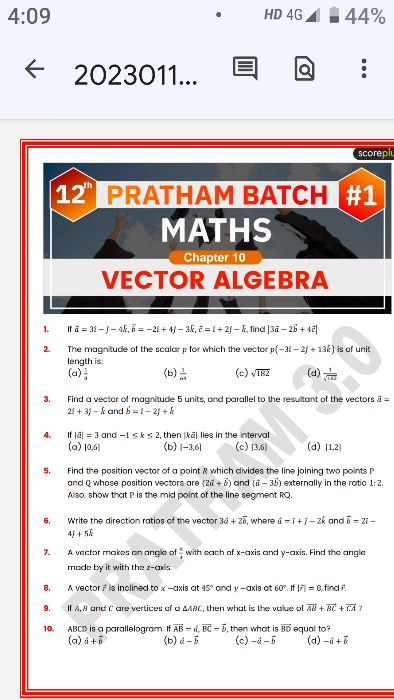
If |𝑎⃗| = 3 and −1 ≤ 𝑘 ≤ 2, then |𝑘𝑎⃗| lies in the interval
(a) [0,6] (b) [−3,6] (c) [3,6] (d) [1,2]

Asked by mohdtayyab682 | 18 Jan, 2023, 10:34: PM
CBSE 12-science - Maths
Asked by durgaprasaddurgaprasad174 | 01 Jul, 2022, 05:05: PM
CBSE 12-science - Maths
Asked by sujaychandramouli | 24 May, 2022, 10:46: AM
CBSE 12-science - Maths
Asked by abhimeena1011 | 18 Jan, 2022, 10:43: AM
CBSE 12-science - Maths
Asked by gurupadam3101985 | 11 Apr, 2020, 10:06: AM
CBSE 12-science - Maths
Asked by lovemaan5500 | 07 Feb, 2020, 09:49: AM
CBSE 12-science - Maths
Asked by lovemaan5500 | 07 Feb, 2020, 09:49: AM
CBSE 12-science - Maths
Asked by lovemaan5500 | 16 Dec, 2019, 09:34: PM
CBSE 12-science - Maths
Asked by Vivek.d8765 | 29 Oct, 2019, 10:14: PM