CBSE Class 9 Answered
Prove that the tangent at any point of a circle is perpendicular to thr radius through the point of contact.
Asked by anusarika mohanty | 21 Dec, 2013, 07:21: PM
Given: A circle C (O, r) and a tangent AB at a point P.
To Prove: OP is perpendicular to AB.
Construction: Take any point Q, other than P, on the tangent AB. Join OQ.
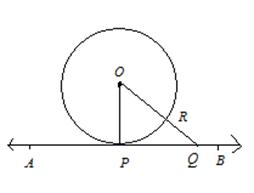
Since, Q is a point on the tangent AB, other than the point of contact P, so Q will be outside the circle.
Let OQ intersect the circle at R.
Then, OQ=OR+RQ
![]() OQ>OR
OQ>OR
![]() OQ>OP (OR=OP=radius)
OQ>OP (OR=OP=radius)
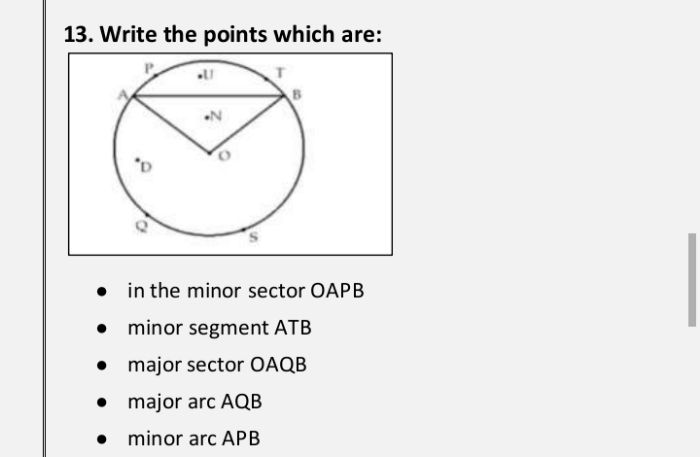
Thus, OP<OQ, i.e., OP is shorter than any other segment joining O to any point of AB.
But, among all the line segments, joining the point O to a point on AB, the shortest one is the perpendicular from O on AB.
Hence, OP is perpendicular to AB.
Answered by | 21 Dec, 2013, 09:27: PM
Application Videos
Concept Videos
CBSE 9 - Maths
Asked by indu.suresh1220 | 27 Dec, 2023, 11:12: PM
CBSE 9 - Maths
Asked by subbusuchi1978 | 05 Nov, 2023, 10:24: AM
CBSE 9 - Maths
Asked by rameshsonkar1234ramesh | 21 Dec, 2022, 11:03: PM
CBSE 9 - Maths
Asked by sdmbotch1123 | 17 Dec, 2022, 09:39: PM
CBSE 9 - Maths
Asked by geetaradha8835 | 16 Dec, 2022, 07:59: PM
CBSE 9 - Maths
Asked by chalkekalyani80 | 06 Mar, 2022, 11:25: AM
CBSE 9 - Maths
Asked by sangeetabharti123456789 | 22 Feb, 2022, 09:01: AM
CBSE 9 - Maths
Asked by manognareddy.p28 | 25 Jul, 2021, 12:52: PM
CBSE 9 - Maths
Asked by Samidhaindulkar133 | 11 Mar, 2021, 04:03: PM