CBSE Class 12-science Answered
Prove of some property of square matrix
Asked by | 14 Mar, 2009, 06:17: PM
For square matices A and B
AB = BA and ABn=BnA
Let P(n): (AB)n=A nBn
For n=1,
L.H.S = AB and R.H.S =AB
 P(n) is true for 1.
P(n) is true for 1.
Let P(n) be true for n = k.
(AB)k = AkBk
Multiply both the side by AB
L.H.S = (AB)k(AB) =(AB)k+1
R.H.S = AkBk (AB) = AkBk (BA) [ AB = BA]
AB = BA]
= Ak (Bk B) A
= Ak Bk+1 A [ABn=BnA ]
= Ak (A Bk+1)
= Ak+1 Bk+1
⇒ P(n) is true for n = k+1
By principle of mathematical induction
P(n) is true for n N.
N.
Hence, (AB)n = AnBn
Answered by | 20 Mar, 2009, 04:05: PM
Concept Videos
CBSE 12-science - Maths
Asked by sdmbotch1123 | 18 Jan, 2023, 11:01: PM
CBSE 12-science - Maths
If |𝑎⃗| = 3 and −1 ≤ 𝑘 ≤ 2, then |𝑘𝑎⃗| lies in the interval
(a) [0,6] (b) [−3,6] (c) [3,6] (d) [1,2]
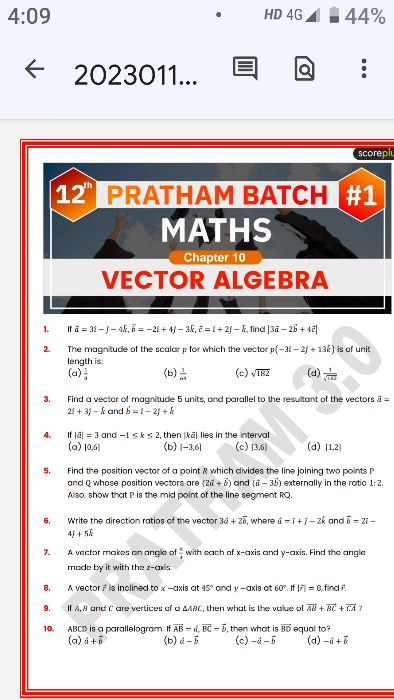

Asked by mohdtayyab682 | 18 Jan, 2023, 10:34: PM
CBSE 12-science - Maths
Asked by durgaprasaddurgaprasad174 | 01 Jul, 2022, 05:05: PM
CBSE 12-science - Maths
Asked by sujaychandramouli | 24 May, 2022, 10:46: AM
CBSE 12-science - Maths
Asked by abhimeena1011 | 18 Jan, 2022, 10:43: AM
CBSE 12-science - Maths
Asked by gurupadam3101985 | 11 Apr, 2020, 10:06: AM
CBSE 12-science - Maths
Asked by lovemaan5500 | 07 Feb, 2020, 09:49: AM
CBSE 12-science - Maths
Asked by lovemaan5500 | 07 Feb, 2020, 09:49: AM
CBSE 12-science - Maths
Asked by lovemaan5500 | 16 Dec, 2019, 09:34: PM
CBSE 12-science - Maths
Asked by Vivek.d8765 | 29 Oct, 2019, 10:14: PM