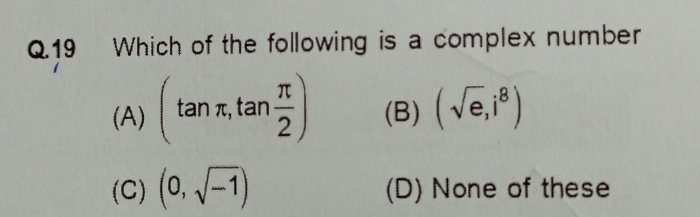CBSE Class 11-science Answered
product of real roots of the equation (given below)is:
a.always +ve
b.always -ve
c.does not exist.
d.none of these.
Asked by abhilipsa satpathy | 16 Jun, 2013, 03:12: PM
Product of roots of a given quadratic equation of the form ax^2 + bx + c = 0 is equal to c/a
Also, here we are talking of the real roots of the equation only.
So, D = b^2-4ac > 0 for real roots
D = b^2 - 4*1*9 = b^2 - 36.
Now, b = 1 for x>=0 and b = -1 for x<0. Hence b^2 in both cases would be 1
D = 1-36 = -35
So, the given equation will not have real roots in any case.
hence, the product of real roots does not exist and hence option c is correct.
Answered by | 16 Jun, 2013, 09:09: PM
Application Videos
Concept Videos
CBSE 11-science - Maths
Asked by tahikpreet0001 | 17 Apr, 2024, 06:41: AM
CBSE 11-science - Maths
Asked by nitinkrjsr07082008 | 15 Apr, 2024, 11:32: PM
CBSE 11-science - Maths
Asked by khairulmasud380 | 08 Feb, 2024, 12:22: AM
CBSE 11-science - Maths
Asked by moulya123 | 08 Jan, 2024, 06:18: AM
CBSE 11-science - Maths
Asked by saanvichawla2007 | 02 Oct, 2023, 12:10: AM
CBSE 11-science - Maths
Asked by Bhaveshkr211 | 30 Jul, 2023, 05:21: PM
CBSE 11-science - Maths
Asked by sdmbotch1123 | 18 Jan, 2023, 11:01: PM
CBSE 11-science - Maths
Asked by simrangurdasani05 | 17 Jul, 2022, 03:39: AM
CBSE 11-science - Maths
Asked by rajshekhara348 | 20 Jun, 2022, 11:23: PM