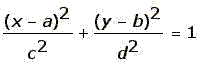CBSE Class 11-science Answered
Expert Answer
All the different types of graphs are conic sections. Since all these can be derived from a cone.
All these have equations in the form of x2 and y2.
Standard Equation of Circle: (x - a)2 + (y - b)2 = r2, where the centre is at (a,b) and radius r.
Standard Equation of Ellipse:
where (a,b) is the centre, c is the major axis (the distance from centre to graph along the horizontal) and d is the minor axis (the distance from centre to graph along the vertical). As you can see, the ellipse is very similar to the circle. Actually, the circle is a special case of the ellipse, when c = d = 1. A parabola may also be characterized as a conic section with an eccentricity of 1. Standard equation of Parabola (y - k)2 = 4a(x - h)
where h and k are the x- and y-coordinates of the vertex of the parabola and a is a non zero real number (in this investigation we consider only cases with positive a).
Application Videos
-
Relation between roots and coefficient of a Quadratic E ...
Video contains the question based on quadratic equation where the relation ... -
Sum of infinite terms of an Arithmetic-Geometric Progre ...
Video contains the question based on how to find the sum of infinite terms ... -
Introduction to Locus of a point and its equation ...
Video contains the question based on finding equation of locus of a point -
Problems based on Equation of Locus of a Point ...
Video contains the question based on finding equation of locus of a poin...
-
Introduction to Translation of Axes and Problems based ...
Video contains the question based on translation of axes
Concept Videos
-
Trigonometric identities and problems
Fundamental trigonometric identities and problems based on them. Sign Conve... -
Degree measure and Radian measure ...
This video explains the concept of negative & positive angles, conversion o... -
Types of sets and its representation
Types of sets and describe two forms of set representation. -
Subsets and Supersets
This video covers the definition of subsets and supersets of a set and also... -
Representing the union and intersection of sets
Venn diagrams. Represent the union and intersection of sets with the help o...
ANSWERED BY EXPERT ANSWERED BY EXPERT ANSWERED BY EXPERT
ANSWERED BY EXPERT ANSWERED BY EXPERT ANSWERED BY EXPERT ANSWERED BY EXPERT
ANSWERED BY EXPERT
