CBSE Class 12-science Answered
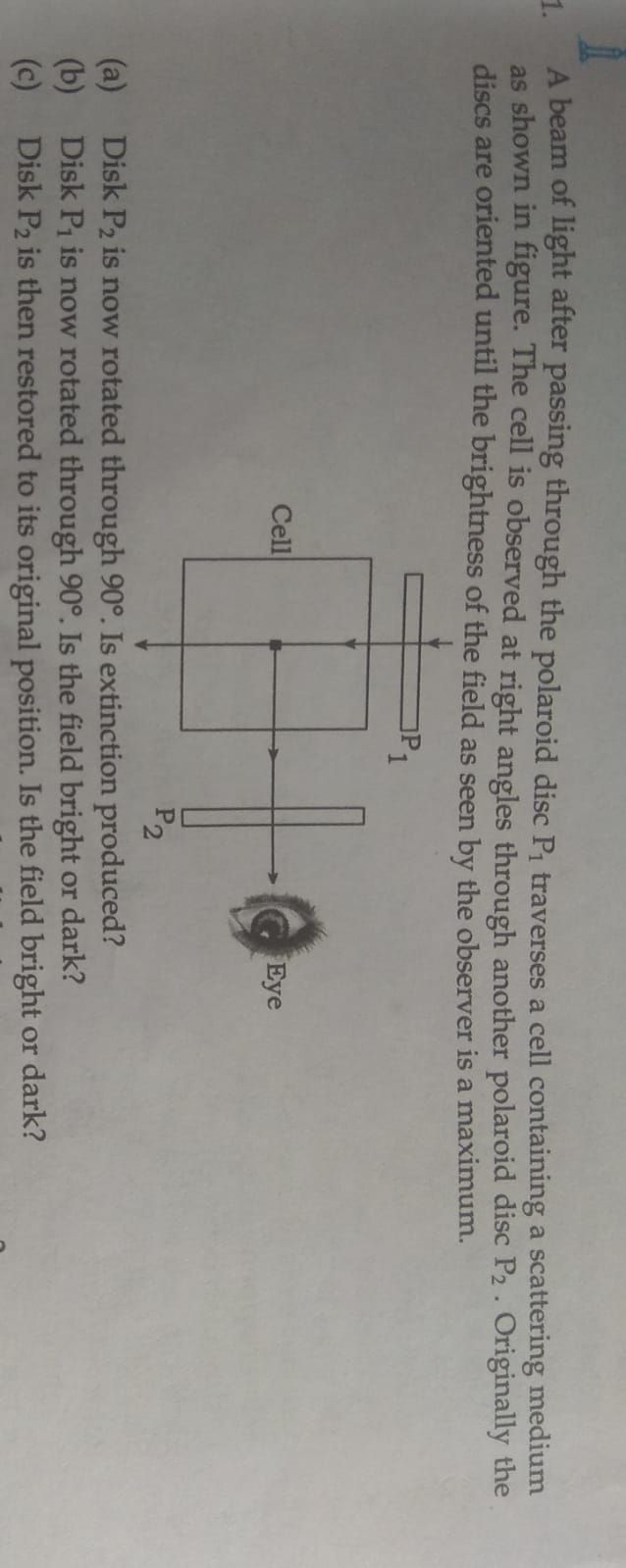
please exlain hygen's principal and law of reflection of wave theory
Asked by Jayesh Singh Gandhi | 06 Sep, 2012, 11:03: AM
WAVE FRONT:From electromagnetic theory, we know that oscillating charges emit electromagnetic waves. For a tiny oscillating dipole, this wave travels out as a spherical disturbance in all directions, with the phase (and so amplitude of the electric and magnetic fields) being constant along this wavefront. This disturbance travels with speed , with n being the refractive index of the medium in which the wave is traveling.
When we have several sources of these waves, we know that the resultant fields at any point are given by the principle of superposition.
Huygens principle is nothing but an effective principle arising from the principle of superposition. If for example, a line source is emitting light, each point along the line (which can be thought of as an atomic oscillating dipole) will emit spherical waves. However, the total wavefront, obtained by the principle of superposition, will be an envelope of all these spherical wavefronts, and will be cylindrical, from cylindrical symmetry. However, far away from sources, the radius of curvature of this wavefront becomes large, reducing its curvature locally.
So, locally the wavefront with a large radius starts appearing as a plane, and the resulting wavefront is called a plane wave wavefront.
According to Huygens principle, each point on a wavefront acts as a source of secondary spherical wavefronts (spherical, because of the point nature), these traveling as before with the speed of light in the medium. The direction of propagation is perpendicular to the wavefront, and called a ray.
This again is a result familiar from electromagnetic theory, where we saw that the fields are perpendicular to the direction of propagation, and are constant (constant amplitude) along the wavefront, which is just the definition of a wavefront. According to Huygens principle, given the forward wavefront (which defines the distance up to which the disturbance has reached at any instant) at some time, we can determine the new forward wavefront at a later time by:
(1) Regarding each point on the wavefront as a source of secondary wavelets, with directions of propagation being given by the rays, all perpendicular to this wavefront, and traveling with the speed of light
(2) Finding the surface simultaneously tangent to all these wavefronts. This forms the envelope of these secondary wavelets, giving the new wavefront. The situation can be illustrated as follows.
Concept Videos
CBSE 12-science - Physics
Asked by shivamtkr7767 | 03 Mar, 2024, 12:45: PM
CBSE 12-science - Physics
Asked by tannusharan31 | 05 Nov, 2023, 05:31: PM
CBSE 12-science - Physics
Asked by prachis14 | 06 Mar, 2023, 04:49: PM
CBSE 12-science - Physics
Asked by rajawatmeena845 | 19 Jan, 2023, 07:21: PM
CBSE 12-science - Physics
Asked by rajawatmeena845 | 19 Jan, 2023, 07:21: PM
CBSE 12-science - Physics
Asked by mrwballari999 | 03 Jan, 2023, 09:33: AM
CBSE 12-science - Physics
Asked by balwansungh111334 | 13 Jul, 2022, 07:34: AM
CBSE 12-science - Physics
Asked by suman.sh01 | 21 May, 2022, 11:09: AM
CBSE 12-science - Physics
Asked by neetaspirantdoctor | 20 May, 2022, 03:57: PM
CBSE 12-science - Physics
Asked by arjunsah797 | 15 May, 2022, 01:18: PM