CBSE Class 9 Answered
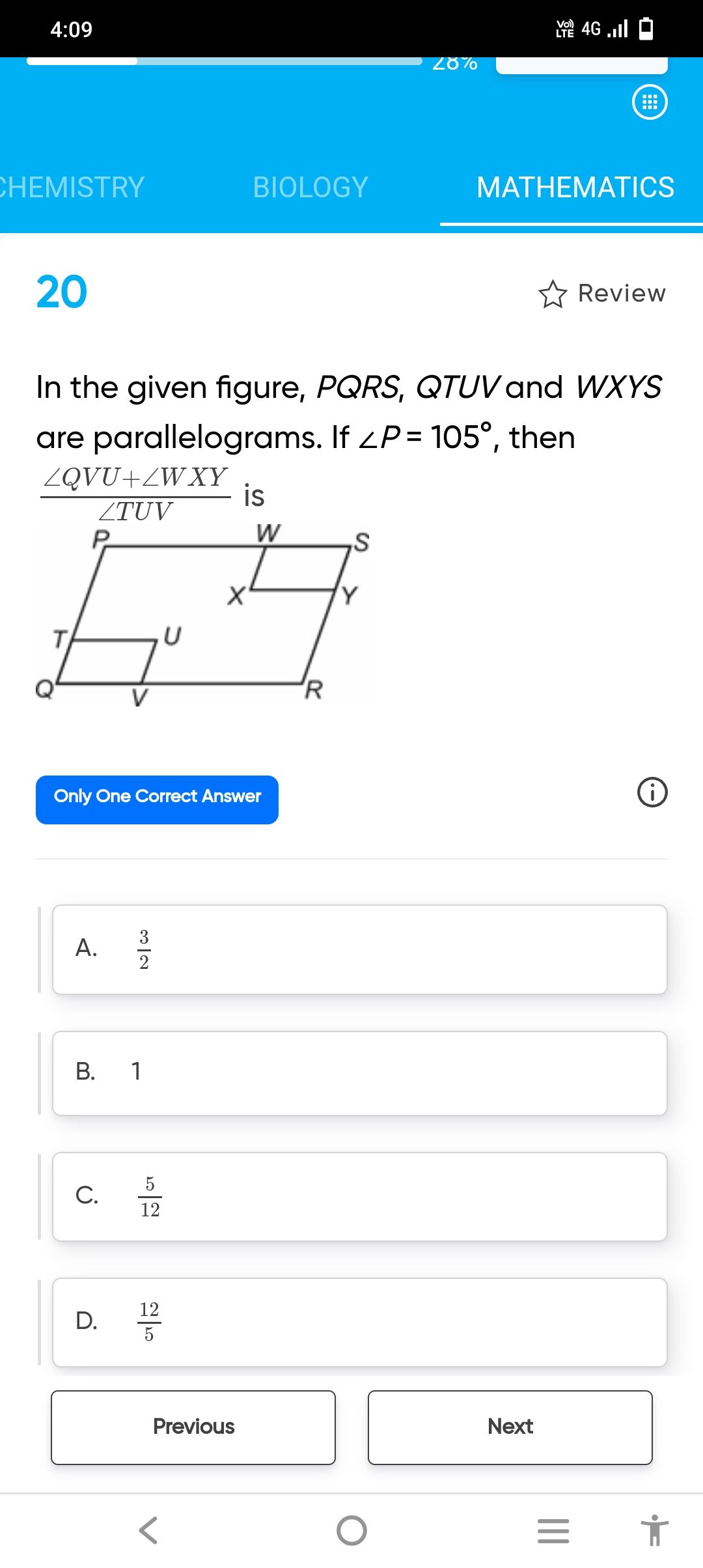
OPQR is a rhombus, whose vertices P,Q,R lie on a circle with centre O . If radius of the circle is 12 cm. Find the area of the rhombus
Asked by Jai Khurana | 29 Jun, 2013, 12:32: PM
Draw a circle with center O. And then, draw a rhombus OPQR inside the circle such that its one vertice lies on the center of the circle and the other 3 lie on the circumference of the circle.
The diagonal OQ would split the triangle in 2 congruent triangles. Moreover, we can prove that triangles OPQ and ORQ are equilateral (with each side equal to radius).
We know that one diagonal of the rhombus = OQ = r
The length of other diagonal = 2 * altitude of the equilateral triangle ORQ = root 3 * r
Now area of rhombus with diagonals d1 and d2 = 1/2. d1 * d2
Hence, (1/2). r . root 3 * r = Area
Since r = 12cm
Hence, area = root(3)/2 *12*12 = 72 root(3) cm^2
Answered by | 29 Jun, 2013, 05:22: PM
Application Videos
Concept Videos
CBSE 9 - Maths
Asked by chandininayak86 | 15 Dec, 2023, 07:36: AM
CBSE 9 - Maths
Asked by sagrawal0081 | 31 Aug, 2023, 07:24: PM
CBSE 9 - Maths
Asked by jahnavinehra | 03 Jul, 2022, 11:19: AM
CBSE 9 - Maths
Asked by seemarana8535 | 11 May, 2022, 04:17: PM
CBSE 9 - Maths
Asked by abhiroy1462 | 16 Apr, 2022, 02:25: PM
CBSE 9 - Maths
Asked by abdullahzedane2008 | 28 Mar, 2022, 12:11: PM
CBSE 9 - Maths
Asked by sanskritiraghudev | 19 Feb, 2022, 09:38: AM
CBSE 9 - Maths
Asked by varundhawan | 31 Jan, 2022, 10:41: PM
CBSE 9 - Maths
Asked by aziznoorani17 | 04 Jan, 2022, 11:32: AM
CBSE 9 - Maths
Asked by vineetyadav77050 | 25 Jul, 2021, 05:45: PM