CBSE Class 12-science Answered

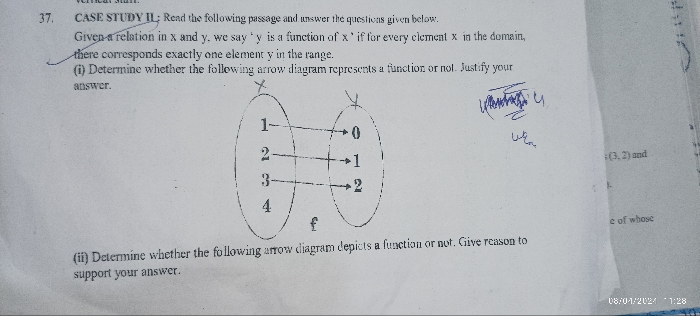
for a function to be one one

 we must have

f(a) not equal to f(b), if a not equal tob.

i.e.[a] not equal to [b] if a not equal to b

so by defn of greates int fn we get that

if a not equal to b then f(a0 not equal to f(b)

so it's not one one.

for a function to be onto

we must have some element c from codomain R such that f(a)=c where a belongs to the domain R.

but if c is not an integer then f(a) can't be c for any a as the answer of the greates integer fn has to be an integer.

so we see that every element of R the codomain does not have a preimage in the domain R.

so by definition of onto functions f is not onto.