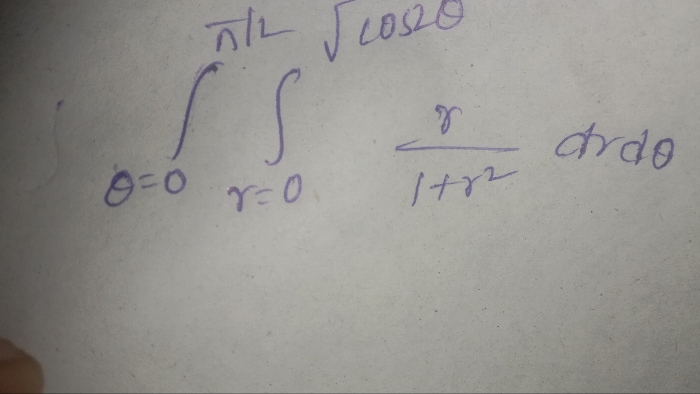CBSE Class 12-science Answered
Integrate the given ques
Asked by spsberry8 | 02 Feb, 2011, 07:49: PM
Expert Answer
Dear student,
sinx + cosx = √2.sin(x+∏/4)
So, log(sinx + cosx) = log[√2.sin(x+∏/4)]
= log√2 + logsin(x+∏/4)
We have:
So, log(sinx + cosx) = log[√2.sin(x+∏/4)]
= log√2 + logsin(x+∏/4)
We have:
I = ∫log√2 dx + ∫logsin(x+∏/4) dx
= (log√2)x + I'
In I', put x+∏/4 = t. So, dx = dt
= (log√2)x + I'
In I', put x+∏/4 = t. So, dx = dt
I' = ∫log(sint) dt
Now, the limit is 0 to ∏/2
So, I = ∏/2.log√2 + I', where the limits are from o to ∏/2 ... (A)
Now, the limit is 0 to ∏/2
So, I = ∏/2.log√2 + I', where the limits are from o to ∏/2 ... (A)
Now, I'=∫ log(sin x).dx ...(1)
I'=∫logsin(π/2-x)dx
I'=∫logcosx dx...(2)
Add1&2,
2I'=∫logsinx+logcosx dx
=∫logsinx*cosx dx
=∫log(sin2x)/2 dx
=∫[logsin2x-log2] dx
=∫logsin2xdx - ∫log2dx ...(3)
In the first integral put 2x=t so dx=dt/2
when x=0,t=0 when x=π/2 ,t=π
So, the first integral can be solved as below:
∫logsint dt with limit t= o & t=π
Now, solve this integral and get the value of the integral I' from the equation (3). Thereafter, equate the value of I' in (A) to get the value of the required integral I.
Now, solve this integral and get the value of the integral I' from the equation (3). Thereafter, equate the value of I' in (A) to get the value of the required integral I.
We hope that clarifies your query.
Regards,
Team
TopperLearning
Answered by | 23 Mar, 2011, 09:25: PM
Concept Videos
-
Introduction to Integration ...
This video explains integration as an anti derivative reverse of differenti... -
Integration by substitution
This video explains the use of section formula to find mid point of a line ... -
Integration by partial fractions
This video covers examples related to Integration by partial fractions, whe... -
Integrals using Trigonometic Identities
This video covers examples related to the use of trigonometric identities t... -
Standard formulae for integration of functions
Learn standard formulae for integration of functions involving quadratic fu...
CBSE 12-science - Maths
Asked by jprathamesh023 | 23 Mar, 2024, 04:01: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by atulmishra514 | 26 Jan, 2024, 10:15: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by aalavarshitha2005 | 21 Jan, 2024, 05:28: PM
ANSWERED BY EXPERT
CBSE 12-science - Maths
Asked by atharvaparkar04 | 17 Dec, 2023, 12:59: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by shashinayaka032 | 05 Dec, 2023, 12:46: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by manyarajawat1 | 06 Nov, 2023, 05:03: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by ps9680677 | 26 Oct, 2023, 11:47: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by manasvichandel49 | 19 Oct, 2023, 08:53: AM
ANSWERED BY EXPERT
