CBSE Class 11-science Answered
In a rocket, the mass of the fuel is 90℅ of the total mass. The exhaust gasses are ejected at a speed of 2km/s. Find the maximum speed attained by the rocket with diagram. Neglect gravity and air resistance and take the initial speed of the rocket to be zero. (Given, log e 10 = 2.3)
Asked by rrpapatel | 18 Oct, 2018, 04:13: PM
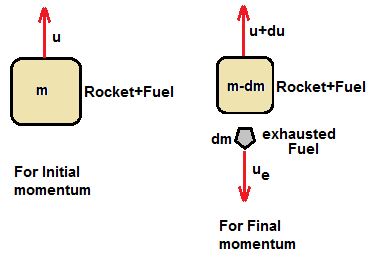
At a given instant, let m be the total mass of rocket+fuel and u be the velocity.
Once small amount dm of fuel is exhausted its velocity increases by u+du. Let ue be the exhaust velocity.
Initial momentum = m×u
final momentum = (m - dm)×(u+du) - dm ×(ue-u) = m×u + m×du - dm×ue (term dm×du is neglected)
Since gravity and air resistance are neglected, there in sno external force, hence momentum is conserved.
By momentum conservation, we have, m×u + m×du - dm×ue = m×u ..............(1)
after simplification of eqn.(1), we get, du = ue × (dm/m) ..............(2)
By integrating eqn.(2), we get

It is given that initial velocity ui = 0. Since 90% of initial mass is fuel and final mass is only rocket weight, mi = 10×mf
Then we have from eqn.(3) final maximum velocity uf = ue×ln(0.1) = -2×(-ln10) = 2 × 2.3 = 4.6 km/s
Answered by Thiyagarajan K | 19 Oct, 2018, 02:46: AM
CBSE 11-science - Physics
Asked by sheikhsaadat24 | 17 Apr, 2024, 09:41: PM
CBSE 11-science - Physics
Asked by sy123946 | 07 Apr, 2024, 04:23: PM
CBSE 11-science - Physics
Asked by derhebha955 | 03 Apr, 2024, 09:03: AM
CBSE 11-science - Physics
Asked by sumedhasingh238 | 29 Mar, 2024, 05:15: PM
CBSE 11-science - Physics
Asked by sumedhasingh238 | 28 Mar, 2024, 11:10: PM
CBSE 11-science - Physics
Asked by roshnibudhrani88 | 23 Mar, 2024, 05:52: PM
CBSE 11-science - Physics
Asked by emad.amd | 21 Mar, 2024, 12:00: PM
CBSE 11-science - Physics
Asked by vinitdubey7735 | 14 Mar, 2024, 11:21: AM
CBSE 11-science - Physics
Asked by om636694 | 04 Mar, 2024, 09:10: PM
CBSE 11-science - Physics
Asked by rajuinwati12 | 04 Mar, 2024, 09:22: AM




