CBSE Class 9 Answered
(i)Side BC of is produced to a point D.The bisector of
is produced to a point D.The bisector of  meets BC at L.Prove
meets BC at L.Prove  .
.
 is produced to a point D.The bisector of
is produced to a point D.The bisector of  meets BC at L.Prove
meets BC at L.Prove  .
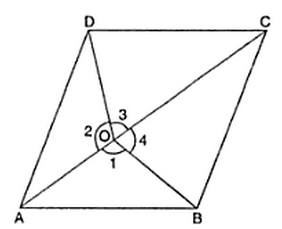
.(ii)A point O is taken inside an equilateral four sided figure ABCD such that its distances from the angular points D & B are equal.Show that AO & OC are in one & the same straight line.
Asked by araima2001 | 13 Sep, 2014, 11:00: AM
In given equilateral four sided figure ABCD
AD = AB ( equilateral quadrilateral)
equilateral quadrilateral)
AO = AO ( common)
common)
OD = OB ( Given)
Given)
Therefore ,  AOD =
AOD =  AOB ……………..(1)
AOB ……………..(1)
Similarly ,  CDO =
CDO =  BOC ……………...(2)
BOC ……………...(2)
But,  AOD +
AOD +  AOB +
AOB +  CDO +
CDO +  BOC = 360
BOC = 360
2  AOB + 2
AOB + 2  BOC = 360 (From eqn (1) and (2) )
BOC = 360 (From eqn (1) and (2) )
2 ( AOB +
AOB +  BOC )= 360
BOC )= 360
 AOB +
AOB +  BOC = 180
BOC = 180
So, AO and OC are in same straight line
Hence proved
Answered by Dharma Teja | 14 Sep, 2014, 11:35: PM
Application Videos
Concept Videos
CBSE 9 - Maths
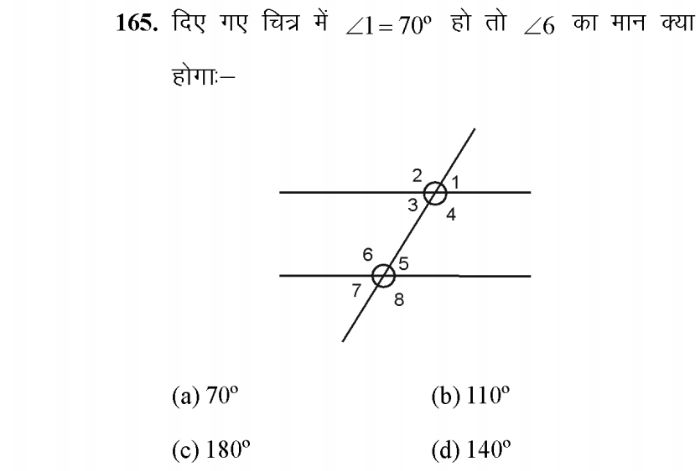
Asked by roshan.rony001 | 19 Sep, 2023, 05:02: PM
CBSE 9 - Maths
Asked by pushpadevipushpadevi19 | 13 Jan, 2023, 08:06: PM
CBSE 9 - Maths
Asked by savitamahajan202 | 02 Jan, 2023, 10:55: AM
CBSE 9 - Maths
Asked by amarjitruksh | 20 Jun, 2022, 10:40: PM
CBSE 9 - Maths
Asked by Abhishita9 | 12 Nov, 2021, 06:41: PM
CBSE 9 - Maths
Asked by raghavgargrg28 | 10 Sep, 2021, 05:11: PM
CBSE 9 - Maths
Asked by kamateganpati | 08 Sep, 2021, 06:17: PM
CBSE 9 - Maths
Asked by swatipuspapatel | 07 Feb, 2021, 07:29: PM
CBSE 9 - Maths
Asked by saritaagarwal30121980 | 14 Oct, 2020, 10:07: AM