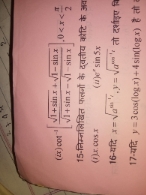CBSE Class 12-science Answered
How to proceed step by step while taking derivatives of inverse trigonometric functions?
Asked by PRAJAY RAIKAR | 15 Jun, 2013, 08:14: PM
Expert Answer
While trying to find a derivative of the inverse trignometric functions, apply the chain rule that you normally apply to the other functions also.
So, first compute the derivate of the inverse, then find the derivative of the function thats inside inverse function.
For example if f(x) = sin^-1 (x^2) and you need to find the derivate of f(x)
f'(x) = derivative of (sin^-1 (x^2)) * derivate of (x^2)
f'(x) = 1/(sqrt(1-(x^2)^2)) * 2x
f'(x) = 1/sqrt(1-x^4)* 2x
f'(x) = 2x/sqrt(1-x^4)
In response to your second question, there is no defined way of determining the use of a trignometric identity. You should remember all the trignometric identities by heart, so that whenever you will se a question which has any of the terms, you can immediately use them. Also, practice as much as you can, so that you reach a point where just by looking at the question, you can determine which identity to use.
Answered by | 15 Jun, 2013, 09:23: PM
Concept Videos
-
Continuous and Discontinuous function
Understand the meaning of function to be continuous and discontinuous, comb... -
Relationship between continuity and differentiability - ...
Relationship between continuity and differentiability, concept of composite... -
Derivative of Parametric Functions
Learn the relation between two variables x and y using the third variable a... -
Second-order derivatives
Learn to find out the second-order derivatives of the given functions. -
Rolle's Theorem and its geometrical interpretation ...
Discuss Rolle's Theorem and its geometrical interpretation. Also discuss La...
CBSE 12-science - Maths
Asked by fehasaf670 | 27 Jan, 2024, 01:39: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by tanyavishwakarma427 | 24 Nov, 2023, 11:56: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by sonkarmukesh007 | 13 Aug, 2023, 08:32: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by unqio66 | 15 May, 2023, 11:48: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by sdmbotch1123 | 21 Dec, 2022, 04:38: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by iasaspirant20s | 09 Oct, 2022, 02:31: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by Raunaksingh4742 | 25 Aug, 2022, 12:31: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by pinkidevnict | 08 Jun, 2022, 07:51: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by suvajyotichaudhury998 | 04 Jun, 2022, 07:54: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by lewisraina069 | 03 Jun, 2022, 06:56: AM
ANSWERED BY EXPERT
