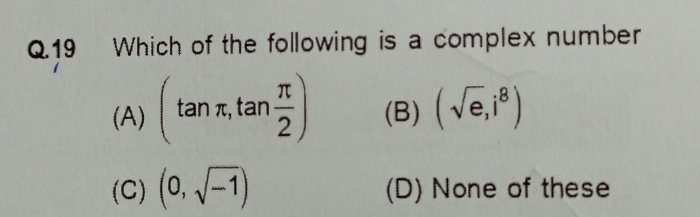Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

CBSE Class 11-science Answered

Given a quadratic equation , where a, b and c are real numbers. If 2+3i is one of the roots of the equation, what is the other root?Ans. In a quadratic equation with real coefficients, if the roots are complex then they always occur in complex conjugate pair. So, the other root is 2-3i
Asked by GARIMA SRIVASTAVA | 12 Mar, 2011, 04:40: AM
answered-by-expert Expert Answer
dear student,
pl note that the complex roots of a quadratic equation always occur in pairs, they are conjugates of each other.
so the other root is 2-3i
this happens because when you are solving the quadratic equation by using the quadratic formula there is a plus or minus sign before the radical sign right?
that radical is not real so comes out as something multiplied by i.
so once it becomes like 2+3i and when you take the negative sign the root is 2-3i
solving one question below to solve your doubt completely.
suppose the use of quad formula gives us
x=
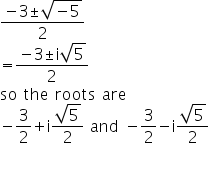
so we see that both the roots have everything else the same except the sign between the two terms of the numerator.Hence when one root is given the other can be found easily by just changing the sign as mentioned above.
hope this clarifies your doubt.
Answered by | 12 Mar, 2011, 08:25: PM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
CBSE 11-science - Maths
find square root of 7+24i
Asked by tahikpreet0001 | 17 Apr, 2024, 06:41: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
if α, ß, Γ be the roots of the equation x^3+px^2+qx+r=0 then find the value of (α+ß-3Γ)(ß+Γ-3α)(α+Γ-3ß)
Asked by nitinkrjsr07082008 | 15 Apr, 2024, 11:32: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
show me the process to solve the problem
question image
Asked by khairulmasud380 | 08 Feb, 2024, 12:22: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
express in the form of a+ib (i)(1-i)^4
Asked by moulya123 | 08 Jan, 2024, 06:18: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
question number 34
question image
Asked by saanvichawla2007 | 02 Oct, 2023, 12:10: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
For a real number r, the quadratics x² + (r - 1) x + 6 and x² + (2r + 1) x + 22 have a common real root. The sum of the possible values of r can be expressed as a/ b where a, b are relatively prime positive integers. Compute 10a + b
Asked by Bhaveshkr211 | 30 Jul, 2023, 05:21: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
Pls solve
question image
Asked by sdmbotch1123 | 18 Jan, 2023, 11:01: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
solve
question image
Asked by Imotheph1504 | 19 Dec, 2022, 08:19: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
the roots of -18i
Asked by simrangurdasani05 | 17 Jul, 2022, 03:39: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
complete solution set of the equation |x^2-3|+|2-x|=|x^2-x-1|
Asked by rajshekhara348 | 20 Jun, 2022, 11:23: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt
Get Latest Study Material for Academic year 24-25 Click here
×