Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

CBSE Class 12-science Answered

Find the range of the function f(x)= |x-1|+|x-2| where x lies in [-1,3]
Asked by Anupriya | 22 May, 2013, 11:53: PM
answered-by-expert Expert Answer
 f(x)= |x-1|+|x-2| 
f(x) = -2x+3 (x<=1) ; 1 (1<x<=2) ; 2x-3 (x>2)
 
On plotting the 3 equations in a x-y graph for specified range of x, we will find that the function is a V -shaped function, with decreasing straight line for x<1 and then constant for x between 1 and 2 and then it is an increasing function. 
 
So, for x between -1 and 3, f(x) will vary from [1,5] 
 
Hence, range of the function is [1,5] when x lies in [-1,3]
 
hence for (-1<=x<=3), 
Answered by | 23 May, 2013, 02:40: AM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
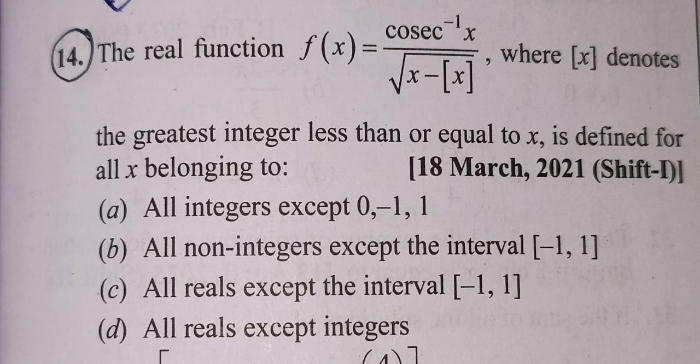
CBSE 12-science - Maths
Please Answer this case study..!!.
question image
Asked by 20muskaansharma23 | 08 Apr, 2024, 11:31: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
pls make the table of this question
question image
Asked by nainaadityathakur | 12 Mar, 2024, 01:22: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
doubt
question image
Asked by jhaprabhat920 | 05 Feb, 2024, 10:33: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
if Z is the set of all integers and R is the relation on Z defined as R ={(a, b) :a b € z and a-b is divisible by 5 } prove that x is an equivalence relation
question image
Asked by aiswaryaanil0009 | 01 Feb, 2024, 08:36: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
what is the range of f(x)=cos2x-sin2x
Asked by raunakumar9300 | 01 Feb, 2024, 04:23: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
A is b' mother. C is b's father. Relation between A and C  
Asked by amandwivesh | 25 Oct, 2023, 11:57: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
Prove that the function f:R-R,given by f(x)=2x,is one-one and onto
Asked by bornalimalakar3 | 05 Oct, 2023, 11:15: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
Let R be an equivalence relation defined in the set A = \{1, 2, 3, 4, 5, 6, 7, 8, 9\} by R=( (x, y) : x,ye A, x and y are either both odd or both even)Write all the equivalence classes of set A.
Asked by rginish12 | 04 Oct, 2023, 11:14: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
solve it.
question image
Asked by sdmbotch1123 | 19 Sep, 2023, 01:53: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
 If B is coded =4 and D is coded 8 , E is coded 10 then what will be code of J
Asked by iamujjy | 21 May, 2023, 04:55: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt
Get Latest Study Material for Academic year 24-25 Click here
×