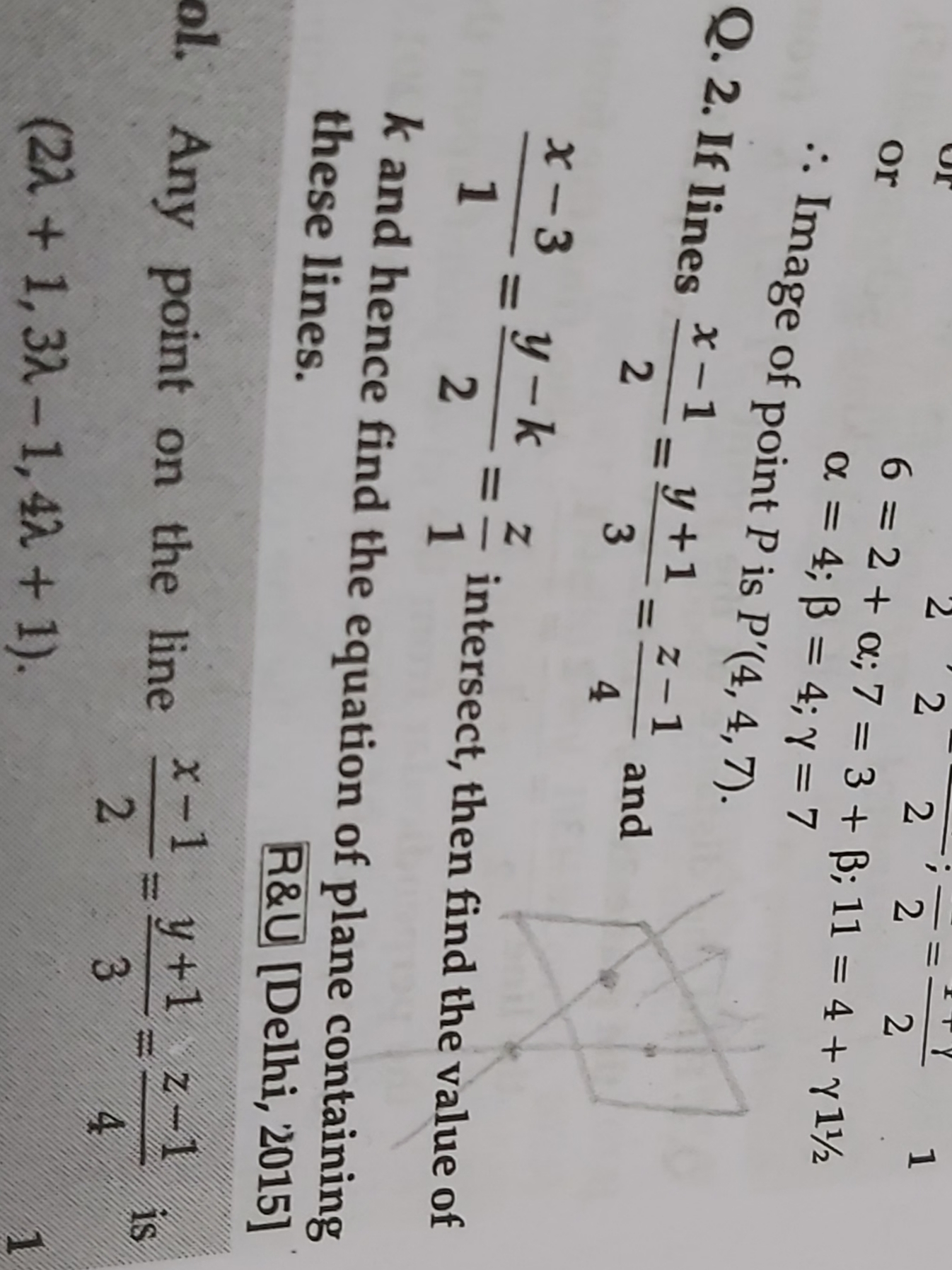CBSE Class 12-science Answered
Find the length and equations of the line of shortest distance between the lines given by:
(x-8)/3=(y+9)/(-16)=(z-10)/7 and (x-15)/3=(y-29)/8=(z-5)/(-5)
The answer given in the book is:14 units, (x-5)/2=(y-7)/3=(z-3)/6
NOTE:
Thank you very much for answering my question posted on 18 May,2013. I also solved this question, but the answer did not come to ?/2 and therefore I posted this question. Please note that there is no mistake in typing at all. A couple of questions or answers to them are wrong in every exercise relating to 3D Geometry.
Asked by Manoj | 24 May, 2013, 07:09: PM
Expert Answer
let P and Q be the points on the given lines, respectively. then the general coordinates of P and Q are:
therefore the direction ratios of PQ are 
now PQ will be the shortest distance if it is perpendicular to both the given lines, therefore by the condition of perpendicularity,
now solving (1) and (2),
equation (1) becomes: 
equation (2) becomes: 
now solving 1(a) and 1(b), we get
hence the points are
therefore the shortest distance between the lines
since we have the points P and Q, the equation of the line which passes through two given points is:
Answered by | 25 May, 2013, 10:42: PM
Concept Videos
-
Cartesian equation of a line
This video covers examples related to Equation of a line in space, Vector a... -
Skew Lines
This video explains how to calculate angle, shortest distance between two l... -
Equation of a Plane
This video explains the equation of a plane in normal form, perpendicular t... -
Intercept Form and Family of Planes
This video explains the equation of a plane through 3 non-collinear points,... -
Co-planarity of two lines
Co-planarity of two lines, angle between two planes & between a line and a ...
CBSE 12-science - Maths
Asked by shubh31122006 | 01 Feb, 2024, 09:06: AM
ANSWERED BY EXPERT
CBSE 12-science - Maths
Asked by kishanradha7392 | 05 Jul, 2022, 04:26: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by suman.sh01 | 07 Jun, 2022, 07:22: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by arjunsah797 | 02 May, 2022, 09:30: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by Madhureddymamilla | 06 Jan, 2020, 01:11: PM
ANSWERED BY EXPERT
CBSE 12-science - Maths
Asked by rsjkumar2003 | 23 Sep, 2019, 01:09: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by harshgarg18012003 | 26 Jun, 2019, 09:11: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by tv2000in | 25 May, 2019, 06:25: PM
ANSWERED BY EXPERT
