CBSE Class 12-science Answered
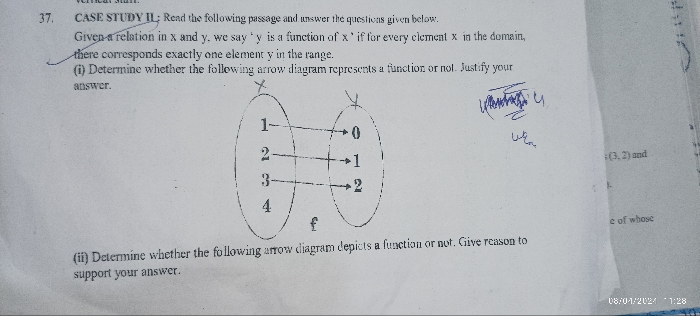
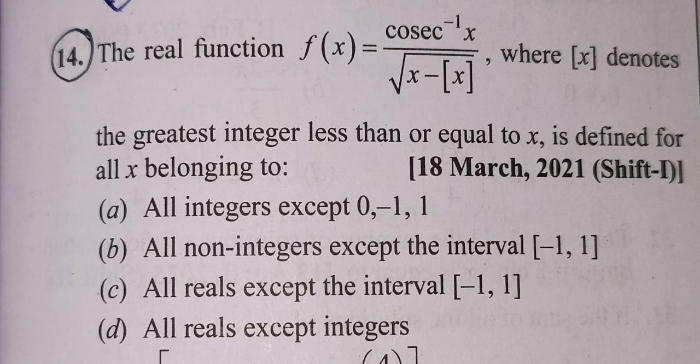
Explain how we can find number of bijective functions from set A to set B if n(A)=n(B).
Asked by abhinavsaini123 | 01 Jun, 2015, 09:00: PM
Expert Answer
If a function defined from set A to set B f:A->B is bijective, that is one-one and and onto, then n(A)=n(B)=n
So first element of set A can be related to any of the 'n' elements in set B.
Once the first is related, the second can be related to any of the remaining 'n-1' elements in set B.
If we proceed like this , the total number of ways of relating every element of set A to a unique element of set B can be found by using multiplication principle of counting,
n(n-1)(n-2)(n-3)....2X1= n!
Answered by satyajit samal | 03 Jun, 2015, 09:10: AM
Application Videos
-
ProblemsbasedonIntoFunction-I
Video contains the question based on verification of a function to be Into ... -
ProblemsbasedonIntoFunction-II
Video contains the question based on verification of a function to be Into ...
Concept Videos
-
Introduction to Relations
This video explains the concept of relations and types of relation like Emp... -
Functions and its type
Learn the definition and way of exhibiting functions. Domain, co-domain and... -
Problems on Functions
This video revists the types of functions and solve problems based on them. -
Concepts related to composition of functions
Define composition of functions and establish the condition for a function... -
Problems on Composition
This video covers the concept of composition of functions and invertible fu...
CBSE 12-science - Maths
Asked by 20muskaansharma23 | 08 Apr, 2024, 11:31: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by nainaadityathakur | 12 Mar, 2024, 01:22: PM
ANSWERED BY EXPERT
CBSE 12-science - Maths
Asked by aiswaryaanil0009 | 01 Feb, 2024, 08:36: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by raunakumar9300 | 01 Feb, 2024, 04:23: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by amandwivesh | 25 Oct, 2023, 11:57: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by bornalimalakar3 | 05 Oct, 2023, 11:15: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by rginish12 | 04 Oct, 2023, 11:14: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by sdmbotch1123 | 19 Sep, 2023, 01:53: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by iamujjy | 21 May, 2023, 04:55: PM
ANSWERED BY EXPERT
