CBSE Class 6 Answered
Draw a circle of any radius and a chord AB in it. Construct a perpendicular from centre to the chord. Does this perpendicular divide the chord in equal parts?
Asked by Topperlearning User | 11 Dec, 2013, 09:47: AM
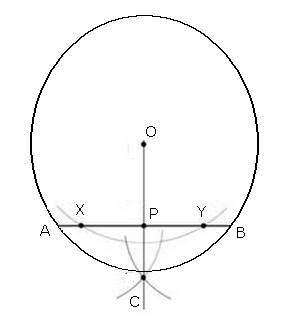
1. Draw a circle with centre O and any radius and make a chord AB.
2. Taking O as a centre and a suitable radius, draw an arc which cut the chord AB at two points X and Y.
3. Taking X and Y as centre and same radius draw two arcs and let them intersect at C.
4. Join OC; name the point of intersection of AB and OC as P.
Measure AP and PB, we find that AP = PB.
Hence, the perpendicular from centre divides the chord in two equal parts.
Answered by | 11 Dec, 2013, 11:47: AM
Concept Videos
CBSE 6 - Maths
Draw a line segmeat xy equal to 8 cm. Using compasses and ruler,construct its perpendicular bisector
Asked by sharmakanishk153 | 13 Dec, 2019, 09:26: PM
CBSE 6 - Maths
Asked by Topperlearning User | 04 Jun, 2014, 01:23: PM
CBSE 6 - Maths
Asked by Topperlearning User | 04 Jun, 2014, 01:23: PM
CBSE 6 - Maths
Asked by Topperlearning User | 25 Nov, 2013, 05:03: AM
CBSE 6 - Maths
Draw a line segment AB of length 10 cm. Divide it 4 equal parts and measure the length of each part.
Asked by Topperlearning User | 11 Dec, 2013, 02:50: AM
CBSE 6 - Maths
Asked by Topperlearning User | 11 Dec, 2013, 01:02: AM
CBSE 6 - Maths
Asked by Topperlearning User | 04 Jun, 2014, 01:23: PM
CBSE 6 - Maths
Asked by Topperlearning User | 11 Dec, 2013, 09:47: AM
CBSE 6 - Maths
Asked by Topperlearning User | 04 Jun, 2014, 01:23: PM
CBSE 6 - Maths
Asked by Topperlearning User | 04 Jun, 2014, 01:23: PM