Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

CBSE Class 11-science Answered

derive the formula for motion of a single pendulum
Asked by shivam gupta | 12 May, 2012, 08:35: AM
answered-by-expert Expert Answer

Rotational Method




pendulum variables
The pendulum is modeled as a point mass at the end of a massless rod. We define the following variables:
  • ? = angle of pendulum (0=vertical)
  • R = length of rod
  • T = tension in rod
  • m = mass of pendulum
  • g = gravitational constant
We will derive the equation of motion for the pendulum using the rotational analog of Newton's second law for motion about a fixed axis, which is ? = I ? where
  • ? = net torque
  • I = rotational inertia
  • ? = ?''= angular acceleration
The rotational inertia about the pivot is I = m R2. Torque can be calculated as the vector cross product of the position vector and the force. The magnitude of the torque due to gravity works out to be ? = ?R m g sin ?. So we have?R m g sin ? = m R2 ?which simplifies to
?'' = ? g?R sin ? (1)
This is the equation of motion for the pendulum.
Answered by | 13 May, 2012, 08:04: PM

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
CBSE 11-science - Physics
the dimension of stopping potential vo in photoelectric effect in units of plank's constant 'h',speed of light 'c' and gravitational constant 'G' and ampereA
Asked by derhebha955 | 03 Apr, 2024, 09:03: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
In this que while solving it we take D F of alpha =D.F of beta.Why is it so?
question image
Asked by sumedhasingh238 | 29 Mar, 2024, 05:15: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
In physics if 2 quantities are dimensionless then their dimensional formula are equal?
Asked by sumedhasingh238 | 28 Mar, 2024, 11:10: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
Power unit
Asked by rosysahu678 | 02 Mar, 2024, 06:09: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
frequency of vibration (n) of a stretched string depends on its length (l) , tension (t) and linear mass density taking proportionality constant k=½ find a relation between them
Asked by ss4172380 | 14 Nov, 2023, 08:05: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
Calculate the wave number of a wave with a frequency of 6.2 *10^7
Asked by doddisriman | 29 Oct, 2023, 09:43: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
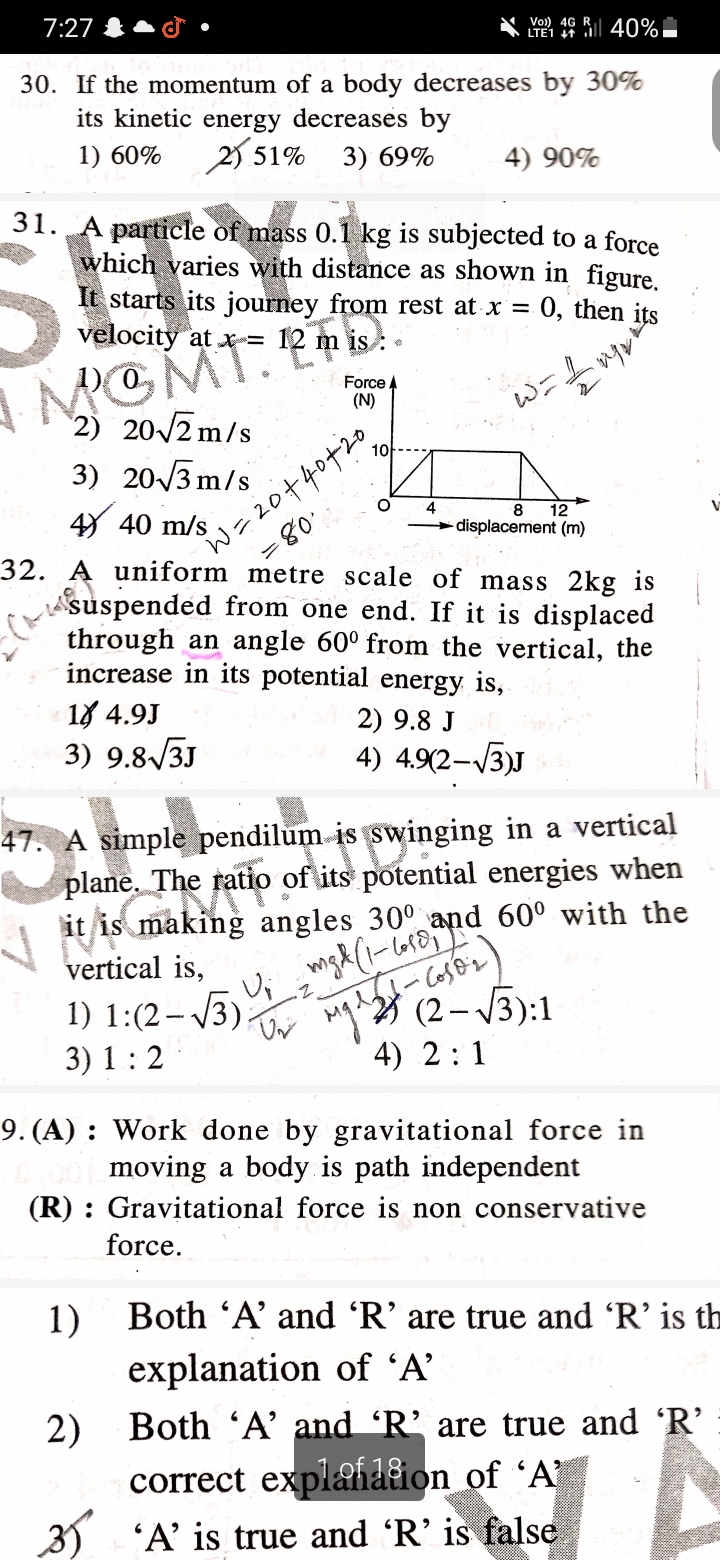
CBSE 11-science - Physics
name to fundamental forces in nature
question image
Asked by sanketnaik338 | 04 Oct, 2023, 09:13: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
Calculate the angle of (a) , 1°(b) 1`(minute of arc or arcmin) and (c) 1``(second of arc second) in radius. Use 360°=2π rad, 1°=60` and 1`=60``.
Asked by mayankvaram96 | 01 Oct, 2023, 06:01: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
The respective number of significant figures for the numbers 23.023; 0.0003 and 2.1´10-3 are (a) 4, 4, 2                     (b) 5, 1, 2                     (c) 5, 1, 5                     (d) 5, 5, 2
Asked by Naveensharma2007 | 26 Jun, 2023, 06:54: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Physics
if the momentum of body decreases by 30% its kinetic energy decreased by
question image
Asked by bhargavchary19 | 23 Dec, 2022, 07:33: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt
Get Latest Study Material for Academic year 24-25 Click here
×