CBSE Class 10 Answered
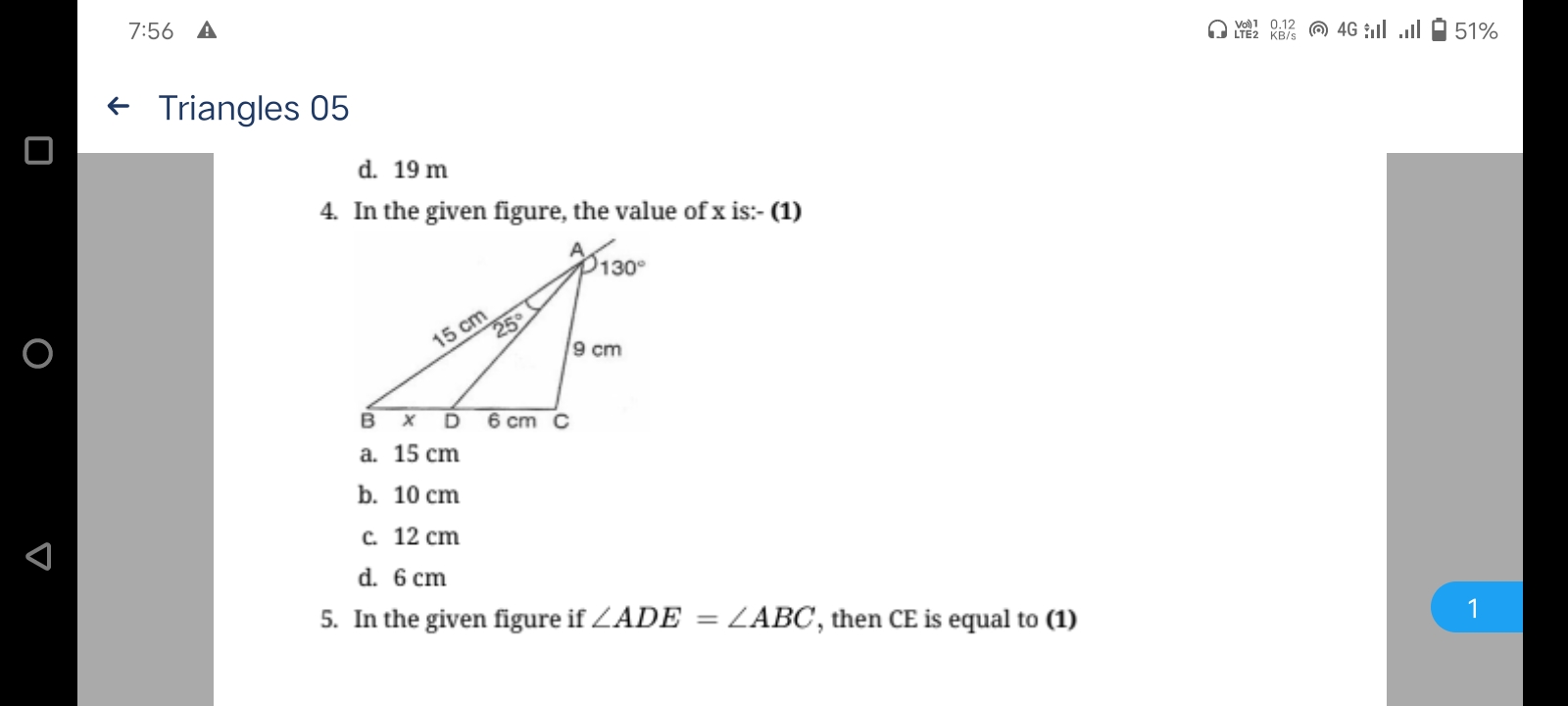
D,E and F are respectively the midpoints of sides AB,BCand CA of triangle ABC. find the ratios of the areas of DEF and ABC
Asked by chandershekhar sharma | 19 May, 2013, 09:39: PM
Expert Answer
Given - A triangle ABC with D, E and F being the mid points of AB, BC and CA respectively.
Join DE, EF and FD.
Using mid point theorem, a line joining the mid points of 2 sides in a triangle is parallel to the third side and equal to half the length of third side.
Hence, DE = 1/2 AC, EF = 1/2AB and FD = 1/2BC
Hence, by SSS similarity,
triangle DEF is similar to triangle CAB
Now, the ratio of areas of similar triangles is equal to the square of the ratios of their sides
Hence, triangle(DEF)/triangle (CAB) = DE^2/CA^2 = 1/4
Answered by | 20 May, 2013, 09:26: AM
Application Videos
-
Application of Basic proportionality theorem ...
This video explains the concept of an isosceles triangle and Basic Proporti... -
Area of a right angled triangle
This video explains how to compute the area of a right angled triangle. -
Word Problem on Pythagoras Theorem
This video explains the concept of Pythagoras Theorem and the area of the r... -
Area of Similar Triangles
The video explains the relation between areas and sides of similar trian...
-
Word Problem on An Equilateral Triangle
The video explains a proof based on equilateral triangle.
Concept Videos
-
Converse of BP theorem
Statement and derivation of the converse of the Basic Proportionality Theor... -
Criteria for similarity of triangles
This video explains the basic criteria for similarity of triangles and unde... -
Revision on concepts related to similar triangles ...
Review concepts related to Similar Triangles and practice problems based on... -
Basic Proportionality Theorem ...
This video explains the statement and derivation of the Basic Proportionali... -
Areas of similar triangles
This video explains the relation between the area of similar triangles and ...
CBSE 10 - Maths
Asked by suryapalsinghgoudmahirajbanna | 17 Sep, 2023, 10:50: PM
ANSWERED BY EXPERTCBSE 10 - Maths
Asked by bnarayanamstr | 03 May, 2023, 10:03: AM
ANSWERED BY EXPERTCBSE 10 - Maths
Asked by savitamahajan202 | 30 Dec, 2022, 12:51: PM
ANSWERED BY EXPERT
CBSE 10 - Maths
Asked by bhu.joshi54 | 04 Jul, 2022, 08:18: PM
ANSWERED BY EXPERTCBSE 10 - Maths
Asked by bhu.joshi54 | 16 Jun, 2022, 07:56: PM
ANSWERED BY EXPERTCBSE 10 - Maths
Asked by dishapalnati82 | 24 Nov, 2021, 09:30: AM
ANSWERED BY EXPERTCBSE 10 - Maths
Asked by psinghaladv | 05 Oct, 2021, 07:49: PM
ANSWERED BY EXPERT
