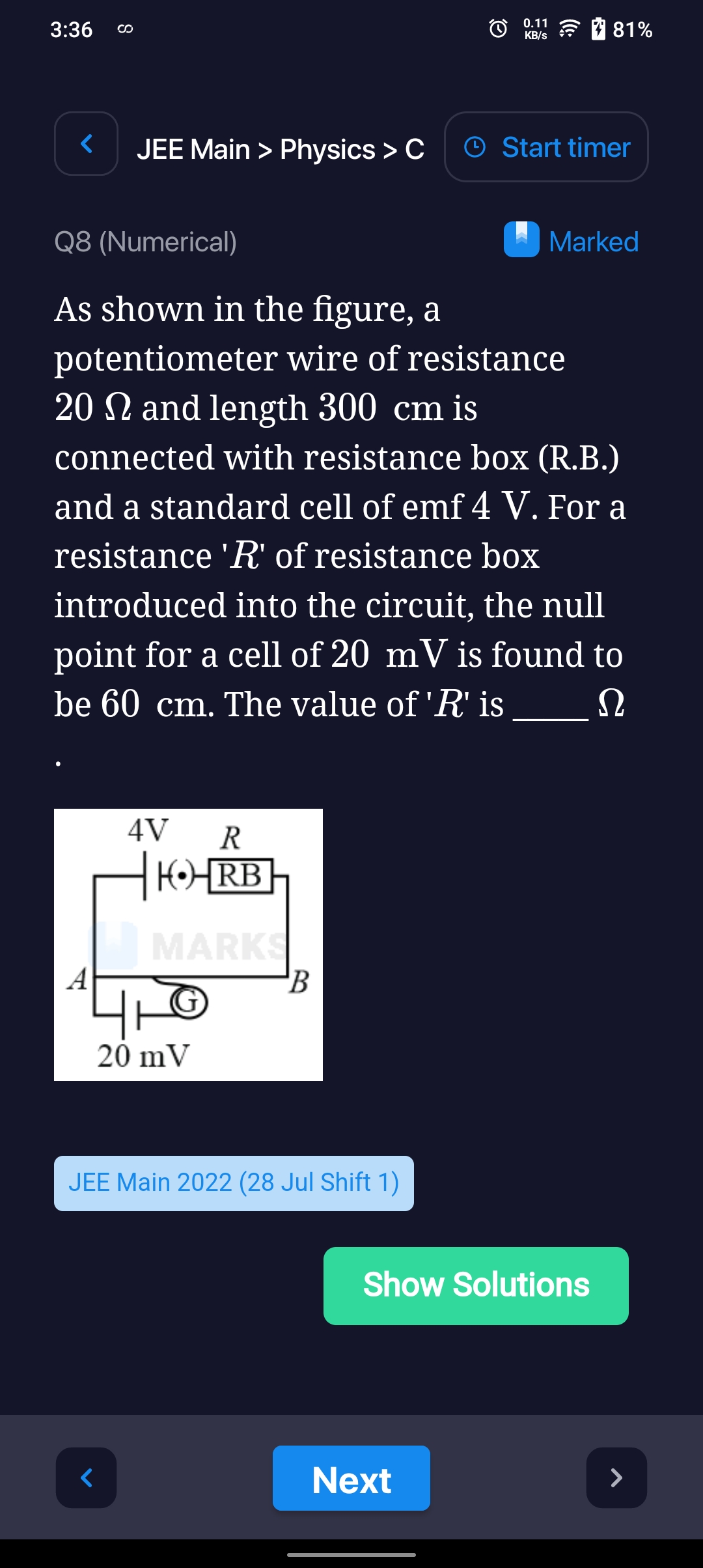
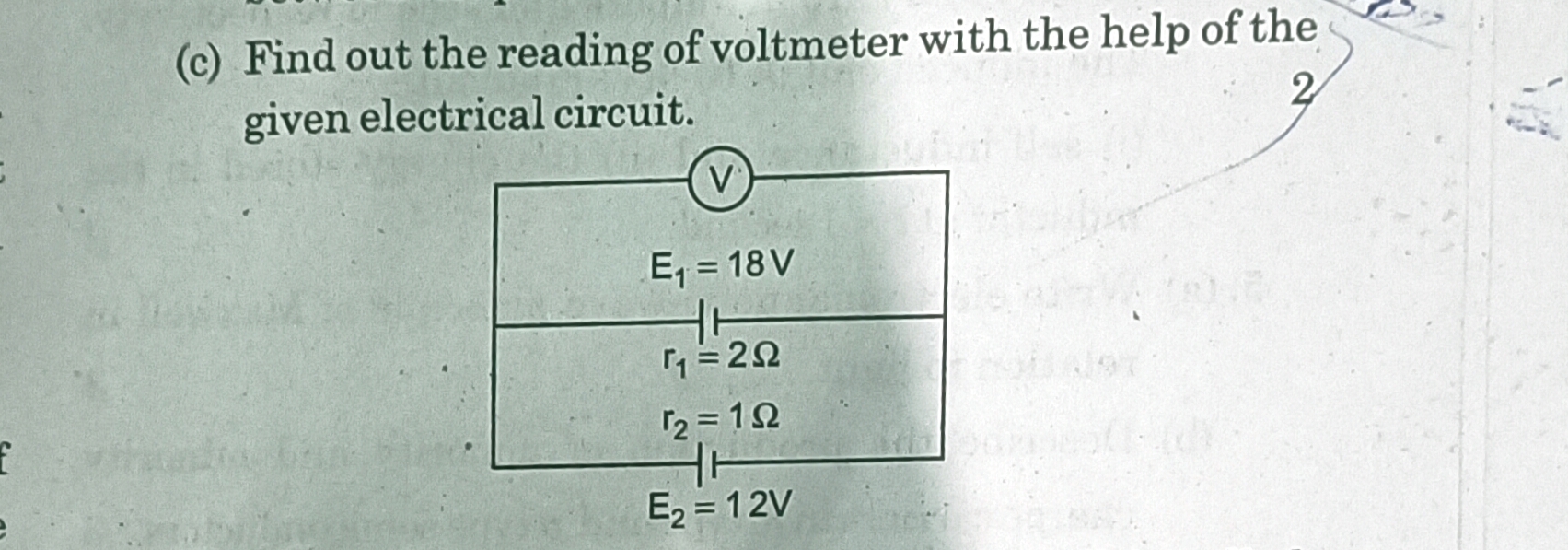
CBSE Class 12-science Answered
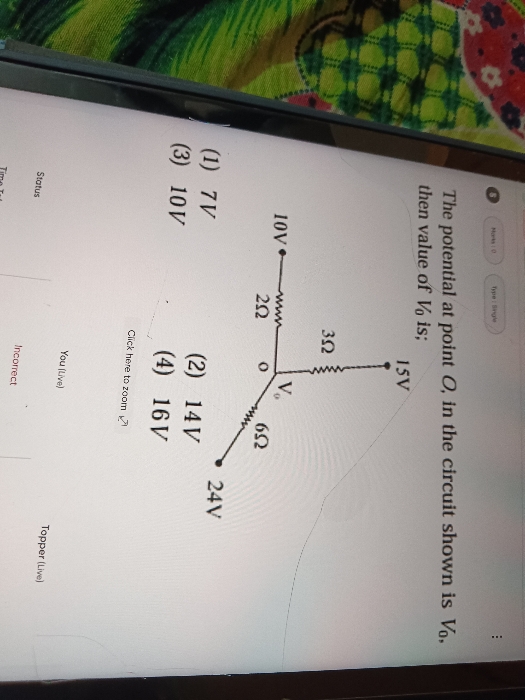
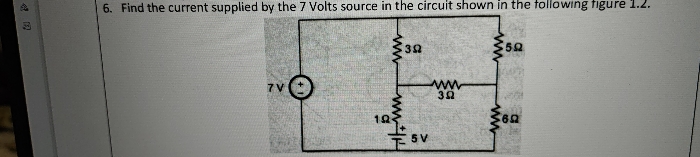
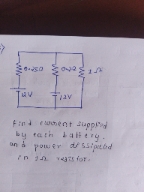
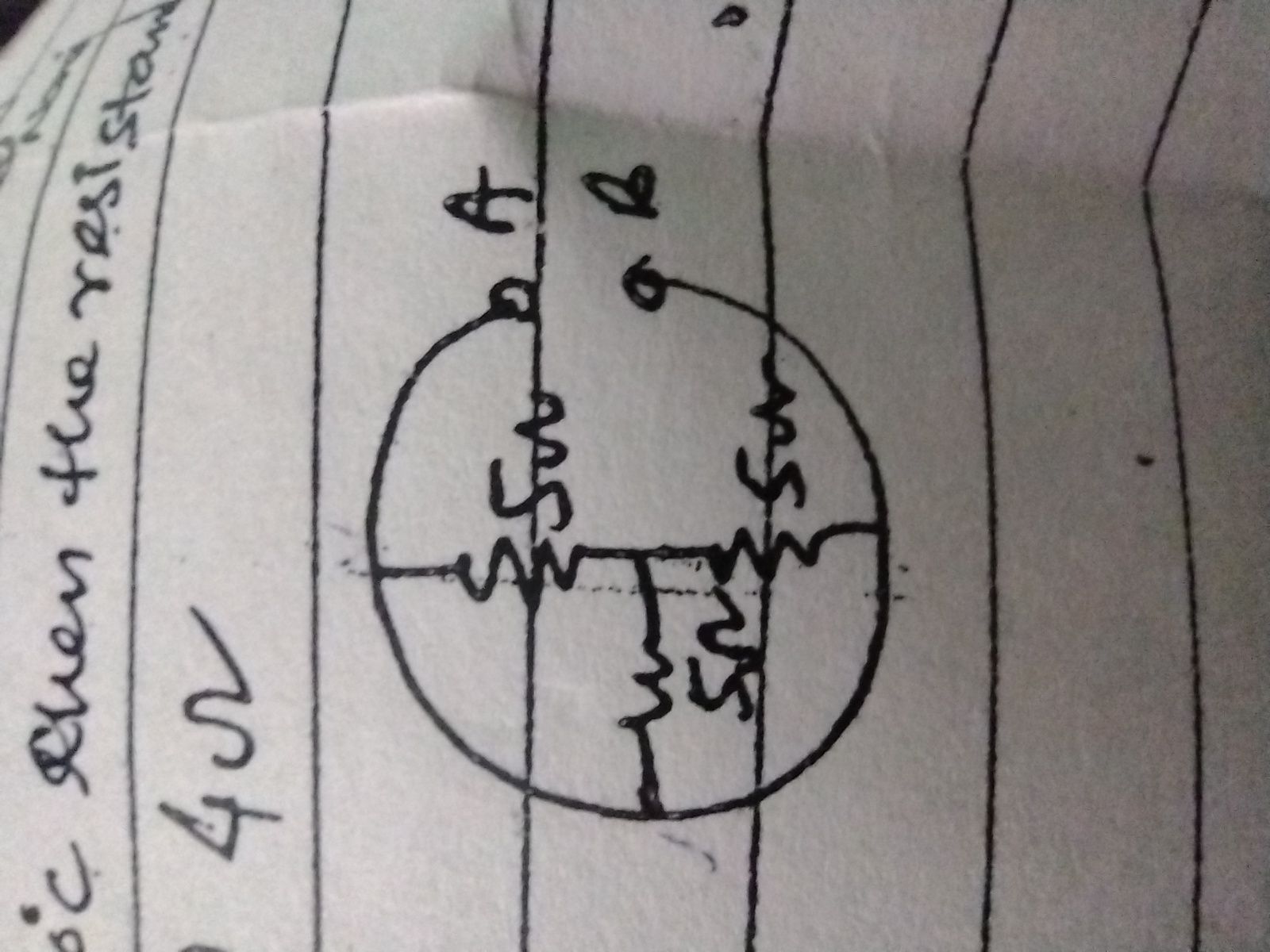
application of kirchhoff rule
Asked by hussain shaikh | 18 May, 2011, 05:24: PM
Expert Answer
APPLYING KIRCHHOFFS RULES:
1. Label + and ? for each battery. The long side of a battery
symbol is +.
2. Label the current in each branch with a symbol and an arrow.
The direction of the arrow can be chosen arbitrarily. If the current
is actually in the opposite direction, it will come out with a minus
sign in the solution.
3. Apply Kirchhoffs junction rule at each junction and the loop
rule for each loop. You will need as many independent equations
as there are unknowns. You may write down more equations than
this, but you will find that some of the equations will be redundant
(that is, not independent in the sense of providing new
information). You may use V = i× R for each resistor, which
sometimes will reduce the number of unknowns.
4. In applying the loop rule, follow each loop in one direction only
(either clockwise or counterclockwise). Pay careful attention to
signs:
a) For a resistor, the sign of the potential difference is negative
if your chosen loop direction is the same as the chosen current
direction through that resistor; the sign is positive if you are
moving opposite to the chosen current direction.
(either clockwise or counterclockwise). Pay careful attention to
signs:
a) For a resistor, the sign of the potential difference is negative
if your chosen loop direction is the same as the chosen current
direction through that resistor; the sign is positive if you are
moving opposite to the chosen current direction.
b) For a battery, the sign of the potential difference is positive if
your loop direction moves from the negative terminal toward
the positive; the sign will be negative if you are moving from
the positive terminal toward the negative terminal.
your loop direction moves from the negative terminal toward
the positive; the sign will be negative if you are moving from
the positive terminal toward the negative terminal.
5. Solve the equations algebraically for the unknowns. Be careful
in manipulating equations not to err with signs. At the end, check
your answer by plugging them into the original equations, or even
by using additional equations not used previously (either loop or
junction rule equation).
Answered by | 18 May, 2011, 12:13: PM
Concept Videos
-
Electric Current
This video explains the concept of electric current. -
Drift of Electrons - Part 1
This video explain the drift speed and its dependence on the externally app... -
Compare the resistivity of conductors, insulators and s ...
Compare the resistivity of conductors, insulators and semiconductors. Study... -
About emf, potential difference & internal resistan ...
Define emf, potential difference & internal resistance. Identify factors on... -
Combination of Cells - Part 1
This video describes about the working of cells in series combination.
CBSE 12-science - Physics
Asked by mailtoanjalip2005 | 16 Mar, 2024, 08:22: PM
ANSWERED BY EXPERTCBSE 12-science - Physics
Asked by patelnamra608 | 26 Jan, 2024, 11:01: AM
ANSWERED BY EXPERTCBSE 12-science - Physics
Asked by smitdholakiya28 | 17 Dec, 2023, 09:37: AM
ANSWERED BY EXPERTCBSE 12-science - Physics
Asked by shivashikhar69 | 09 Dec, 2023, 02:27: PM
ANSWERED BY EXPERTCBSE 12-science - Physics
Asked by nikhilsai2616 | 19 Nov, 2023, 01:05: AM
ANSWERED BY EXPERTCBSE 12-science - Physics
Asked by snehashiragannavar773 | 21 Oct, 2023, 02:38: PM
ANSWERED BY EXPERTCBSE 12-science - Physics
Asked by dr.strange45678 | 02 Aug, 2023, 12:31: PM
ANSWERED BY EXPERTCBSE 12-science - Physics
Asked by komalbrar0987 | 17 May, 2023, 06:15: PM
ANSWERED BY EXPERT
CBSE 12-science - Physics
Asked by amanpanday6384 | 22 Dec, 2022, 12:42: PM
ANSWERED BY EXPERT
