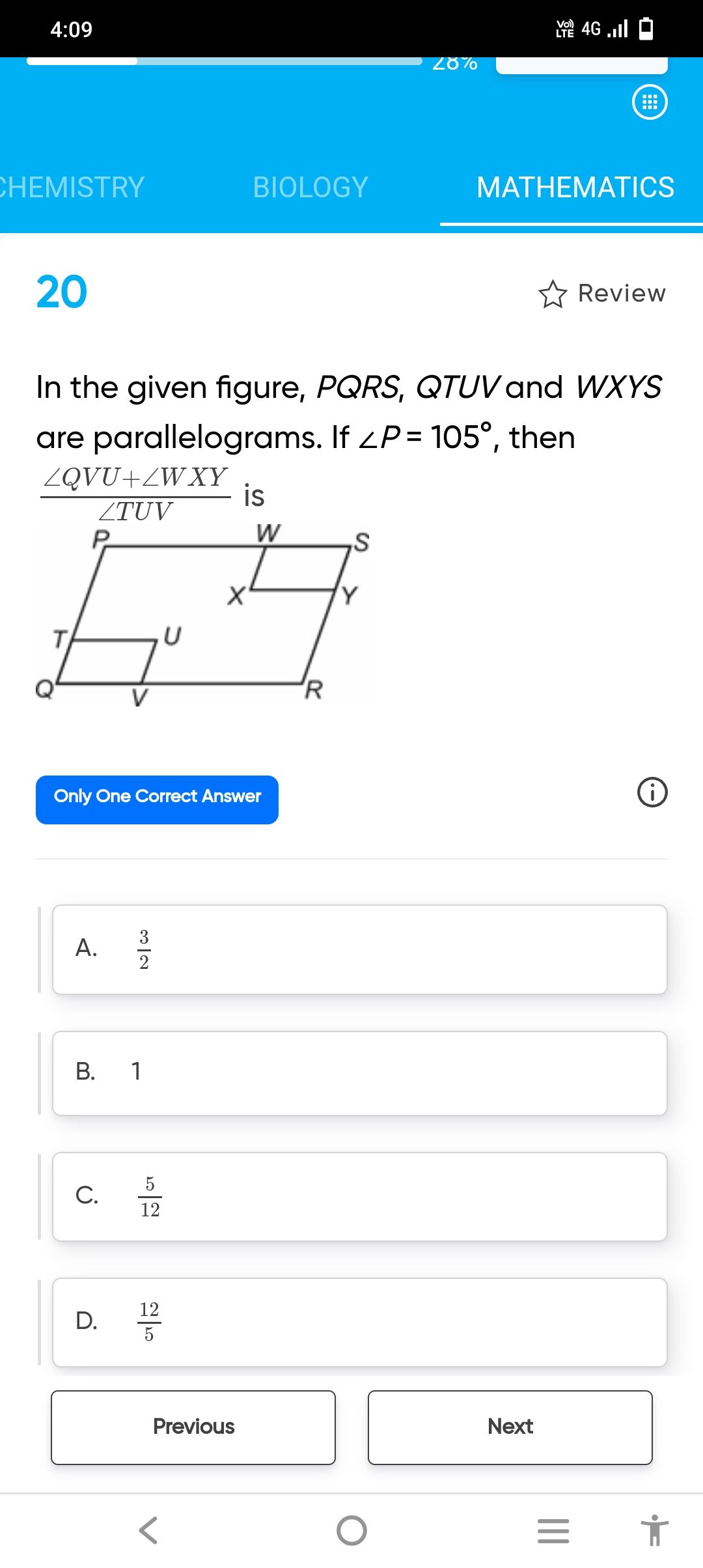
CBSE Class 9 Answered
ABCD is a quadrilateral in which P,Q,R and S are mid points of sides AB BC CD DA
show that PQRS IS A PARALELLOGRAM
Asked by Sanmit Ratnaparkhi | 19 Mar, 2014, 11:48: PM
Expert Answer
ABCD is a quadrilateral.
P, Q, R, S are the mid points of sides AB, BC, CD and DA.
Join Diagonal AC.
Consider triangle ABC.
Here, P and Q are the mid points of sides AB and BC respectively.
So, by using the mid point theorem we can say that PQ is parallel to AC.
Similarly RS can be shown parallel to AC.
So, we have PQ parallel to RS (as both are parallel to AC)
In the same way you can join diagonal BD and show that PS is parallel to QR.
So we have a quadrilateral PQRS in which both pairs of opposite sides are parallel. SO, it becomes a parallelogram.
Answered by | 20 Mar, 2014, 10:12: AM
Application Videos
-
Relation between Parallelogram and Triangle ...
This video explains the relation between the parallelogram and the triangle... -
Properties of a Parallelogram
This video contains a question which is solved using the property of a para... -
Use of Mid-point theorem
The video explains case study based type of question which is based on t...
-
Properties of Parallelogram
The video explains case study based type of question which is based on t...
-
Properties of quadrilateral
The video explains case study based type of question which is based on t...
Concept Videos
-
About relation between opposite angles in a parallelogr ...
Relation between opposite angles in a parallelogram. Explore the conditions... -
Relation between the diagonals of a parallelogram ...
Establish the relation between the diagonals of the parallelogram. Conditio... -
Mid Point Theorem
This video explains the Mid - Point theorem and its converse for a triangle... -
Types and Angle Sum Property
. Definition of Quadrilateral & special quadrilaterals: rectangle, square,... -
Parallelograms: Basic Properties
Parallelograms:Basic Properties
CBSE 9 - Maths
Asked by chandininayak86 | 15 Dec, 2023, 07:36: AM
ANSWERED BY EXPERTCBSE 9 - Maths
Asked by sagrawal0081 | 31 Aug, 2023, 07:24: PM
ANSWERED BY EXPERTCBSE 9 - Maths
Asked by jahnavinehra | 03 Jul, 2022, 11:19: AM
ANSWERED BY EXPERTCBSE 9 - Maths
Asked by seemarana8535 | 11 May, 2022, 04:17: PM
ANSWERED BY EXPERTCBSE 9 - Maths
Asked by abhiroy1462 | 16 Apr, 2022, 02:25: PM
ANSWERED BY EXPERTCBSE 9 - Maths
Asked by abdullahzedane2008 | 28 Mar, 2022, 12:11: PM
ANSWERED BY EXPERTCBSE 9 - Maths
Asked by sanskritiraghudev | 19 Feb, 2022, 09:38: AM
ANSWERED BY EXPERTCBSE 9 - Maths
Asked by varundhawan | 31 Jan, 2022, 10:41: PM
ANSWERED BY EXPERTCBSE 9 - Maths
Asked by aziznoorani17 | 04 Jan, 2022, 11:32: AM
ANSWERED BY EXPERTCBSE 9 - Maths
Asked by vineetyadav77050 | 25 Jul, 2021, 05:45: PM
ANSWERED BY EXPERT
