Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

JEE Class main Answered

 A particle is projected under gravity with a velocity u in a direction making an  angle a with the horizon. Show that the amount of deviation D in direction of  motion of the particle is given by : tan ( cos ) / ( sin )
Asked by dhandas060104 | 24 Oct, 2020, 21:29: PM
answered-by-expert Expert Answer
Let uo be initial projection velocity at an angle α with horizontal
 
At any instant t, vertical component of velocity = ( uo sinα )- gt
 
At any instant t, horizontal component of velocity = uo cosα
 
Angle β made by direction of motion with horizontal is determined as
 
tanβ = [ ( uo sinα )- gt ] / ( uo cosα )  = tanα - [ (gt) / (uo cosα ) ] ...................(1)
 
From above equation-(1) , we get , tanα - tanβ = [ (gt) / (uo cosα ) ]  ....................(2)
 
also we get from eqn.(1) , tanα tanβ = tan2α - [ ( g t sinα ) / ( uo cos2α ) ]  .................(3)
 
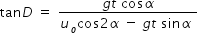
Angle of deviation D in direction of motion,  D = ( α - β)
 
tanD = tan(α - β ) = [ tanα - tanβ ] / [ 1 - (tanα tanβ) ] .............. (4)
 
Using eqn.(2) and eqn.(3) , eqn.(4) is rewritten after simplification as
 
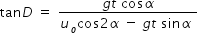
begin mathsize 14px style tan D space equals space fraction numerator g t space cos alpha over denominator u subscript o cos 2 alpha space minus space g t space sin alpha space end fraction end style
 
 
Answered by Thiyagarajan K | 25 Oct, 2020, 10:07: AM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
JEE main - Physics
a marble block of mass 2 kg lying on ice when a given velocity of 6 ms–1 is stopped by friction in 10 seconds then co efficient of friction is
Asked by sumalathamadarapu9 | 23 Oct, 2024, 22:06: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
show that the frequency of oscillations remain unchanged whether a mass attached to a spring is made to oscillate in the vertical or in the horizontal direction
Asked by py309649 | 13 Oct, 2024, 13:39: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
Square of the time period of revolution of a planet is proportional to the cube of the mean distance between sun and planet. If mean distance is increased by 2% then percentage change in its time period is
Asked by coolskrish | 13 Oct, 2024, 12:50: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two particles of mass m and 4m are initially actress the sad moving towards each other due to mutual gravitational force at an instantly of m is vî then at this instant the velocity of mass 4m is
Asked by midnightmoon3355 | 09 Oct, 2024, 09:09: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the second law of thermodynamics
Asked by rambabunaidu4455 | 03 Oct, 2024, 16:03: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the relation between drift velocity and mobility
Asked by ratchanavalli07 | 17 Sep, 2024, 07:46: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
A ball of mass m approaches a moving wall of infinite mass with speed v along the normal to the wall. The speed of the ball is u towards the ball. The speed of the ball after elastic collision with the wall is
Asked by yayashvadutta45 | 15 Sep, 2024, 19:47: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
a current of 2a is flowing through a conductor of length 22m bent in arc radius 5cm
Asked by adithireddy999 | 03 Sep, 2024, 09:35: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two vectors vector a and vector B lie in one plane vector c lie in the different plane then vector a + vector b + vector c is
Asked by vaishalinirmal739 | 29 Aug, 2024, 18:07: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
What is impulse?
Asked by vradhysyam | 26 Aug, 2024, 17:17: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt