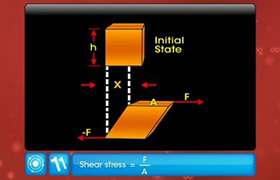
CBSE Class 11-science Answered

When a wire is put under a tensile stress, work is done against the inter-atomic forces. This work is stored in the wire in the form of elastic potential energy. When a wire of original length L and area of cross-section A is subjected to a deforming force F along the length of the wire, let the length of the wire is elongated by l. Then we have F=YA (l/L). Here Y is the Young’s modulus of the material of the wire. Now for a further elongation of infinitesimal small length dl, then the work done dW is F dl or Y A l d l /L.
Therefore the amount of work done (W ) in increasing the length of the wire from l to L + l, that is from l = 0 to l = l is

 

 

Careful observations with the Young’s modulus experiment show that there is also a slight reduction in the cross-section (or in the diameter) of the wire. The strain perpendicular to the applied force is called lateral strain. Simon Poisson pointed out that within the elastic limit; lateral strain is directly proportional to the longitudinal strain. The ratio of the lateral strain to the longitudinal strain in a stretched wire is called Poisson’s ratio. If the original diameter of the wire is d and the contraction of the diameter under stress is ?d, the lateral strain is ?d/d. If the original length of the wire is L and the elongation under stress is ?L, the longitudinal strain is ?L/L. Poisson’s ratio is then (?d/d)/(?L/L) or (?d/?L) (L/d). Poisson’s ratio is a ratio of two strains; it is a pure number and has no dimensions or units. Its value depends only on the nature of material. For steels the value is between 0.28 and 0.30, and for aluminum alloys it is about 0.33.